Collisions Elastic vs Inelastic But first an example

Collisions Elastic vs. Inelastic

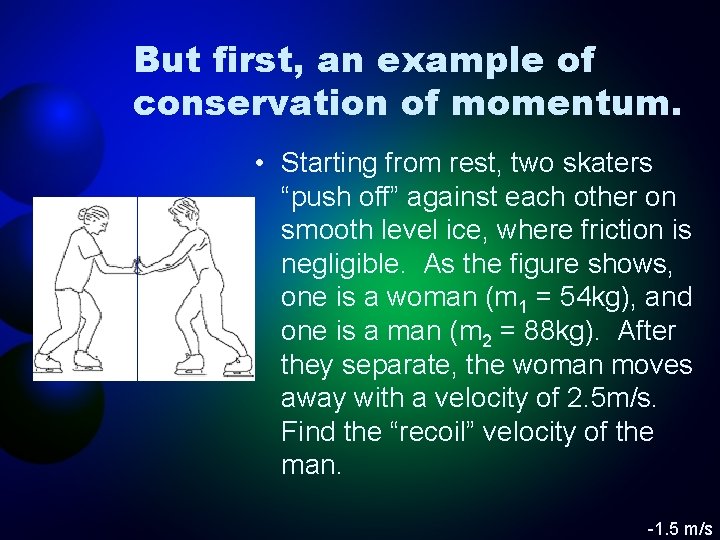
But first, an example of conservation of momentum. • Starting from rest, two skaters “push off” against each other on smooth level ice, where friction is negligible. As the figure shows, one is a woman (m 1 = 54 kg), and one is a man (m 2 = 88 kg). After they separate, the woman moves away with a velocity of 2. 5 m/s. Find the “recoil” velocity of the man. -1. 5 m/s

The law of conservation of momentum is seen in collisions. Whenever objects collide in the absence of external forces, the total or net momentum never changes. Net momentum before collision = Net momentum after collision

Elastic collisions • When a moving billiard ball makes a headon collision with another billiard ball at rest, the moving ball comes to rest, and the struck ball moves with the initial velocity of the colliding ball. • Momentum is simply transferred from one ball to the other.

Elastic collisions • When the colliding objects rebound without lasting deformation or the generation of heat, the collision is said to be an elastic collision. p 1 i + p 2 i = p 1 f + p 2 f m 1 v 1 i + m 2 v 2 i = m 1 v 1 f + m 2 v 2 f

Inelastic collisions • Momentum conservation holds true even when the colliding objects become distorted and generate heat during the collision. • Such collisions are called inelastic collisions. • If the masses stick together, “perfectly inelastic” m 1 v 1 i + m 2 v 2 i = (m 1 + m 2)vf

A simple inelastic collision problem… • A freight train is being assembled in a switching yard, and the figure shows two boxcars. Car 1 has a mass of 65, 000 kg and moves at a velocity of +0. 80 m/s. Car 2, with a mass of 92, 000 kg and a velocity of +1. 3 m/s, reaches car 1 and couples to it. Neglecting friction, find the common velocity of the cars after they become coupled. 1. 1 m/s

Elastic collisions also conserve kinetic energy while inelastic collisions don’t. • Why? Because some kinetic energy is lost to heat and deformation.

Elastic collision in 1 -D • A ball of mass 0. 250 kg and velocity +5. 00 m/s collides head on with a ball of mass 0. 800 kg that is initially at rest. No external forces act on the balls. If the collision is elastic, what are the velocities of the balls after collision? -2. 62 m/s, +2. 38 m/s

A ballistic pendulum • A ballistic pendulum can be used to measure the speed of a projectile, such as a bullet. The ballistic pendulum consists of a block of wood mass 2. 50 kg suspended by a wire of negligible mass. A bullet mass 0. 0100 kg is fired with a speed vi 1. Just after the bullet collides with it, the block (with the bullet in it) has a speed vf and then swings to a maximum height of 0. 650 m above the initial position. Find the initial speed of the bullet, assuming that air resistance is negligible. h +896 m/s

The bottom line… • In any collision – no matter how complicated – as long as there are no net external forces, momentum is conserved!!! • Energy however may or may not be conserved.