Chapter 7 Similarity and Proportion Express a ratio
![Properties of Proportion [AKA – Different ways to say the same thing] is equivalent](https://slidetodoc.com/presentation_image_h/04c6ec308003e1c4bd3f2a232d81b3ed/image-20.jpg "Properties of Proportion [AKA – Different ways to say the same thing] is equivalent")
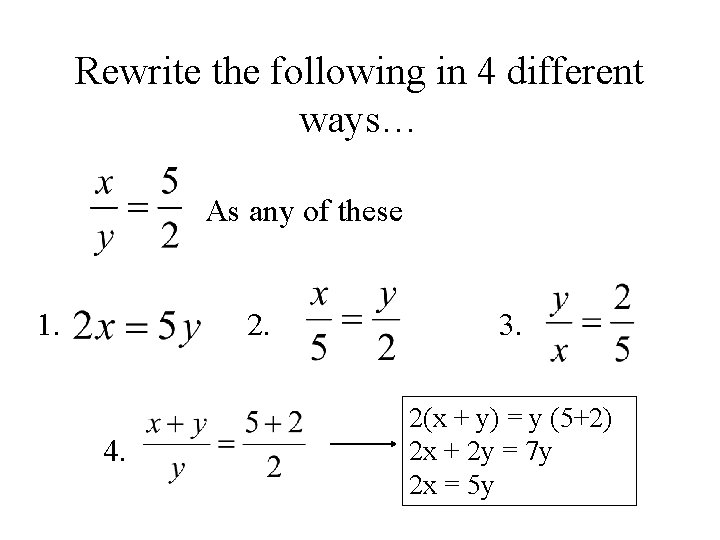
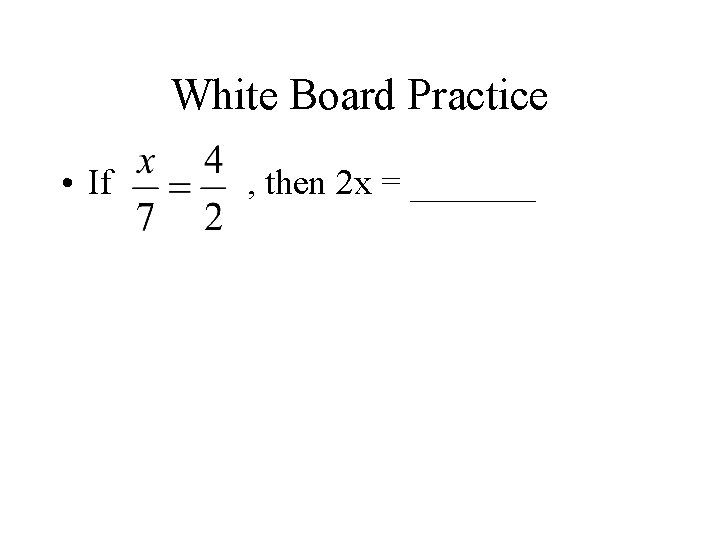
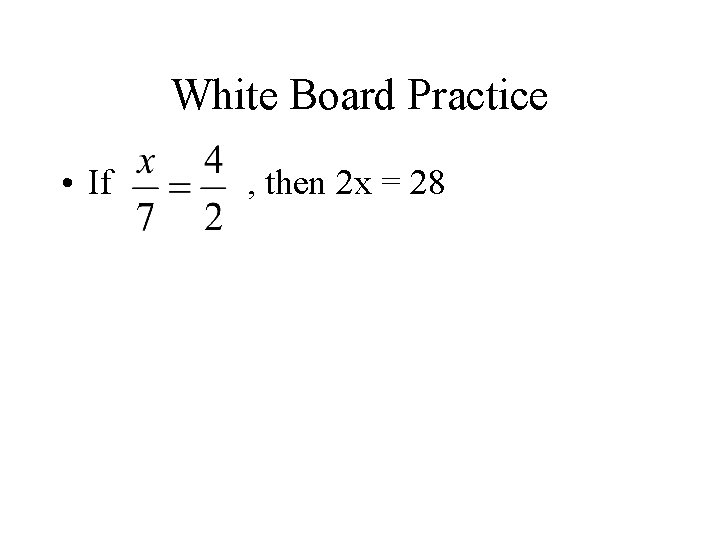
- Slides: 91
Chapter 7 Similarity and Proportion • Express a ratio in simplest form. • State and apply the properties of similar polygons. • Use theorems about similar triangles.
Warm –up • In complete sentences, explain what a ratio is. Create a real-life example of a ratio being used. • In complete sentences, explain what a proportion is.
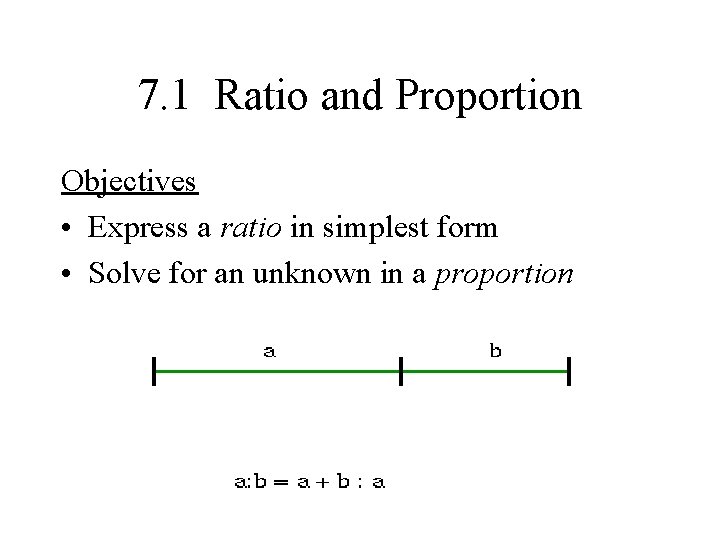
7. 1 Ratio and Proportion Objectives • Express a ratio in simplest form • Solve for an unknown in a proportion
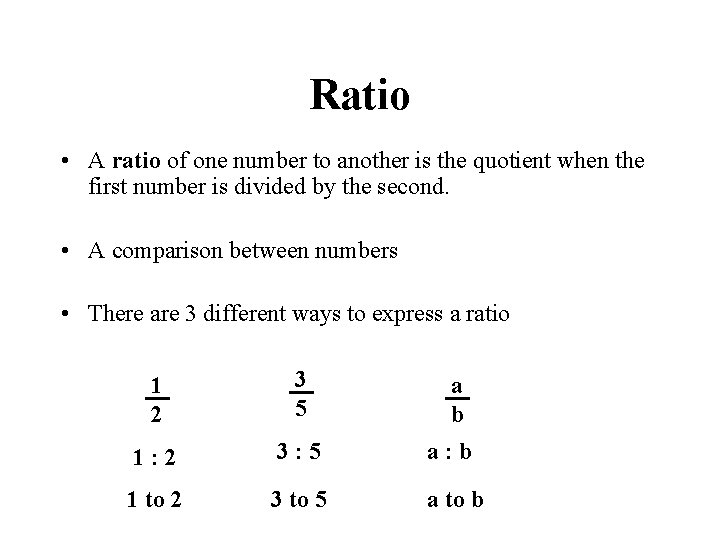
Ratio • A ratio of one number to another is the quotient when the first number is divided by the second. • A comparison between numbers • There are 3 different ways to express a ratio 1 2 3 5 1: 2 3: 5 a: b 1 to 2 3 to 5 a to b a b
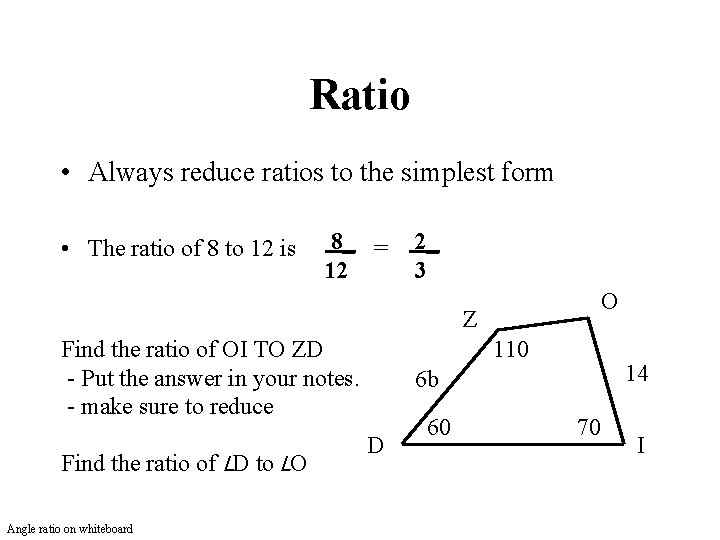
Ratio • Always reduce ratios to the simplest form • The ratio of 8 to 12 is 8_ = 12 2_ 3 O Z Find the ratio of OI TO ZD - Put the answer in your notes. - make sure to reduce Find the ratio of LD to LO Angle ratio on whiteboard 110 14 6 b D 60 70 I
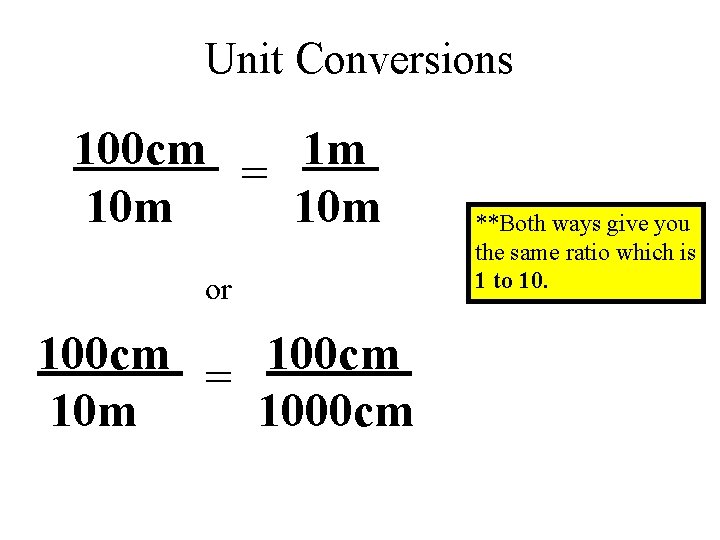
Additional Elements of Ratios • What is the ratio of 100 cm to 10 m ? 100 = 10 ? 10 1 • WHY? ? – To find the ratio of two lengths, they must always be measured in the terms of the same unit.
Unit Conversions 100 cm 1 m = 10 m or 100 cm = 10 m 1000 cm **Both ways give you the same ratio which is 1 to 10.
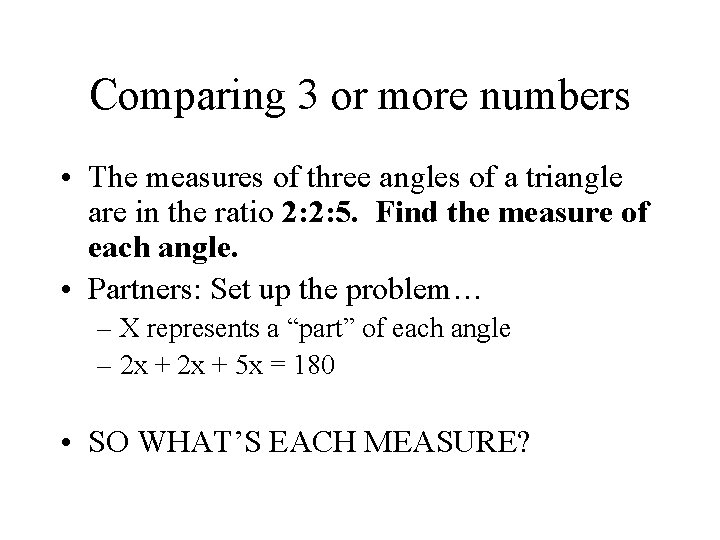
Comparing 3 or more numbers • We use the following form to represent three or more numbers that are in ratio to each other… 3: 5: 7 • Reads as. . “ 3 to 5 to 7"
Comparing 3 or more numbers • The measures of three angles of a triangle are in the ratio 2: 2: 5. Find the measure of each angle. • Partners: Set up the problem… – X represents a “part” of each angle – 2 x + 5 x = 180 • SO WHAT’S EACH MEASURE?
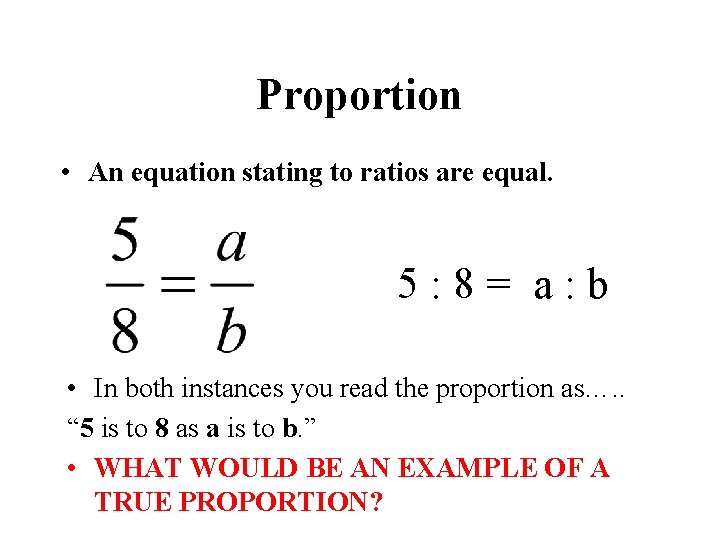
Proportion • An equation stating to ratios are equal. 5: 8= a: b • In both instances you read the proportion as…. . “ 5 is to 8 as a is to b. ” • WHAT WOULD BE AN EXAMPLE OF A TRUE PROPORTION?
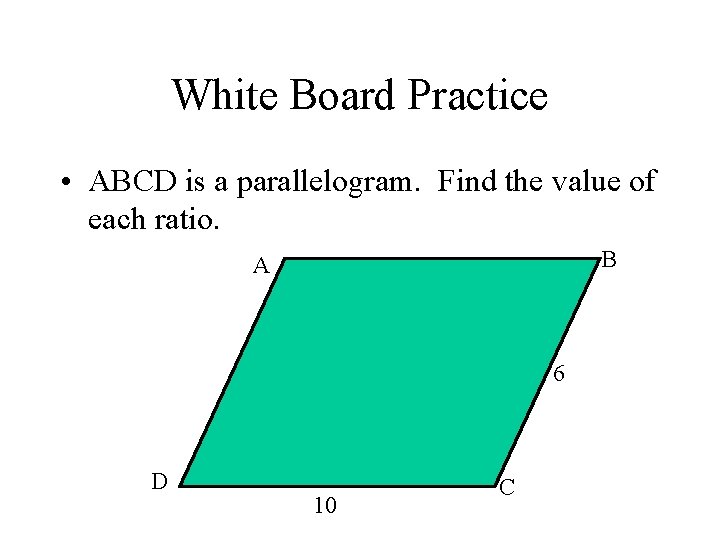
White Board Practice • ABCD is a parallelogram. Find the value of each ratio. B A 6 D 10 C
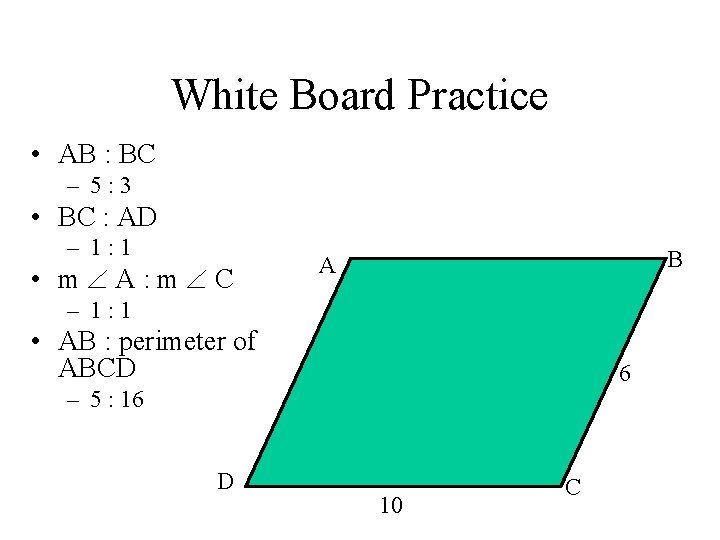
White Board Practice • AB : BC – 5: 3 • BC : AD – 1: 1 • m A: m C B A – 1: 1 • AB : perimeter of ABCD 6 – 5 : 16 D 10 C
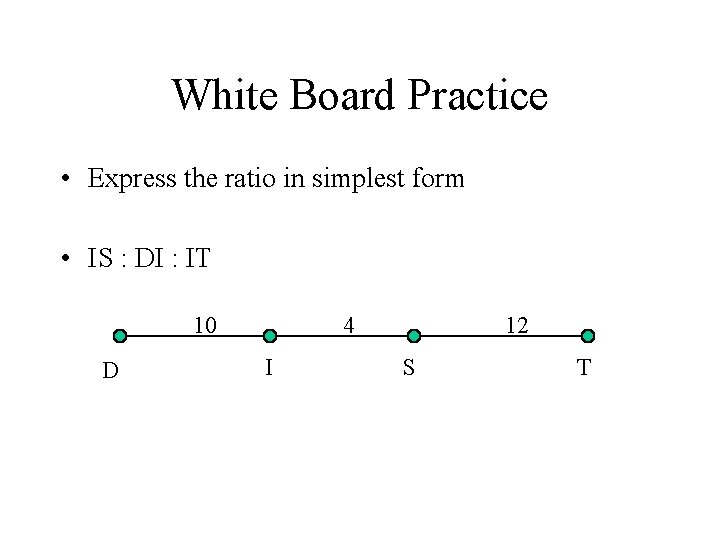
White Board Practice • Express the ratio in simplest form • IS : DI : IT 10 D 4 I 12 S T
White Board Practice • Express the ratio in simplest form • IS : DI : IT • 4 : 10 : 16 • 2 : 5 : 8 -----To reduce, find GCF 10 D 4 I 12 S T
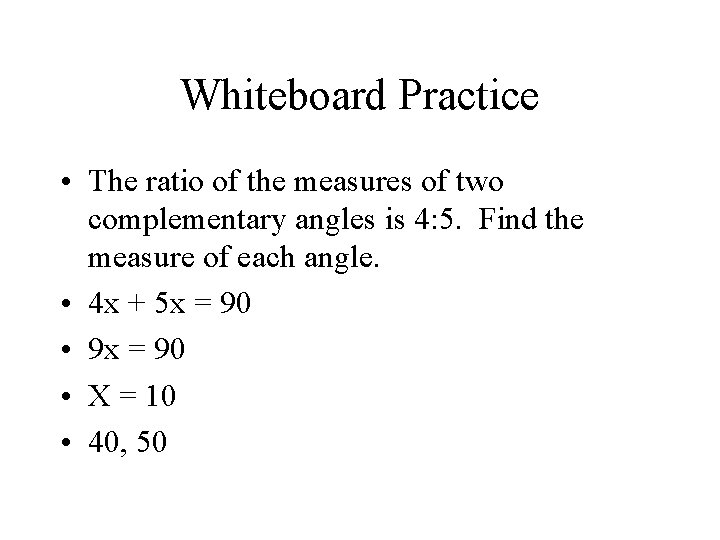
Whiteboard Practice • The ratio of the measures of two complementary angles is 4: 5. Find the measure of each angle. • 4 x + 5 x = 90 • 9 x = 90 • X = 10 • 40, 50
7. 2 Properties of Proportions Objectives • Express a given proportion in an equivalent form.
Warm - up 1. Come up with an example of a true proportion 2. How do you solve for a proportion that has a missing variable?
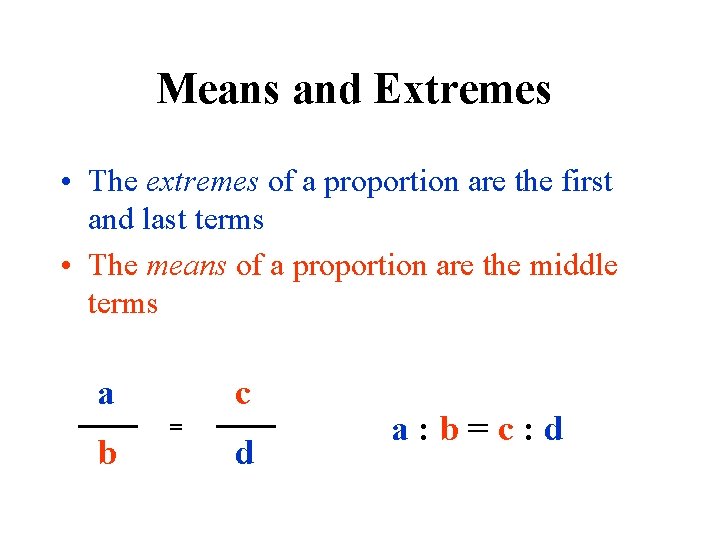
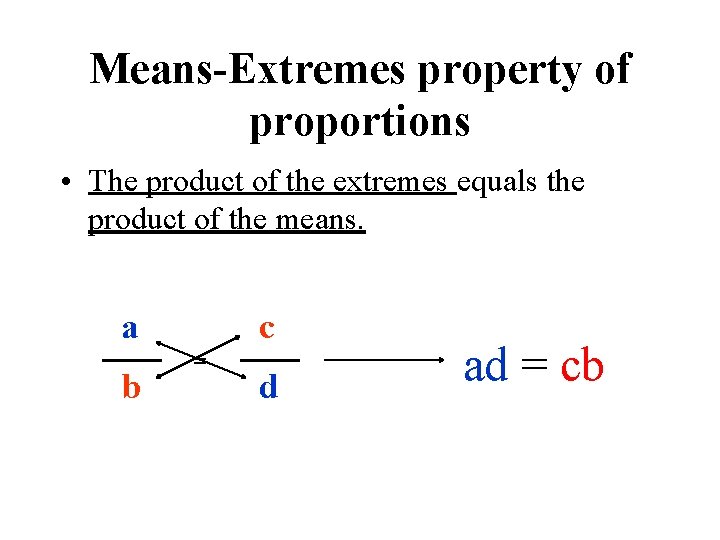
Means and Extremes • The extremes of a proportion are the first and last terms • The means of a proportion are the middle terms a b c = d a: b=c: d
Means-Extremes property of proportions • The product of the extremes equals the product of the means. a b c = d ad = cb
Properties of Proportion [AKA – Different ways to say the same thing] is equivalent to a. b. d. c. Bottom Line: When I cross multiply any of these, I will always end up back at ad=bc.
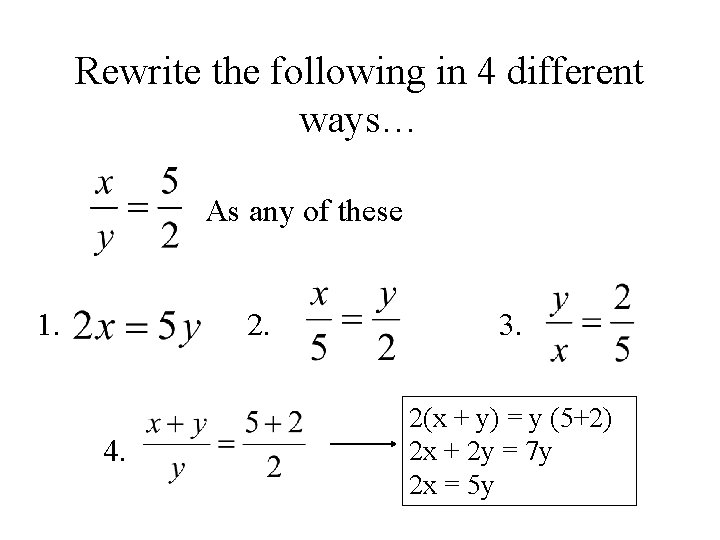
Rewrite the following in 4 different ways… As any of these 1. 2. 4. 3. 2(x + y) = y (5+2) 2 x + 2 y = 7 y 2 x = 5 y
Another Property call it the “addition property” Show whiteboard example
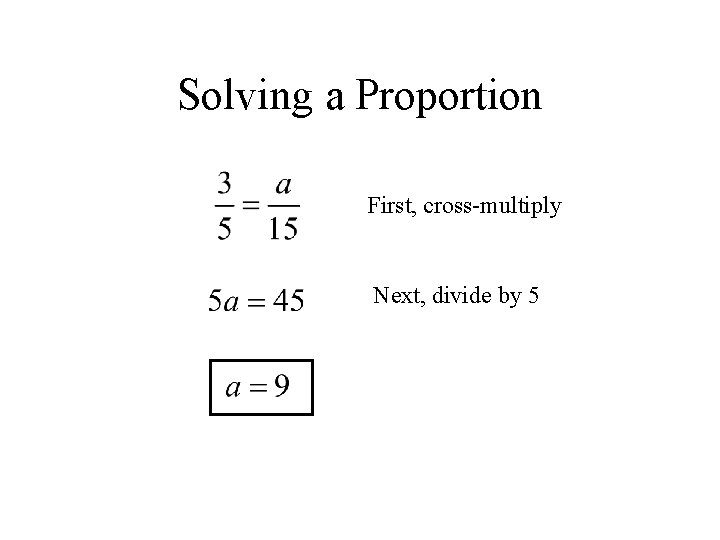
Solving a Proportion First, cross-multiply Next, divide by 5
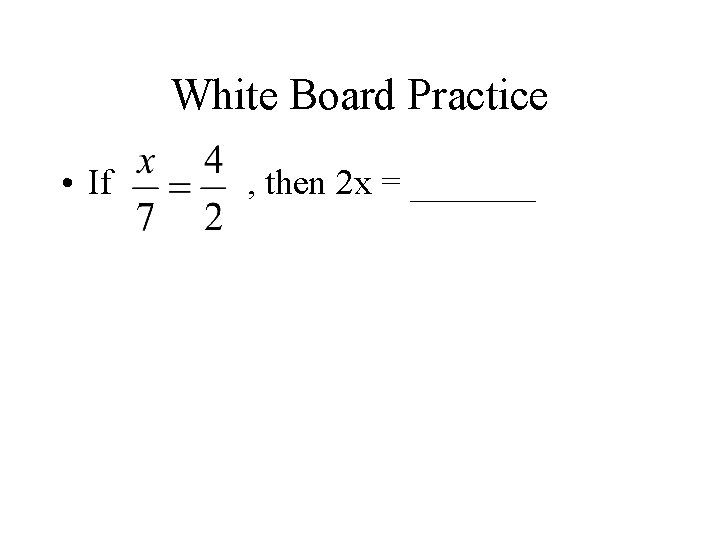
White Board Practice • If , then 2 x = _______
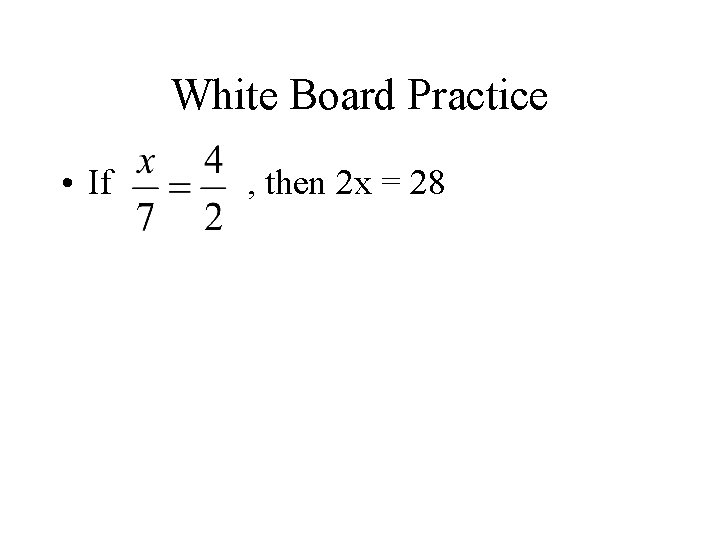
White Board Practice • If , then 2 x = 28
White Board Practice • If 2 x = 3 y, then
White Board Practice • If 2 x = 3 y, then
White Board Practice • If , then
White Board Practice • If , then
White Board Practice • If , then
White Board Practice • If , then
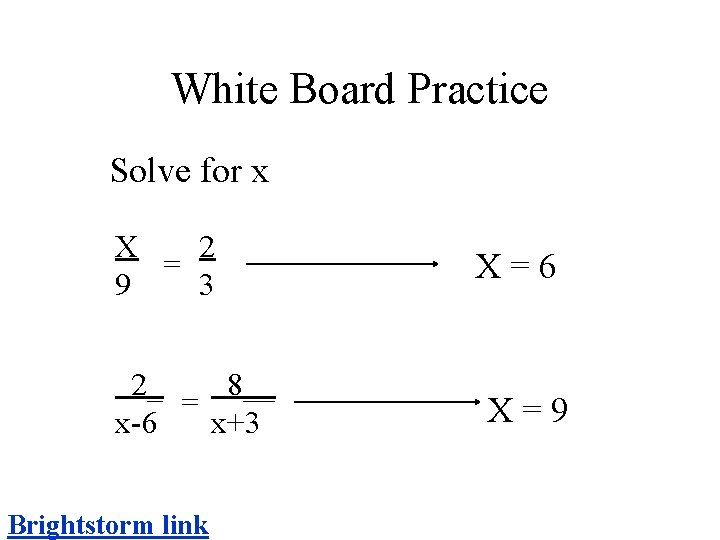
White Board Practice Solve for x X 2 = 9 3 2_ 8__ = x-6 x+3 Brightstorm link X=6 X=9
Whiteboard practice • Page 246 – #2
WARM UP • In order for 2 polys to be congruent, 2 rules must be satisfied… 1. All ________________ 2. All________________ • In a complete sentence, what do you think the difference is between 2 polys that are congruent and 2 polys that are similar?
7. 3 Similar Polygons Objectives • State and apply the properties of similar polygons.
Similarity • Coaching Football – When I need to show my players the diagram of a play, I am not going to use a piece of paper that is 50 yards wide and 100 yards long… – So what do I do? ? ? – Draw the same shape of the field but with a length and width that is drawn to a smaller scale.
Similar Polygons • Same shape • Not the same size Why? Because they would be congruent!!
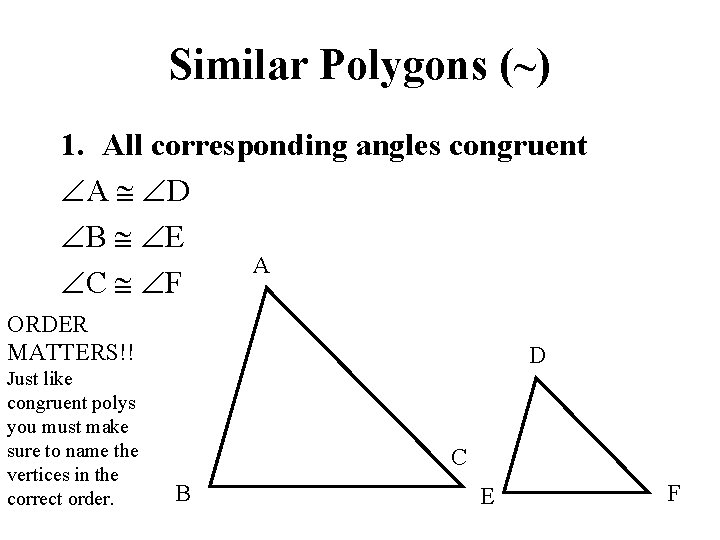
Similar Polygons (~) 1. All corresponding angles congruent A D B E A C F ORDER MATTERS!! Just like congruent polys you must make sure to name the vertices in the correct order. D C B E F
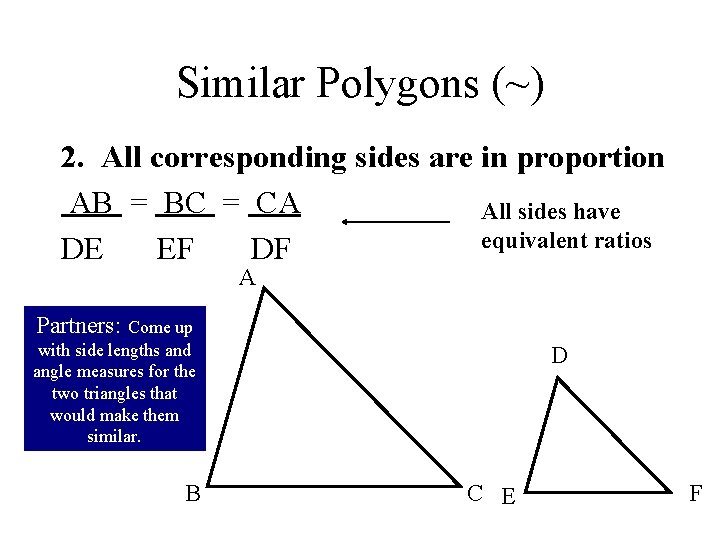
Similar Polygons (~) 2. All corresponding sides are in proportion AB = BC = CA All sides have equivalent ratios DE EF DF A Partners: Come up with side lengths and angle measures for the two triangles that would make them similar. B D C E F
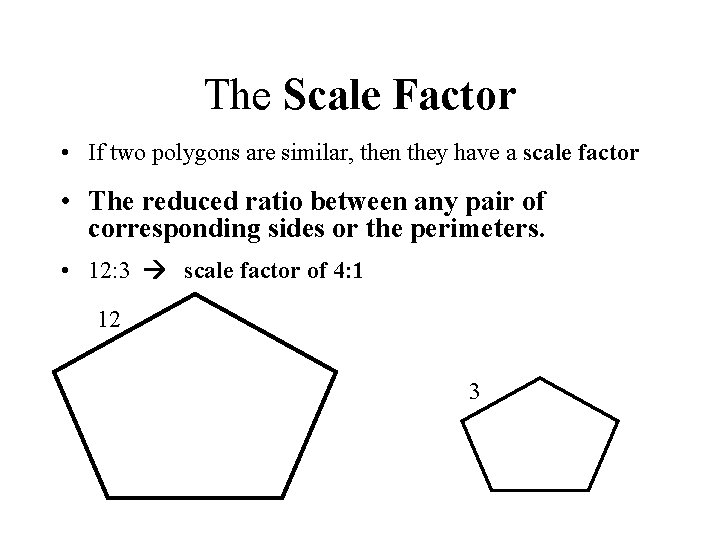
The Scale Factor • If two polygons are similar, then they have a scale factor • The reduced ratio between any pair of corresponding sides or the perimeters. • 12: 3 scale factor of 4: 1 12 3
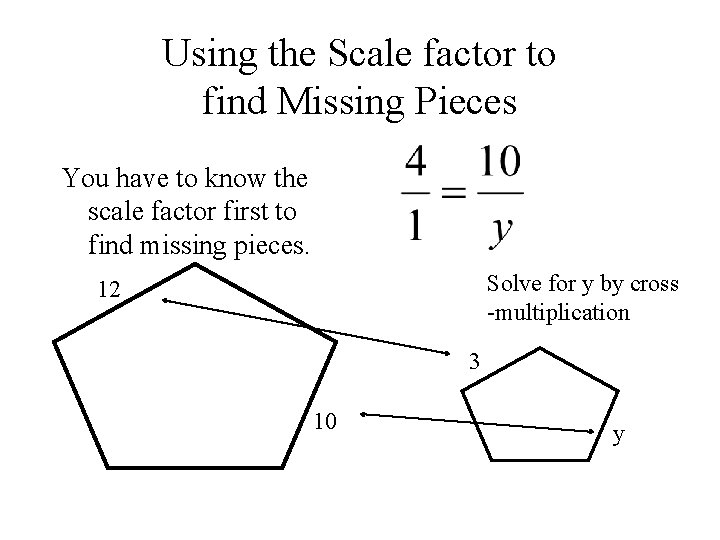
Using the Scale factor to find Missing Pieces You have to know the scale factor first to find missing pieces. Solve for y by cross -multiplication 12 3 10 y
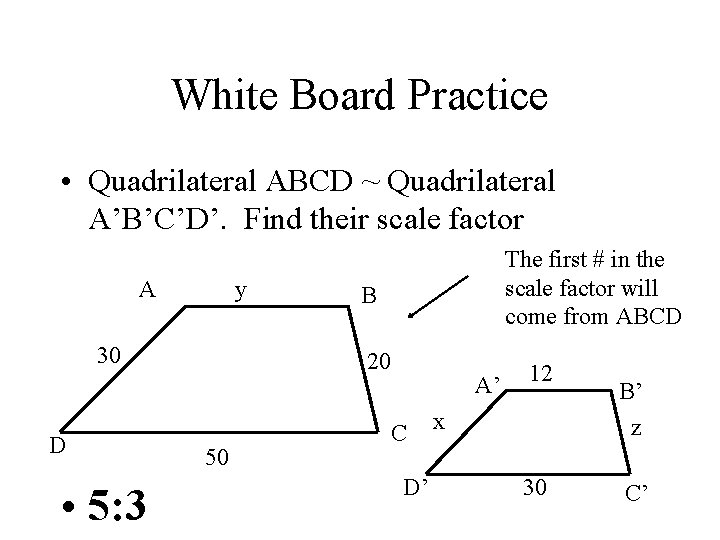
White Board Practice • Quadrilateral ABCD ~ Quadrilateral A’B’C’D’. Find their scale factor A y 30 D • 5: 3 The first # in the scale factor will come from ABCD B 20 50 A’ C D’ 12 x 30 B’ z C’
White Board Practice • Quadrilateral ABCD ~ Quadrilateral A’B’C’D’. Find the values of x, y, and z A y 30 D • x = 18 • y = 20 • z = 12 B 20 50 A’ C D’ 12 x 30 B’ z C’
White Board Practice • Quadrilateral ABCD ~ Quadrilateral A’B’C’D’. Find the ratio of the perimeters A y 30 D • 5: 3 B 20 50 A’ C D’ 12 x 30 B’ z C’
Additional Problems • Example • Page 250 – Classroom ex. – #10
Quiz Review Section 7. 1 • • Putting ratios into simplest form Find the measure of each angle based on a ratio – i. e. Pg. 244 #24 – 29 Section 7. 2 • Properties of proportions ( purple box pg. 245) – i. e. how can the proportion be changed around and still be equal to the original # 1 -8) • Find the value of X ( Cross multiply and solve) – i. e. Pg. 247 #9 – 20 Section 7. 3 • • Understand the definition of similar polygons (~) Finding the scale factor of similar polys – Compare the lengths of corresponding sides (reduce) • Use the scale factor to find unknown lengths – i. e. Pg. 251 #15 - 26 (i. e. pg. 247
Warm – Up • Using the book or notes… • Write down the definitions for the following – Ratio – Proportion – Scale factor – Similar Polygons
7. 4 A Postulate for Similar Triangles Objectives • Learn to prove triangles are similar.
What we have learned… • Two polygons are similar by showing that they satisfy the definition of similar polygons (~) – 3 pair of corresponding angles are congruent – 3 pair of corresponding sides are in proportion Why does this whole 3 pair thing sound so familiar?
Index Card Experiment Supplies: Index card, Scissors, Ruler 1. Cut out a triangle using a 3 x 5 index card 2. Label the vertices A, B, C 3. Take side BC of your triangle
Index Card Experiment 4. Draw a line that is twice the length of AC and label the endpoints X and Z 5. At X line up angle A of your triangle and trace it on the paper. Then do the same thing for Z with angle C. 6. Use a straight edge to continue the sides of the angles until they intersect
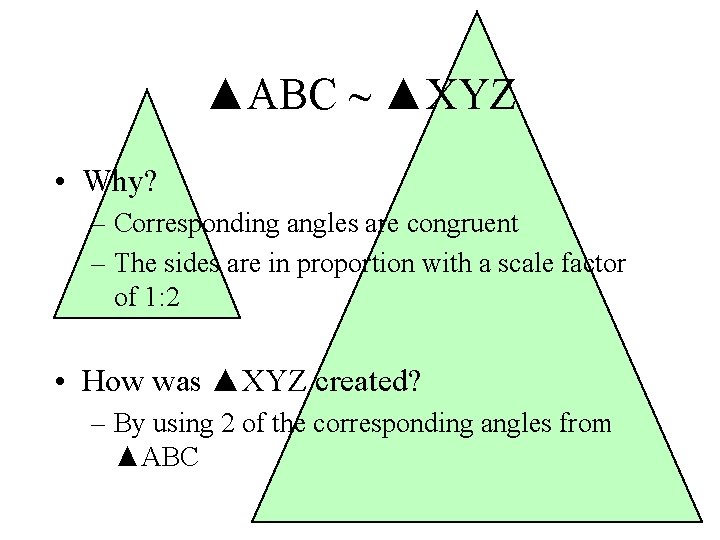
▲ABC ~ ▲XYZ • Why? – Corresponding angles are congruent – The sides are in proportion with a scale factor of 1: 2 • How was ▲XYZ created? – By using 2 of the corresponding angles from ▲ABC
AA Similarity Postulate (AA~ Post) If two angles of one triangle are congruent to two angles of another triangle, then the triangles are similar. A D B C F E
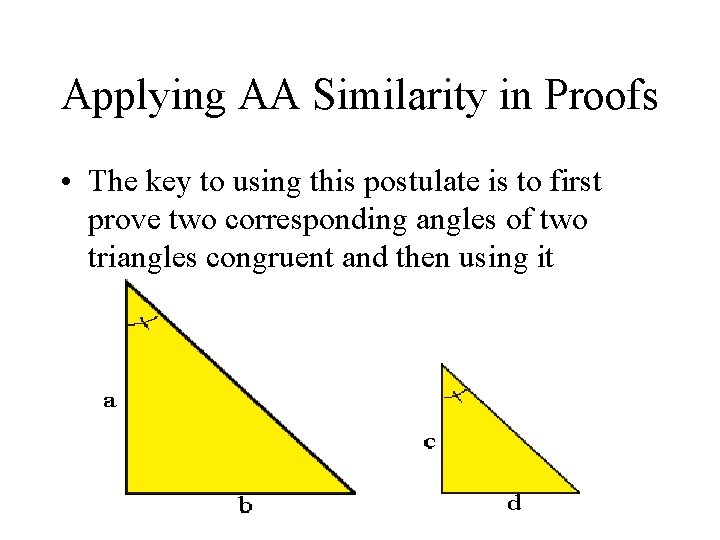
Applying AA Similarity in Proofs • The key to using this postulate is to first prove two corresponding angles of two triangles congruent and then using it
Remote Time • T – Similar Triangles • F – Not Similar
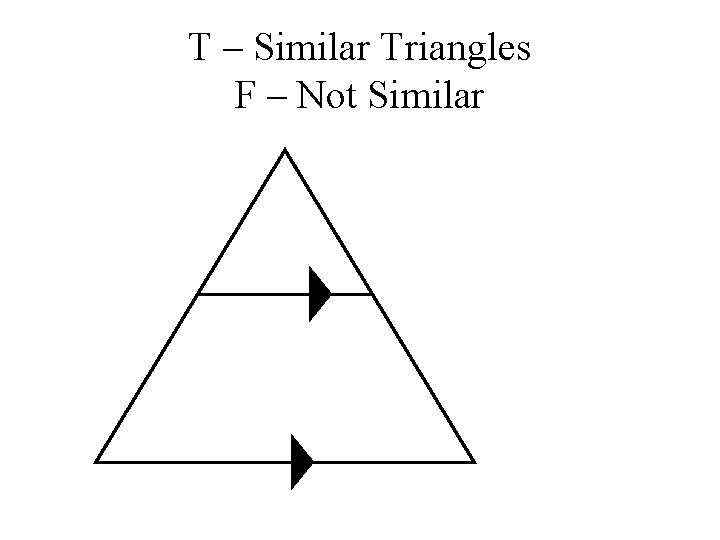
T – Similar Triangles F – Not Similar
T – Similar Triangles F – Not Similar
T – Similar Triangles F – Not Similar
Whiteboards • Page 256 – #9 – #11 – #13
brightstorm • Example
7 -5: Theorems for Similar Triangles Objectives • Learn about 2 additional ways to prove triangles are similar.
WARM-UP in NOTES What we have learned… • SAS Congruency – Write down in your own words what this means. • SSS Congruency – Write down in your own words what this means.
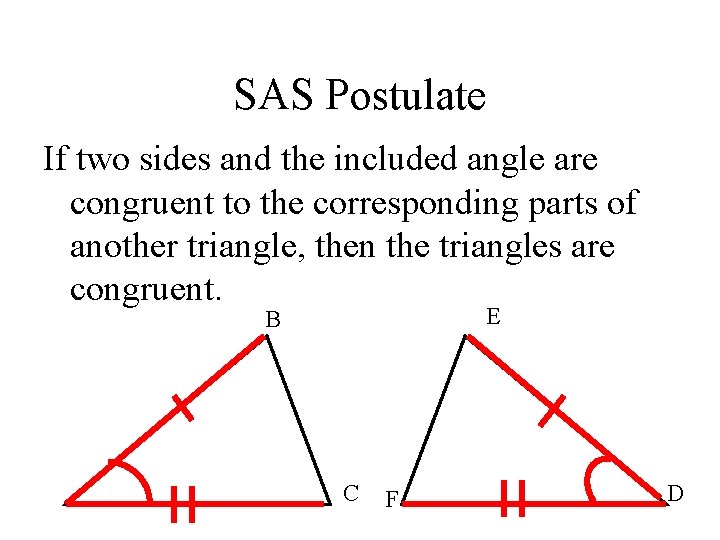
SAS Postulate If two sides and the included angle are congruent to the corresponding parts of another triangle, then the triangles are congruent. E B C F D
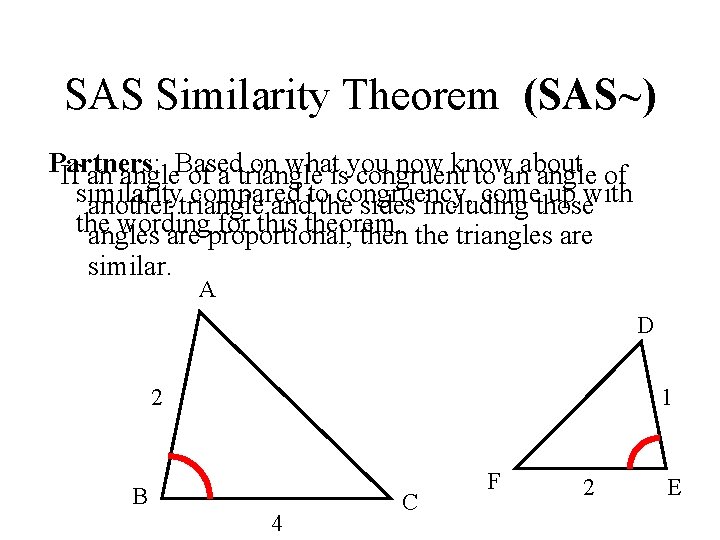
SAS Similarity Theorem (SAS~) Partners: on whatisyou now know If an angle. Based of a triangle congruent to anabout angle of similarity compared congruency, comethose up with another triangle andtothe sides including the wording for this theorem. angles are proportional, then the triangles are similar. A D 2 1 B 4 C F 2 E
- What is the scale factor of the ~ triangles - Name the triangles C - Name the postulate or theorem Included angle Scale Factor = 2/3 ▲CDE ~ ▲CAB by SAS ~ 6 D 3 10 E 5 A B
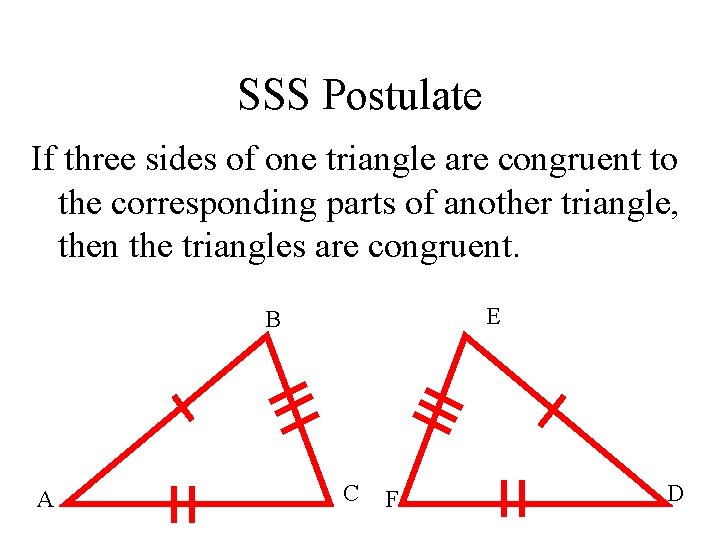
SSS Postulate If three sides of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. E B A C F D
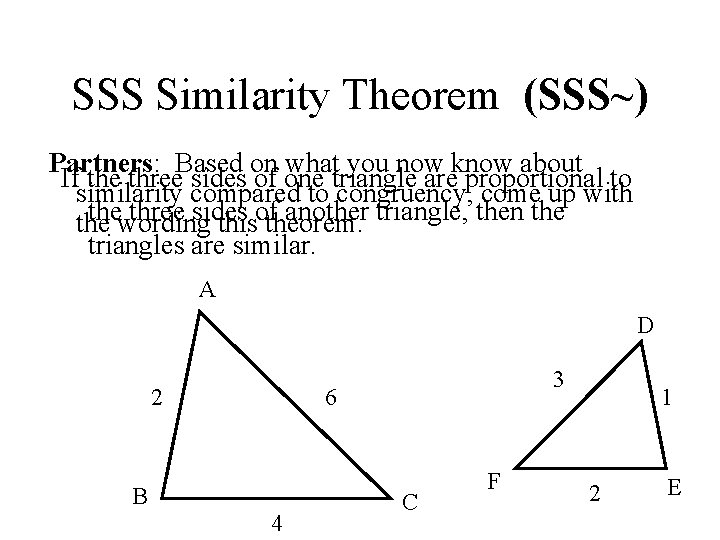
SSS Similarity Theorem (SSS~) Partners: Based on what you now know about If the three sides of one triangle are proportional to similarity compared to congruency, come up with thewording three sides another triangle, then the thisoftheorem. triangles are similar. A D 2 3 6 B 4 C F 1 2 E
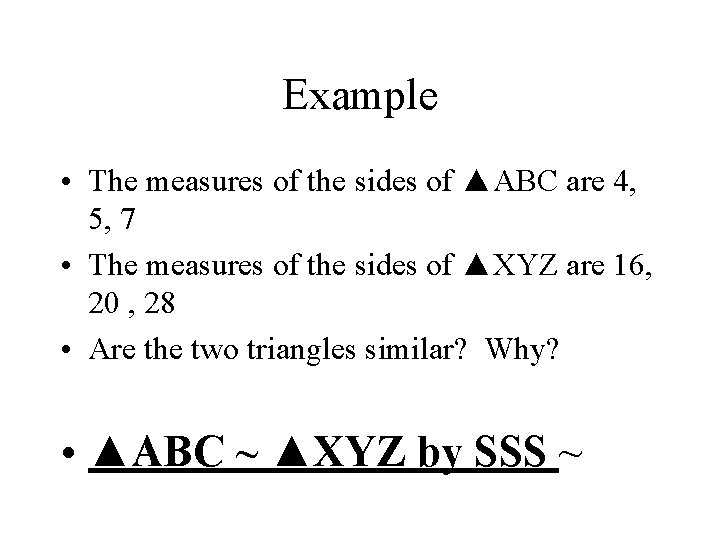
Example • The measures of the sides of ▲ABC are 4, 5, 7 • The measures of the sides of ▲XYZ are 16, 20 , 28 • Are the two triangles similar? Why? • ▲ABC ~ ▲XYZ by SSS ~
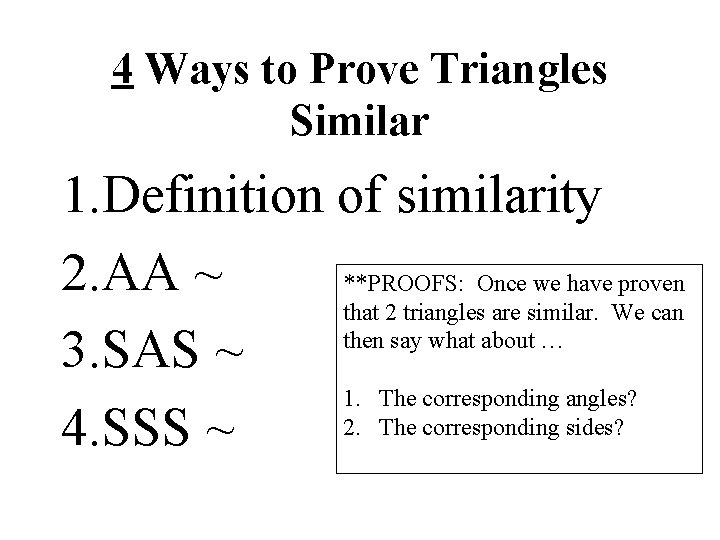
4 Ways to Prove Triangles Similar 1. Definition of similarity 2. AA ~ **PROOFS: Once we have proven that 2 triangles are similar. We can then say what about … 3. SAS ~ 1. The corresponding angles? 2. The corresponding sides? 4. SSS ~
White Board Practice • Name the similar triangles and give the postulate or theorem that justifies your answer…
A D 80◦ ▲ADE ~ ▲ABC by AA ~ E 80◦ B C
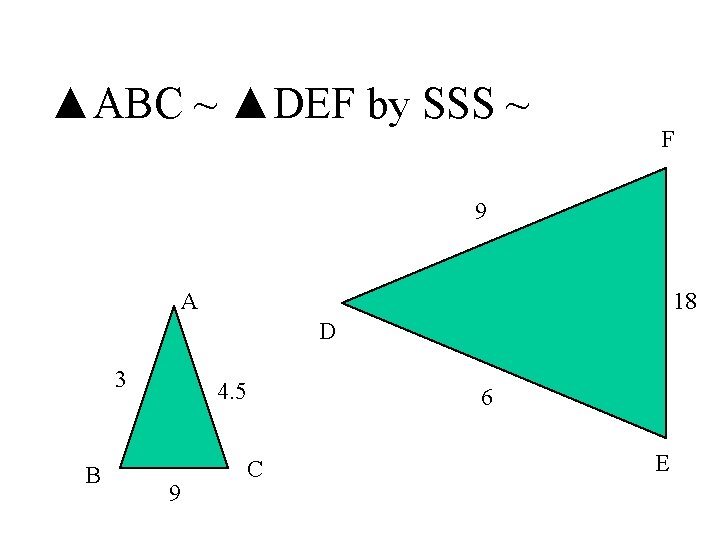
▲ABC ~ ▲DEF by SSS ~ F 9 A 18 D 3 B 4. 5 9 6 C E
Z 20 S 15 R 25 D 12 T ▲TRS ~ ▲ZRD by SAS ~
• You want to prove ▲RST ~ ▲ XYZ by SSS ~ – State the ratios that you know have to be equal to one another • You want to prove ▲RST ~ ▲ XYZ by SAS ~ – If you know LR congruent LX, what else do you need to prove?
7 -6: Proportional Lengths Objectives • Apply the Triangle Proportionality Theorem and its corollary • State and apply the Triangle Angle-bisector Theorem
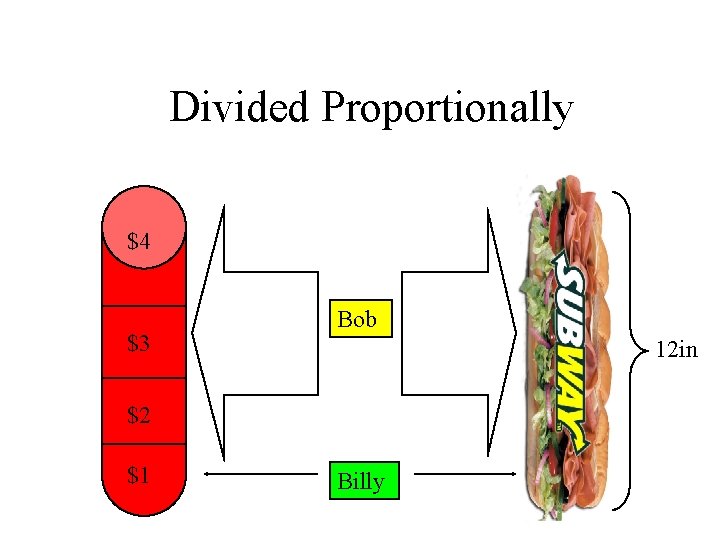
Billy and Bob Ø Billy and Bob want a foot-long sub from Subway that costs $4 Ø Billy has $1 and Bob has $3 Ø They combine their money and buy the sub Ø How much of the sub should each person get based on the amount of money they paid?
Divided Proportionally $4 $3 Bob 12 in $2 $1 Billy
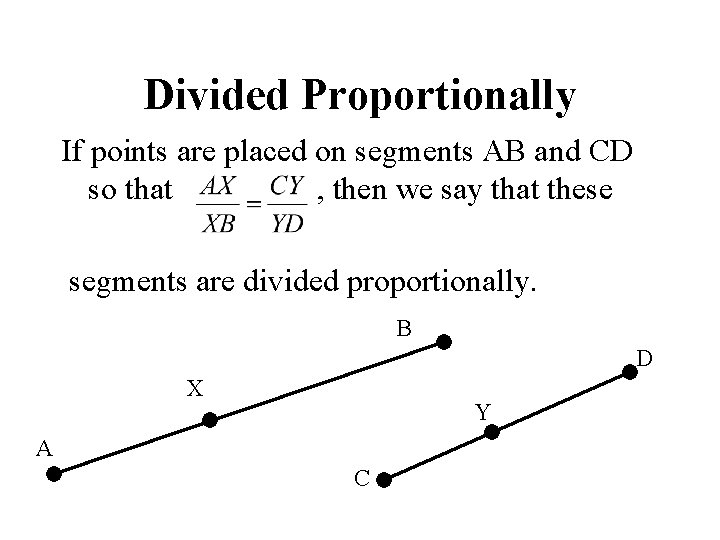
Divided Proportionally If points are placed on segments AB and CD so that , then we say that these segments are divided proportionally. B D X Y A C
Categorize the parts of each segment to help decide what can be matched up! Example B D X A 2 AX = CY XB YD 2 = 4 4 1 2 Y 1 C AX = XB CY YD 2 = 1 4 2 2 AX = CY AB CD 2 = 6 1 3 *Partners: determine another correct proportion as well as one that wouldn’t work*
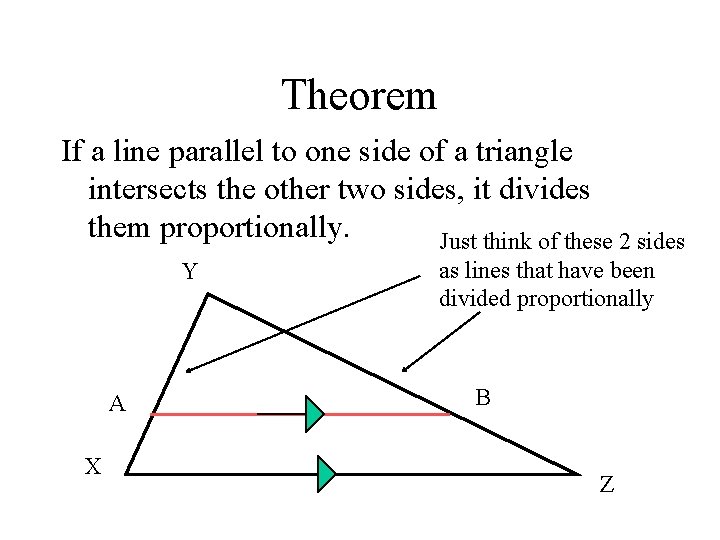
Theorem If a line parallel to one side of a triangle intersects the other two sides, it divides them proportionally. Just think of these 2 sides Y A X as lines that have been divided proportionally B Z
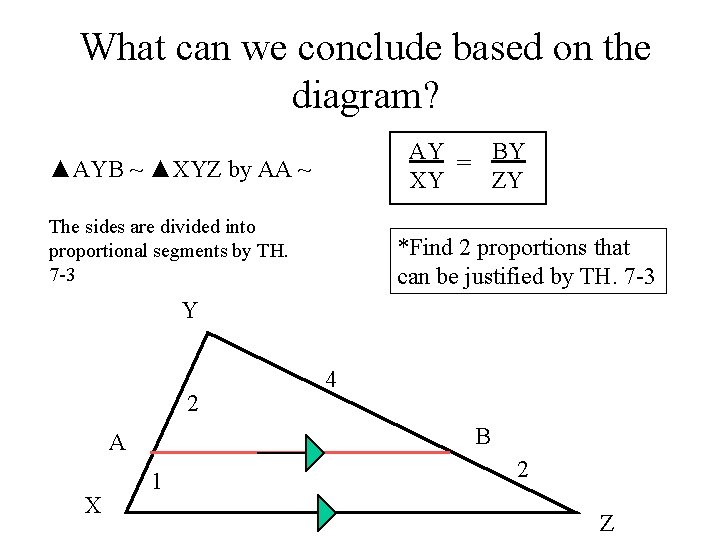
What can we conclude based on the diagram? AY = BY XY ZY ▲AYB ~ ▲XYZ by AA ~ The sides are divided into proportional segments by TH. 7 -3 *Find 2 proportions that can be justified by TH. 7 -3 Y 2 B A X 4 1 2 Z
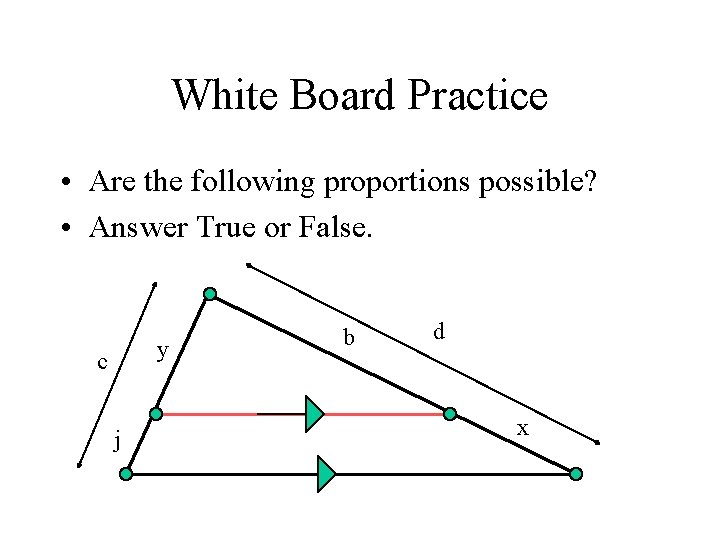
White Board Practice • Are the following proportions possible? • Answer True or False. y c j b d x
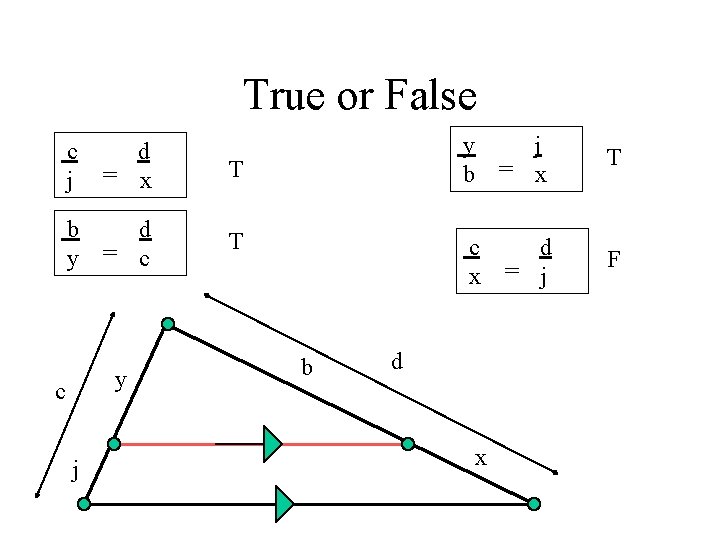
True or False c j d = x T b d y = c T y c j b y b j = x T c x d = j F d x
Corollary If. What threetheorem paralleldoes linesthis intersect tworemind transversals, diagram you of? then they divide the transversals proportionally. R W S T X RS = WX ST XY Y
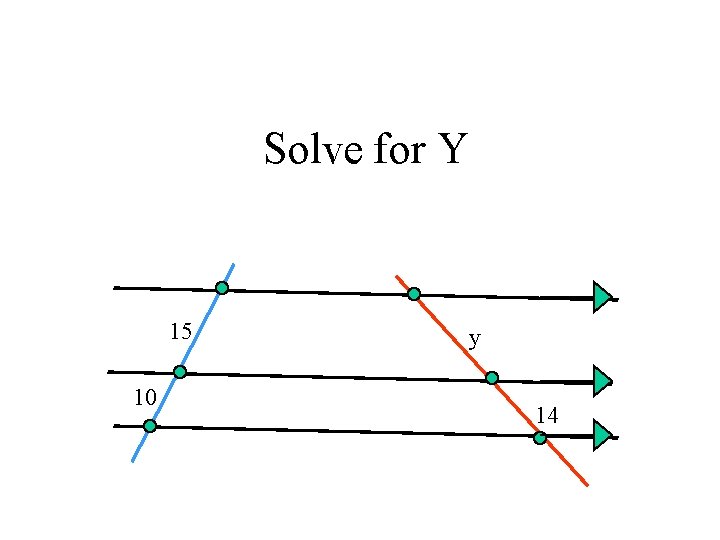
Solve for Y 15 10 y 14
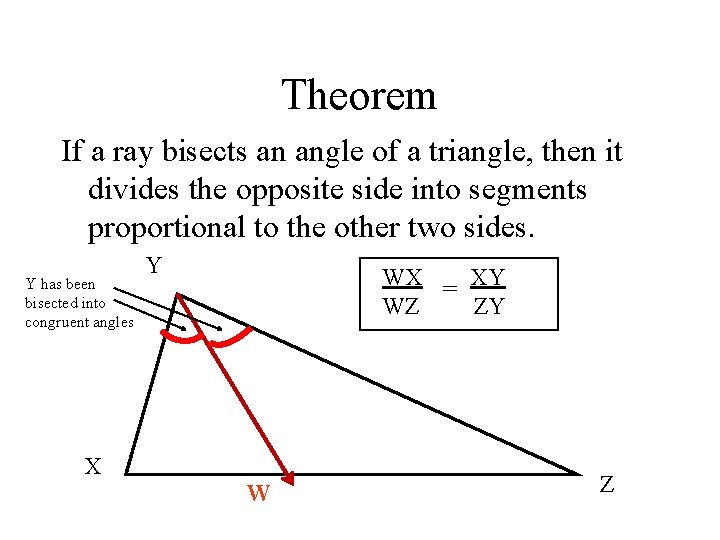
Theorem If a ray bisects an angle of a triangle, then it divides the opposite side into segments proportional to the other two sides. Y has been bisected into congruent angles Y WX = XY WZ ZY X W Z
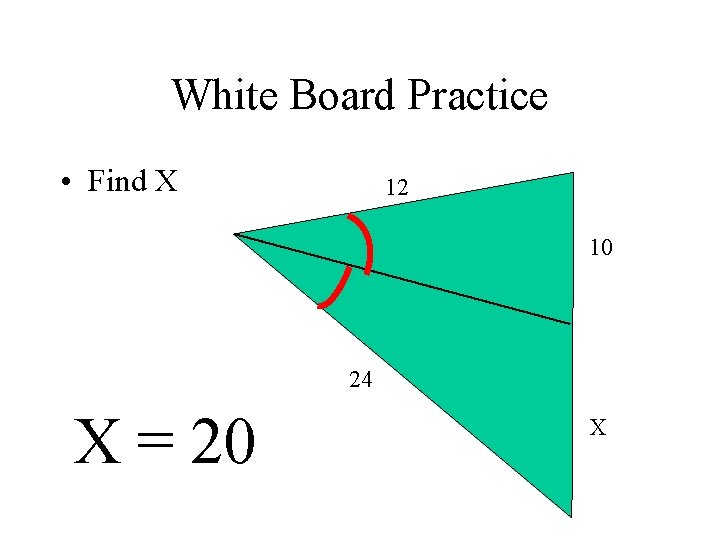
White Board Practice • Find X 12 10 24 X = 20 X
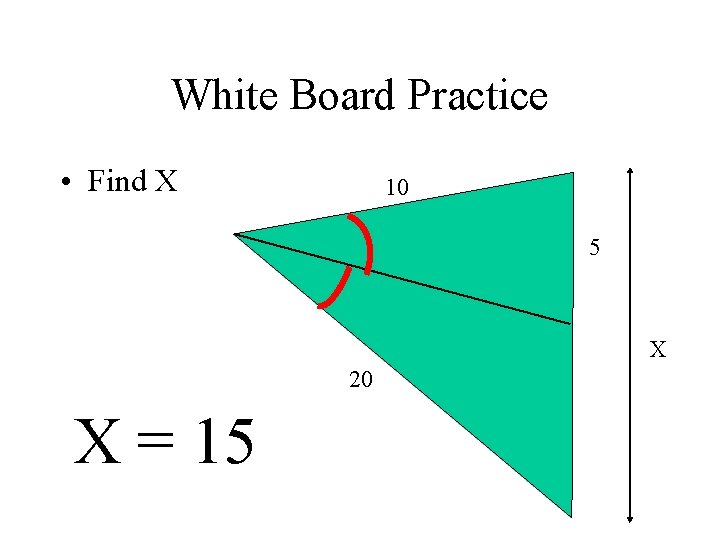
White Board Practice • Find X 10 5 X 20 X = 15
Ch. 7 Test Review Section 7. 1 • Putting ratios into simplest form • Find the measure of each angle based on a ratio – i. e. Pg. 244 #24 – 29 Section 7. 2 • Properties of proportions ( purple box pg. 245) – i. e. how can the proportion be changed around and still be equal to the original (i. e. pg. 247 # 1 -8) • Find the value of X ( Cross multiply and solve) – i. e. Pg. 247 #9 - 20
Ch. 7 Test Review Section 7. 3 • Understand the definition of similar polygons (~) • Finding the scale factor of similar polys – Compare the lengths of corresponding sides (reduce) • Use the scale factor to find unknown lengths – i. e. Pg. 251 #15 - 26 Section 7. 4 and 7. 5 • **pg. 258 #16** • Proving 2 triangles similar – AA ~ , SAS ~ , SSS ~ – i. e. pg. 266 # 1 – 6 – **REMEMBER ORDER MATTERS WHEN NAMING THE SIMILAR TRIANGLES!!!
Ch. 7 Test Review Section 7. 6 • Understand the 2 theorems and the corollary – i. e. P. 272 # 3 - 9 and 20 – 23 PROOFS • Study the following – Understand why a certain statement was given and its reason for it. • i. e. p 255 proof example • P. 266 # 11 - 16