Chapter 4 Quadratic Functions and Various Nonlinear Topics











- Slides: 30

Chapter 4 Quadratic Functions and Various Nonlinear Topics Section 4. 2

Section 4. 2 Parabolas and Their Applications • Definition of Quadratic Function • Parabolas: Features and Graphs • General and Vertex Forms of Quadratic Functions • Average Rate of Change

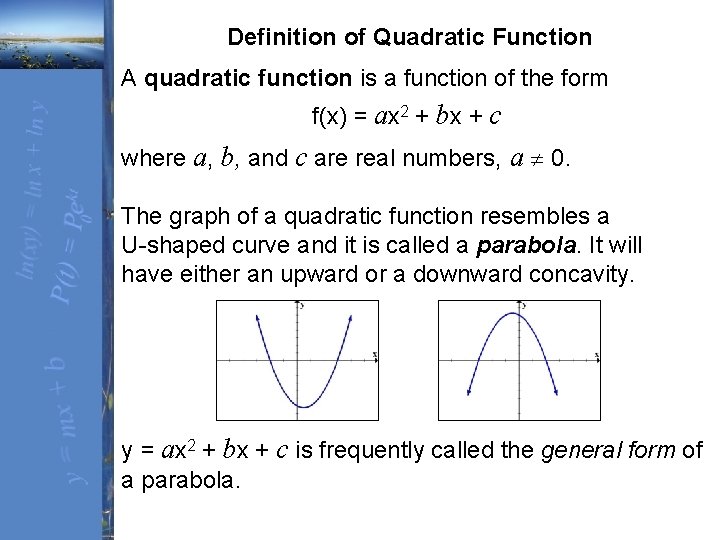
Definition of Quadratic Function A quadratic function is a function of the form f(x) = ax 2 + bx + c where a, b, and c are real numbers, a 0. The graph of a quadratic function resembles a U-shaped curve and it is called a parabola. It will have either an upward or a downward concavity. y = ax 2 + bx + c is frequently called the general form of a parabola.

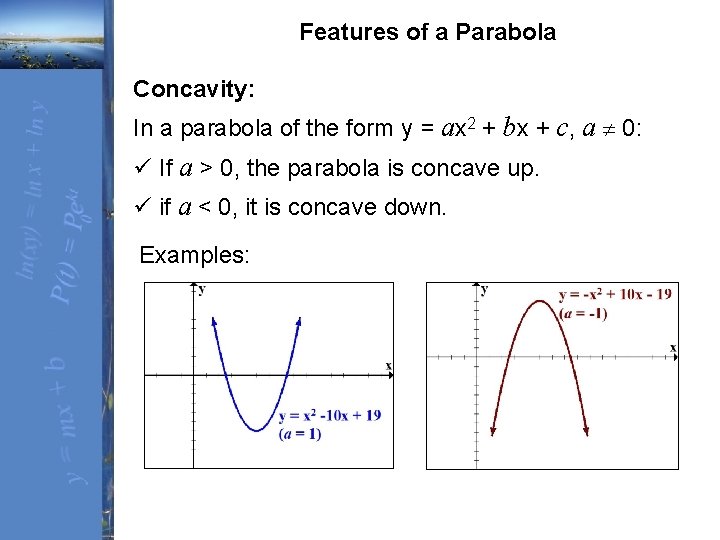
Features of a Parabola Concavity: In a parabola of the form y = ax 2 + bx + c, a 0: If a > 0, the parabola is concave up. if a < 0, it is concave down. Examples:

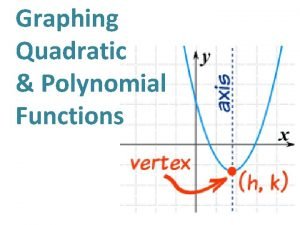
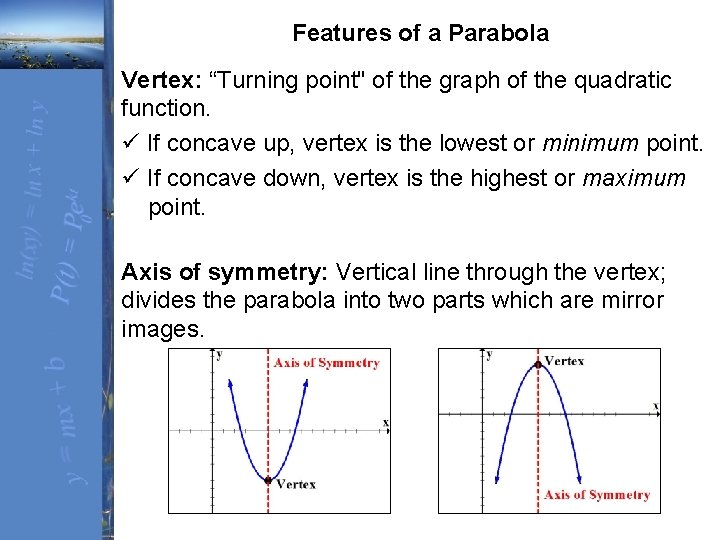
Features of a Parabola Vertex: “Turning point" of the graph of the quadratic function. If concave up, vertex is the lowest or minimum point. If concave down, vertex is the highest or maximum point. Axis of symmetry: Vertical line through the vertex; divides the parabola into two parts which are mirror images.

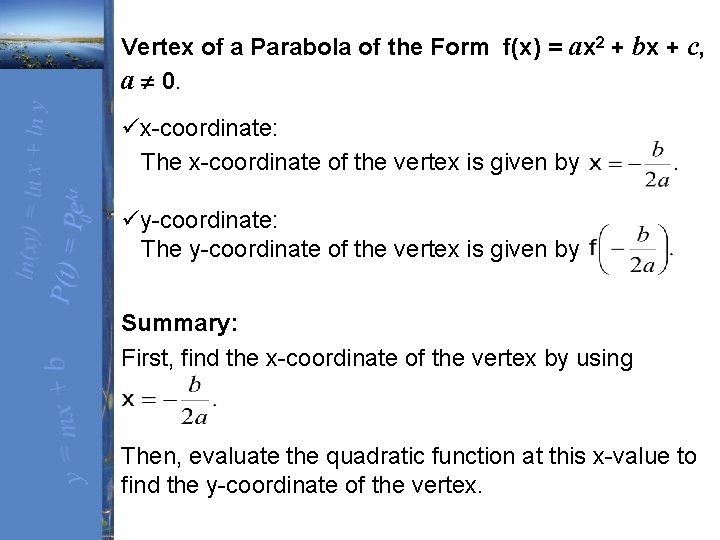
Vertex of a Parabola of the Form f(x) = ax 2 + bx + c, a 0. x-coordinate: The x-coordinate of the vertex is given by y-coordinate: The y-coordinate of the vertex is given by Summary: First, find the x-coordinate of the vertex by using Then, evaluate the quadratic function at this x-value to find the y-coordinate of the vertex.

Given the quadratic function f(x) = 3 x 2 – 12 x – 15, find the coordinates of the vertex and state whether it is a maximum or minimum point. x-coordinate of the vertex: y-coordinate of the vertex: Coordinates of the vertex: (2, – 27) a > 0, thus the parabola is concave up and the vertex is a minimum point.

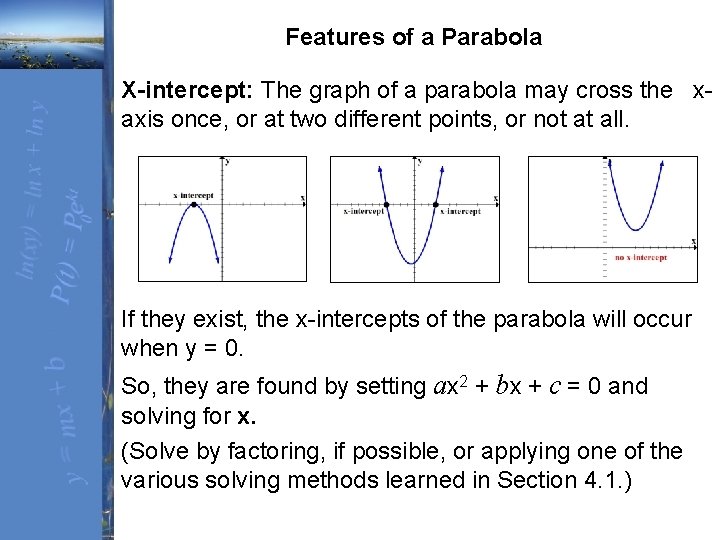
Features of a Parabola X-intercept: The graph of a parabola may cross the xaxis once, or at two different points, or not at all. If they exist, the x-intercepts of the parabola will occur when y = 0. So, they are found by setting ax 2 + bx + c = 0 and solving for x. (Solve by factoring, if possible, or applying one of the various solving methods learned in Section 4. 1. )

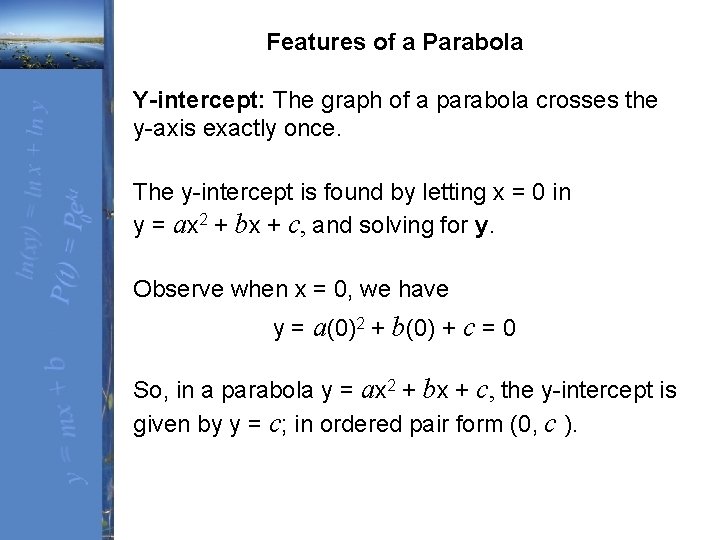
Features of a Parabola Y-intercept: The graph of a parabola crosses the y-axis exactly once. The y-intercept is found by letting x = 0 in y = ax 2 + bx + c, and solving for y. Observe when x = 0, we have y = a(0)2 + b(0) + c = 0 So, in a parabola y = ax 2 + bx + c, the y-intercept is given by y = c; in ordered pair form (0, c ).

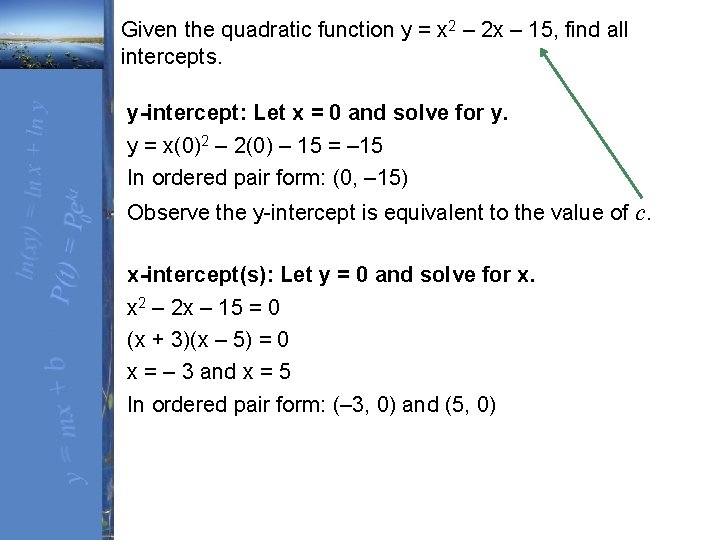
Given the quadratic function y = x 2 – 2 x – 15, find all intercepts. y-intercept: Let x = 0 and solve for y. y = x(0)2 – 2(0) – 15 = – 15 In ordered pair form: (0, – 15) Observe the y-intercept is equivalent to the value of c. x-intercept(s): Let y = 0 and solve for x. x 2 – 2 x – 15 = 0 (x + 3)(x – 5) = 0 x = – 3 and x = 5 In ordered pair form: (– 3, 0) and (5, 0)

Graphing a Parabola of the Form y = ax 2 + bx + c, a 0. 1. Determine the concavity: If a > 0, the parabola is concave up; if a < 0, concave down 2. Find the vertex: (–b/2 a, f(–b/2 a)) If concave up, the vertex is a minimum point If concave down, the vertex is a maximum point 3. Identify the y-intercept: f(0) is given by the value of c. 1. 4. Find any x-intercepts: Let y = 0 and solve for x. • Plot the vertex, the y-intercept, and x-intercepts (if any), and connect them with a smooth U-shaped curve. Show the axis of symmetry. (If needed, use the axis of symmetry to plot a symmetric point to the y-intercept to complete graph. )

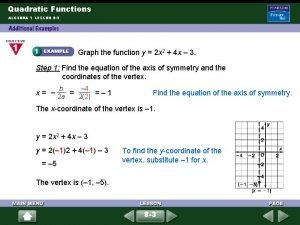
Graph the quadratic function f(x) = x 2 – 4 x – 5. 1. Concavity: a > 0, therefore, the parabola is concave up. 2. Vertex: (–b/2 a, f(–b/2 a)) x-coordinate: –b/2 a a = 1, b = – 4 So, x = –(– 4)/2(1) = 2 y-coordinate: f(–b/2 a) 2. So, f(2) = (2)2 – 4(2) – 5 = – 9 3. Coordinates of the Vertex: (2, – 9) 4. (continued on the next slide) 5.

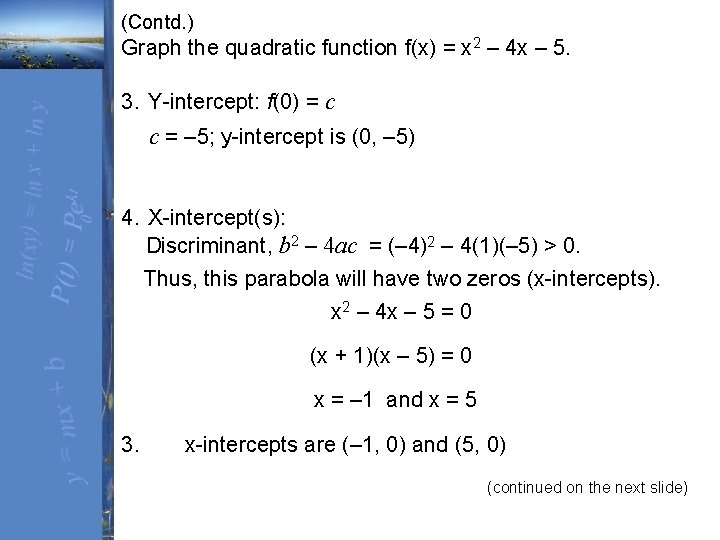
(Contd. ) Graph the quadratic function f(x) = x 2 – 4 x – 5. 3. Y-intercept: f(0) = c c = – 5; y-intercept is (0, – 5) 4. X-intercept(s): Discriminant, b 2 – 4 ac = (– 4)2 – 4(1)(– 5) > 0. Thus, this parabola will have two zeros (x-intercepts). x 2 – 4 x – 5 = 0 (x + 1)(x – 5) = 0 x = – 1 and x = 5 3. x-intercepts are (– 1, 0) and (5, 0) (continued on the next slide)

(Contd. ) The graph of the quadratic function f(x) = x 2 – 4 x – 5 is shown below:

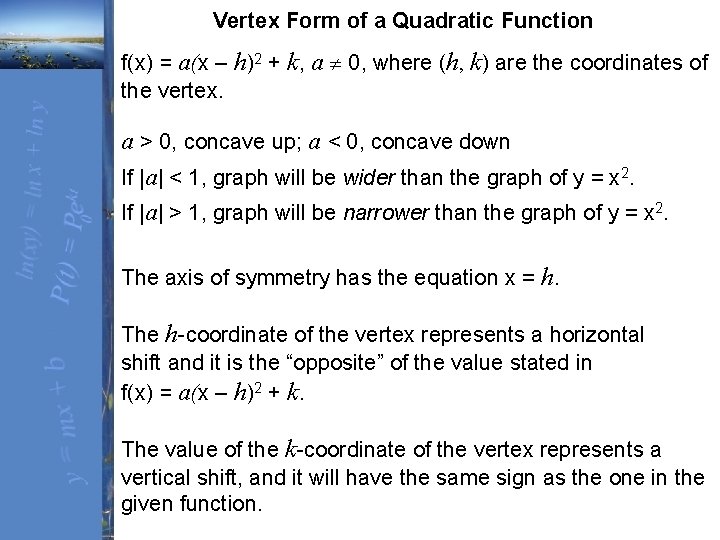
Vertex Form of a Quadratic Function f(x) = a(x – h)2 + k, a 0, where (h, k) are the coordinates of the vertex. a > 0, concave up; a < 0, concave down If |a| < 1, graph will be wider than the graph of y = x 2. If |a| > 1, graph will be narrower than the graph of y = x 2. The axis of symmetry has the equation x = h. The h-coordinate of the vertex represents a horizontal shift and it is the “opposite” of the value stated in f(x) = a(x – h)2 + k. The value of the k-coordinate of the vertex represents a vertical shift, and it will have the same sign as the one in the given function.

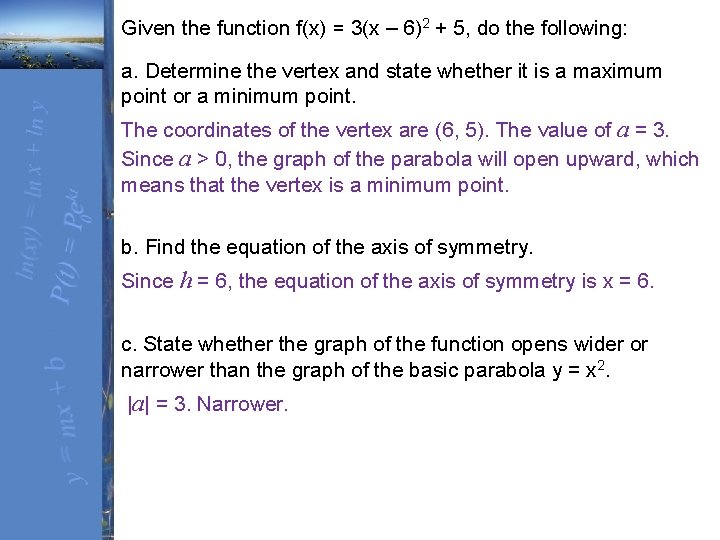
Given the function f(x) = 3(x – 6)2 + 5, do the following: a. Determine the vertex and state whether it is a maximum point or a minimum point. The coordinates of the vertex are (6, 5). The value of a = 3. Since a > 0, the graph of the parabola will open upward, which means that the vertex is a minimum point. b. Find the equation of the axis of symmetry. Since h = 6, the equation of the axis of symmetry is x = 6. c. State whether the graph of the function opens wider or narrower than the graph of the basic parabola y = x 2. |a| = 3. Narrower.

(Contd. ) d. Graph the given function, f(x) = 3(x – 6)2 + 5, and corroborate your answers to parts (a)-(c). e. State the domain and range of the given function. Domain: (–∞, ∞) Range: [5, ∞)

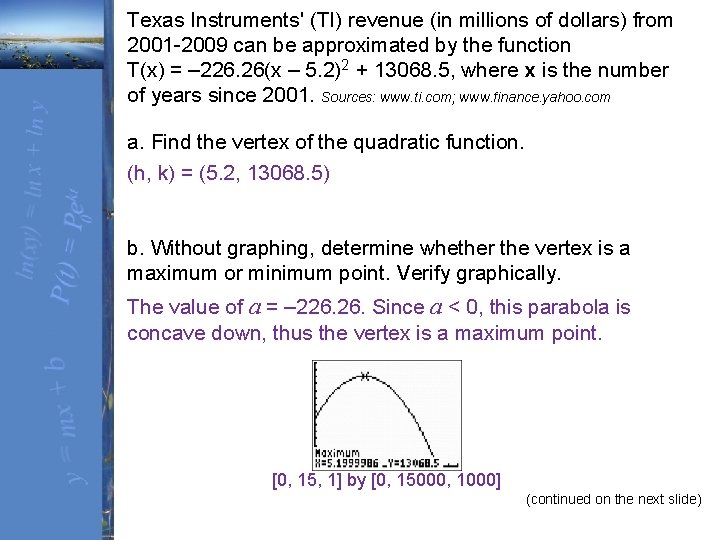
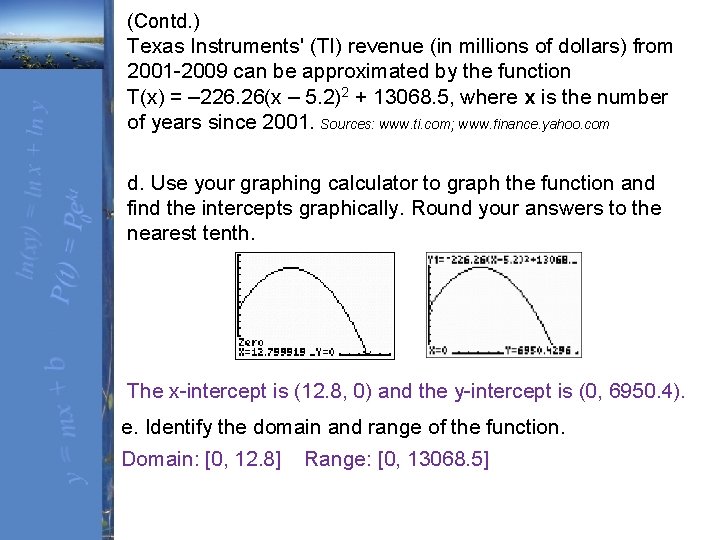
Texas Instruments' (TI) revenue (in millions of dollars) from 2001 -2009 can be approximated by the function T(x) = – 226. 26(x – 5. 2)2 + 13068. 5, where x is the number of years since 2001. Sources: www. ti. com; www. finance. yahoo. com a. Find the vertex of the quadratic function. (h, k) = (5. 2, 13068. 5) b. Without graphing, determine whether the vertex is a maximum or minimum point. Verify graphically. The value of a = – 226. Since a < 0, this parabola is concave down, thus the vertex is a maximum point. [0, 15, 1] by [0, 15000, 1000] (continued on the next slide)

(Contd. ) Texas Instruments' (TI) revenue (in millions of dollars) from 2001 -2009 can be approximated by the function T(x) = – 226. 26(x – 5. 2)2 + 13068. 5, where x is the number of years since 2001. Sources: www. ti. com; www. finance. yahoo. com c. Interpret the vertex as it applies to this problem. The vertex is given by (5. 2, 13068. 5). We know that x is the number of years since 2001, thus x = 5 represents 2006. In early 2006, the revenue was approximately $13068. 5 (in millions of dollars). (continued on the next slide)

(Contd. ) Texas Instruments' (TI) revenue (in millions of dollars) from 2001 -2009 can be approximated by the function T(x) = – 226. 26(x – 5. 2)2 + 13068. 5, where x is the number of years since 2001. Sources: www. ti. com; www. finance. yahoo. com d. Use your graphing calculator to graph the function and find the intercepts graphically. Round your answers to the nearest tenth. The x-intercept is (12. 8, 0) and the y-intercept is (0, 6950. 4). e. Identify the domain and range of the function. Domain: [0, 12. 8] Range: [0, 13068. 5]

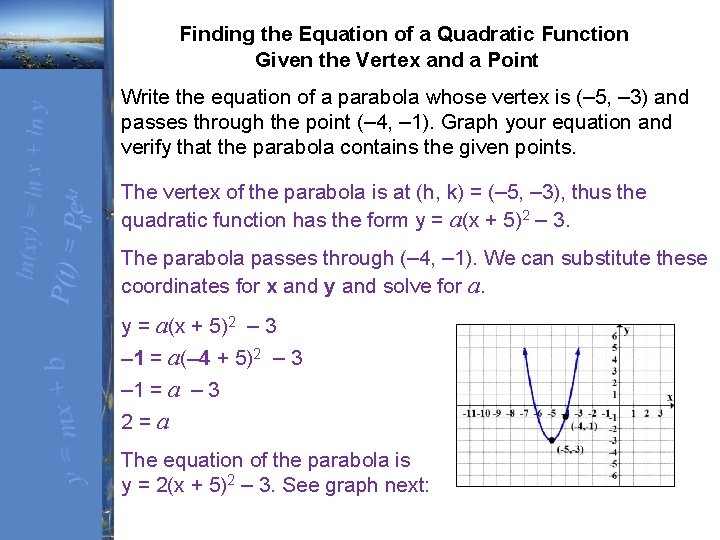
Finding the Equation of a Quadratic Function Given the Vertex and a Point Write the equation of a parabola whose vertex is (– 5, – 3) and passes through the point (– 4, – 1). Graph your equation and verify that the parabola contains the given points. The vertex of the parabola is at (h, k) = (– 5, – 3), thus the quadratic function has the form y = a(x + 5)2 – 3. The parabola passes through (– 4, – 1). We can substitute these coordinates for x and y and solve for a. y = a(x + 5)2 – 3 – 1 = a(– 4 + 5)2 – 3 – 1 = a – 3 2 = a The equation of the parabola is y = 2(x + 5)2 – 3. See graph next:

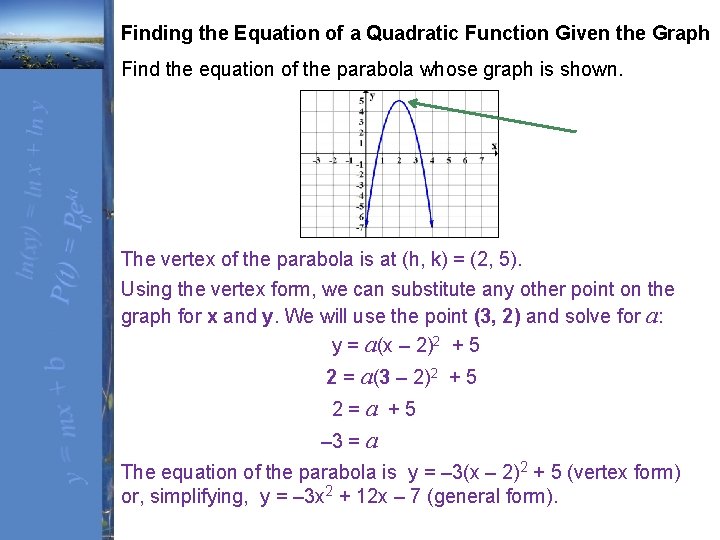
Finding the Equation of a Quadratic Function Given the Graph Find the equation of the parabola whose graph is shown. The vertex of the parabola is at (h, k) = (2, 5). Using the vertex form, we can substitute any other point on the graph for x and y. We will use the point (3, 2) and solve for a: y = a(x – 2)2 + 5 2 = a(3 – 2)2 + 5 2 = a + 5 – 3 = a The equation of the parabola is y = – 3(x – 2)2 + 5 (vertex form) or, simplifying, y = – 3 x 2 + 12 x – 7 (general form).

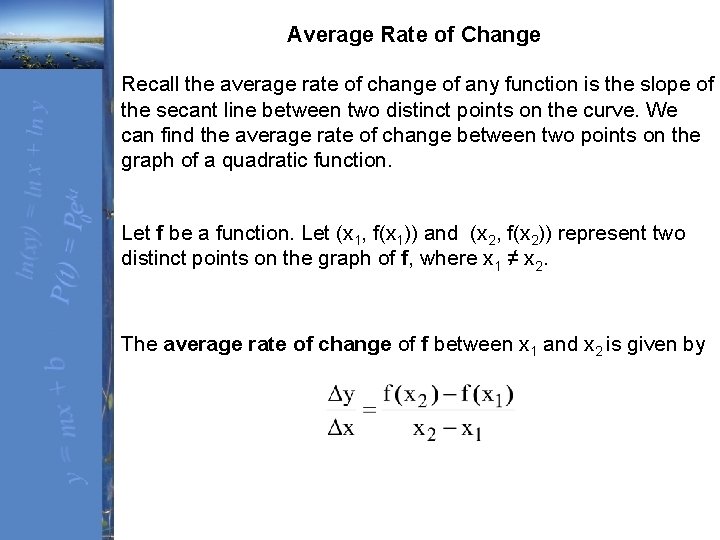
Average Rate of Change Recall the average rate of change of any function is the slope of the secant line between two distinct points on the curve. We can find the average rate of change between two points on the graph of a quadratic function. Let f be a function. Let (x 1, f(x 1)) and (x 2, f(x 2)) represent two distinct points on the graph of f, where x 1 ≠ x 2. The average rate of change of f between x 1 and x 2 is given by

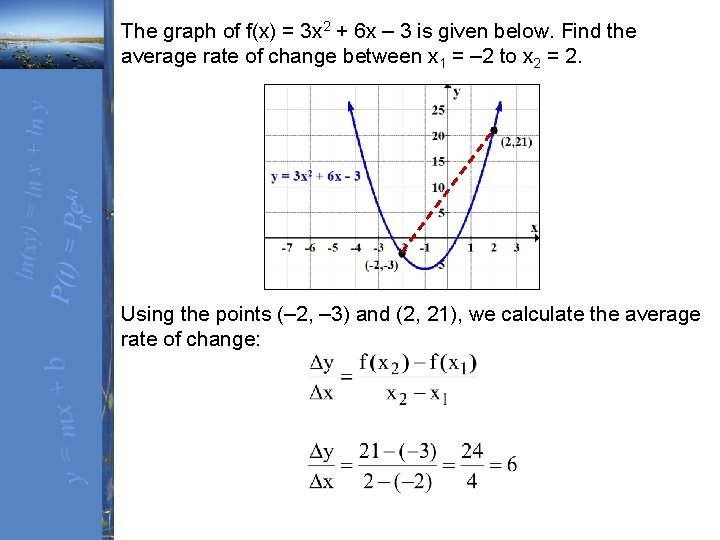
The graph of f(x) = 3 x 2 + 6 x – 3 is given below. Find the average rate of change between x 1 = – 2 to x 2 = 2. Using the points (– 2, – 3) and (2, 21), we calculate the average rate of change:

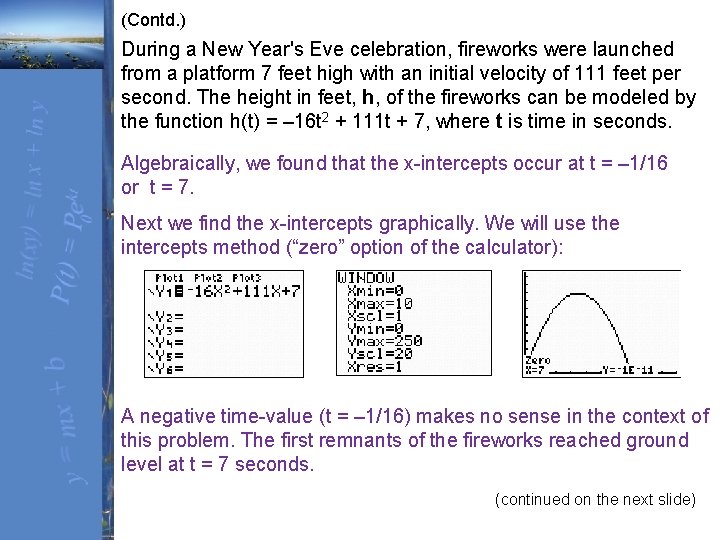
During a New Year's Eve celebration, fireworks were launched from a platform 7 feet high with an initial velocity of 111 feet per second. The height in feet, h, of the fireworks can be modeled by the function h(t) = – 16 t 2 + 111 t + 7, where t is time in seconds. a. Find the vertex of the quadratic function. Round your answer to the nearest tenth and interpret using a complete sentence. Vertex: (–b/2 a, h(–b/2 a)) t = – 111/2(– 16) = 3. 5 h(3. 5) = – 16(3. 5)2 + 111(3. 5) + 7 = 199. 5 Coordinates of the vertex: (3. 5, 199. 5) The fireworks reached a maximum height of 199. 5 feet after 3. 5 seconds. (continued on the next slide)

(Contd. ) During a New Year's Eve celebration, fireworks were launched from a platform 7 feet high with an initial velocity of 111 feet per second. The height in feet, h, of the fireworks can be modeled by the function h(t) = – 16 t 2 + 111 t + 7, where t is time in seconds. b. Find any x-intercept(s) algebraically and graphically. Interpret using a complete sentence. Let h(t) = 0 and solve the quadratic equation for t. – 16 t 2 + 111 t + 7 = 0 16 t 2 – 111 t – 7 = 0 (Divide both sides by – 1) (16 t + 1)(t – 7) = 0 16 t + 1 = 0 or t – 7 = 0 t = – 1/16 or t = 7 (continued on the next slide)

(Contd. ) During a New Year's Eve celebration, fireworks were launched from a platform 7 feet high with an initial velocity of 111 feet per second. The height in feet, h, of the fireworks can be modeled by the function h(t) = – 16 t 2 + 111 t + 7, where t is time in seconds. Algebraically, we found that the x-intercepts occur at t = – 1/16 or t = 7. Next we find the x-intercepts graphically. We will use the intercepts method (“zero” option of the calculator): A negative time-value (t = – 1/16) makes no sense in the context of this problem. The first remnants of the fireworks reached ground level at t = 7 seconds. (continued on the next slide)

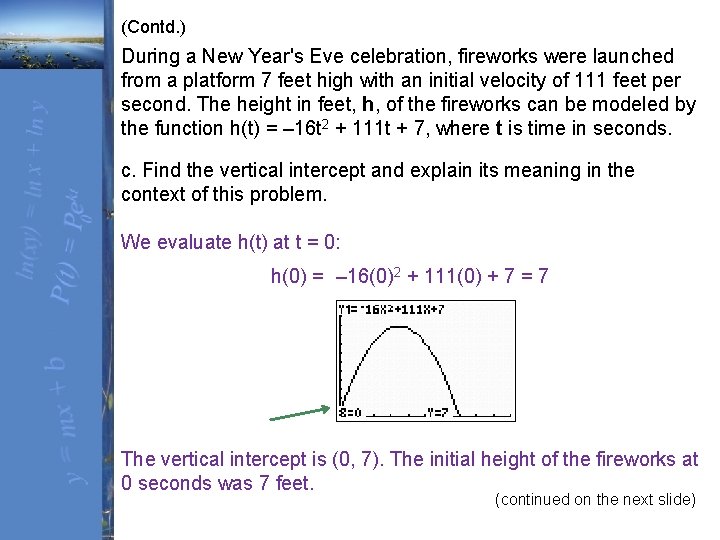
(Contd. ) During a New Year's Eve celebration, fireworks were launched from a platform 7 feet high with an initial velocity of 111 feet per second. The height in feet, h, of the fireworks can be modeled by the function h(t) = – 16 t 2 + 111 t + 7, where t is time in seconds. c. Find the vertical intercept and explain its meaning in the context of this problem. We evaluate h(t) at t = 0: h(0) = – 16(0)2 + 111(0) + 7 = 7 The vertical intercept is (0, 7). The initial height of the fireworks at 0 seconds was 7 feet. (continued on the next slide)

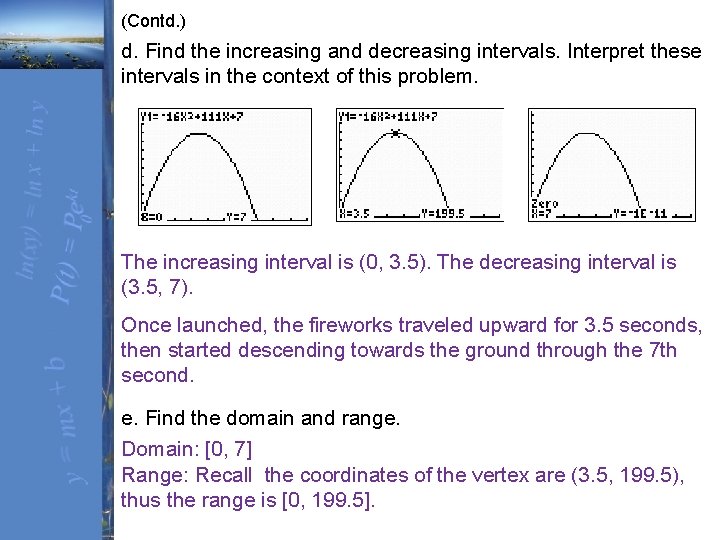
(Contd. ) d. Find the increasing and decreasing intervals. Interpret these intervals in the context of this problem. The increasing interval is (0, 3. 5). The decreasing interval is (3. 5, 7). Once launched, the fireworks traveled upward for 3. 5 seconds, then started descending towards the ground through the 7 th second. e. Find the domain and range. Domain: [0, 7] Range: Recall the coordinates of the vertex are (3. 5, 199. 5), thus the range is [0, 199. 5].

Using your textbook, practice the problems assigned by your instructor to review the concepts from Section 4. 2.
 Non linear quadratic equations
Non linear quadratic equations Linear or nonlinear table
Linear or nonlinear table Patterns and nonlinear functions
Patterns and nonlinear functions Chapter 9 quadratic equations and functions
Chapter 9 quadratic equations and functions Chapter 8: quadratic functions and equations answer key
Chapter 8: quadratic functions and equations answer key Exploring quadratic graphs
Exploring quadratic graphs Chapter 3 linear and quadratic functions
Chapter 3 linear and quadratic functions Chapter 1 quadratic functions and factoring
Chapter 1 quadratic functions and factoring Classification of management
Classification of management Lesson 8-1 identifying quadratic functions
Lesson 8-1 identifying quadratic functions Linear and quadratic functions and modeling
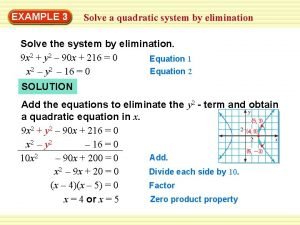
Linear and quadratic functions and modeling How to solve quadratic equations by elimination
How to solve quadratic equations by elimination Find the discriminant
Find the discriminant Crime scene factoring and quadratic functions answer key
Crime scene factoring and quadratic functions answer key Linear quadratic exponential tables
Linear quadratic exponential tables Quadratic function maximum
Quadratic function maximum Quadratic functions and their graphs
Quadratic functions and their graphs Crime scene factoring and quadratic functions answer key
Crime scene factoring and quadratic functions answer key Function in standard form
Function in standard form Y^2=ax^2+bx+c
Y^2=ax^2+bx+c Relative vs absolute max and min
Relative vs absolute max and min What is the domain of a parabola
What is the domain of a parabola Solving graphing and analyzing quadratic functions
Solving graphing and analyzing quadratic functions 4-1 quadratic functions and transformations
4-1 quadratic functions and transformations Quadratic functions: vertex form assignment
Quadratic functions: vertex form assignment Transformations of quadratic functions
Transformations of quadratic functions Graph using transformations
Graph using transformations Quadratics facts
Quadratics facts Introduction to quadratic function
Introduction to quadratic function 8-3 graphing quadratic functions
8-3 graphing quadratic functions Quadratic formula vocabulary words
Quadratic formula vocabulary words