Chapter 2 Motion Along a Line Motion Along

Chapter 2 Motion Along a Line

Motion Along a Line • Position & Displacement • Speed & Velocity • Acceleration • Describing motion in 1 D • Free Fall

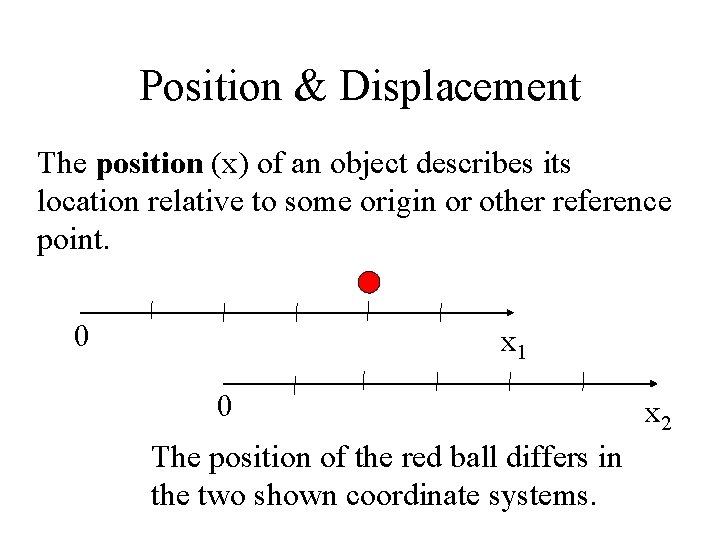
Position & Displacement The position (x) of an object describes its location relative to some origin or other reference point. 0 x 1 0 The position of the red ball differs in the two shown coordinate systems. x 2

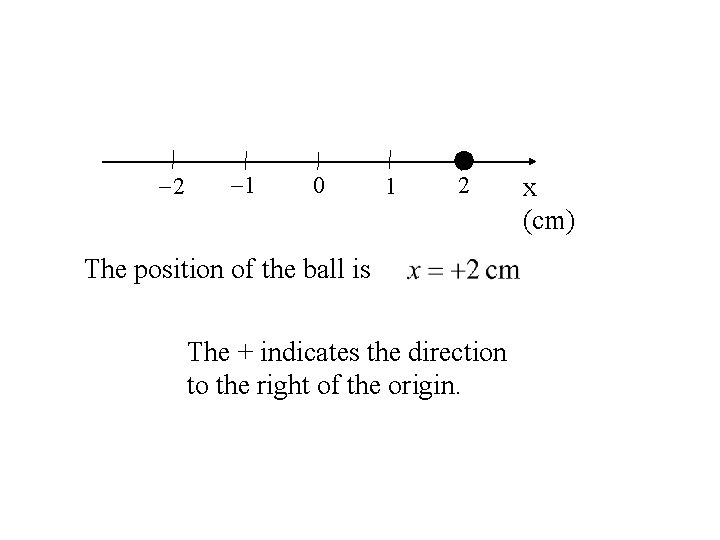
2 1 0 1 2 The position of the ball is The + indicates the direction to the right of the origin. x (cm)

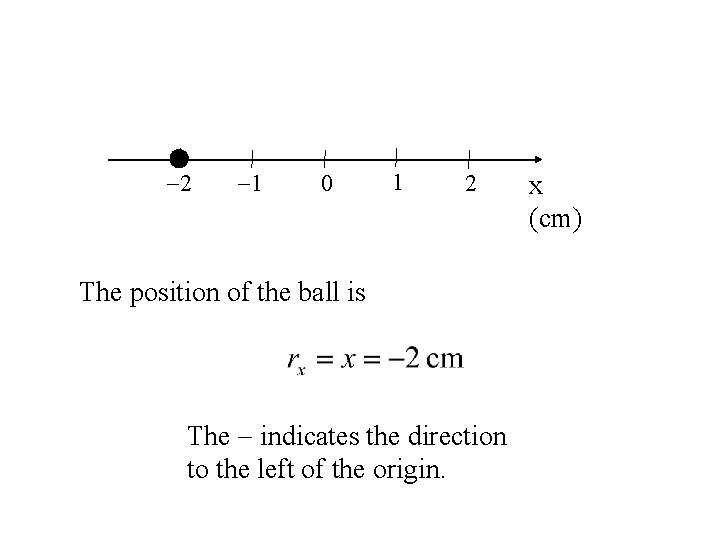
2 1 0 1 2 The position of the ball is The indicates the direction to the left of the origin. x (cm)

The displacement is the change in an object’s position. It depends only on the beginning and ending positions. All Δ quantities will have the final value 1 st and the inital value last.

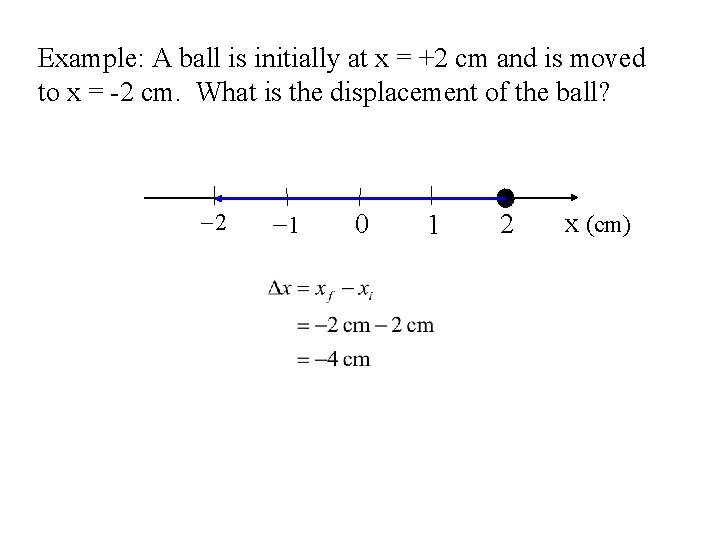
Example: A ball is initially at x = +2 cm and is moved to x = -2 cm. What is the displacement of the ball? 2 1 0 1 2 x (cm)

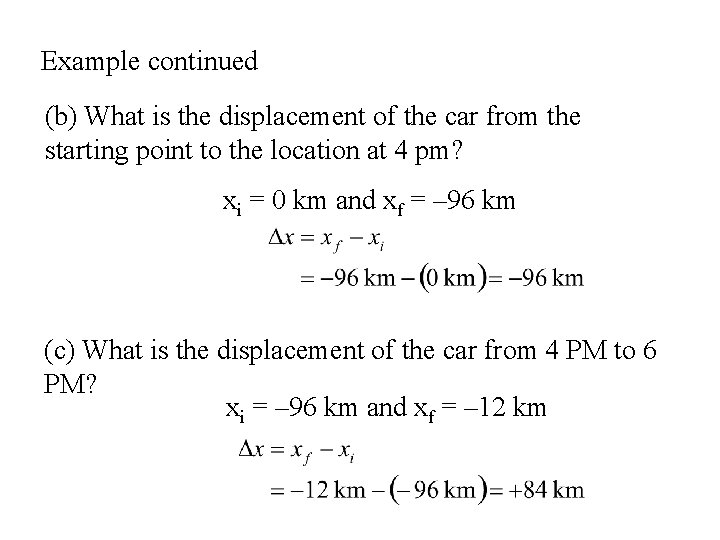
Example: At 3 PM a car is located 20 km south of its starting point. One hour later its is 96 km farther south. After two more hours it is 12 km south of the original starting point. (a) What is the displacement of the car between 3 PM and 6 PM? Use a coordinate system where north is positive. xi = – 20 km and xf = – 12 km

Example continued (b) What is the displacement of the car from the starting point to the location at 4 pm? xi = 0 km and xf = – 96 km (c) What is the displacement of the car from 4 PM to 6 PM? xi = – 96 km and xf = – 12 km

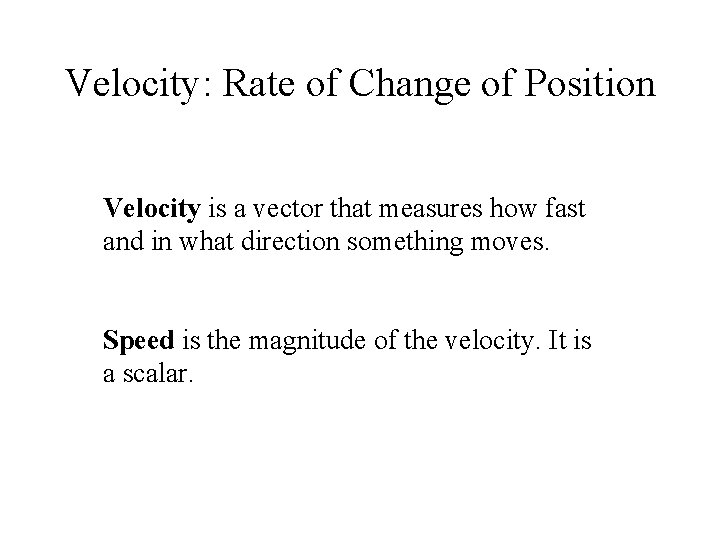
Velocity: Rate of Change of Position Velocity is a vector that measures how fast and in what direction something moves. Speed is the magnitude of the velocity. It is a scalar.

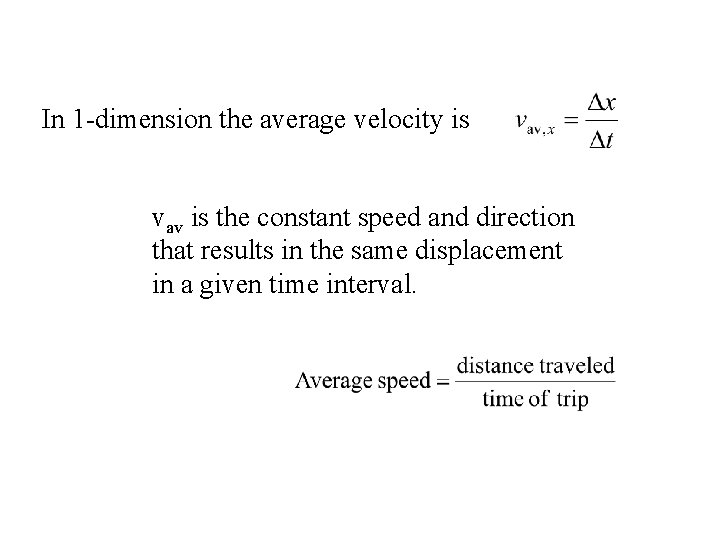
In 1 -dimension the average velocity is vav is the constant speed and direction that results in the same displacement in a given time interval.

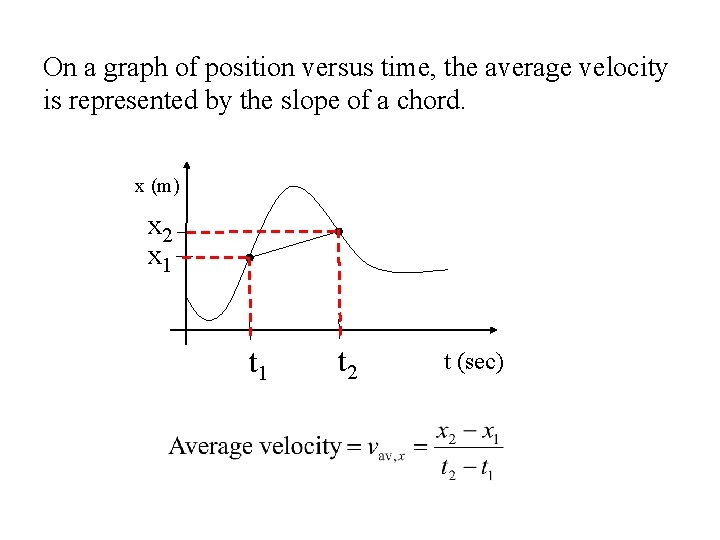
On a graph of position versus time, the average velocity is represented by the slope of a chord. x (m) x 2 x 1 t 2 t (sec)

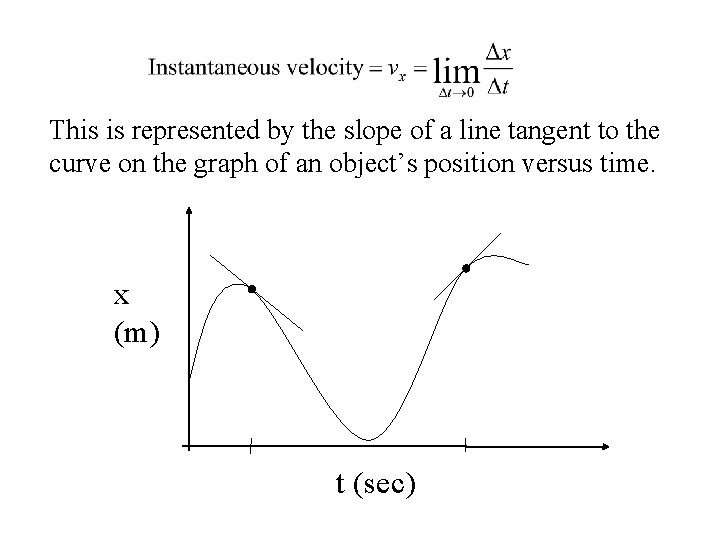
This is represented by the slope of a line tangent to the curve on the graph of an object’s position versus time. x (m) t (sec)

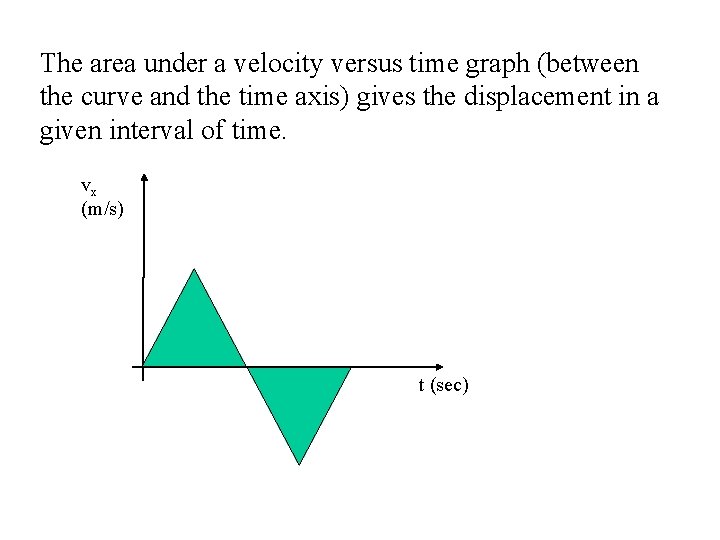
The area under a velocity versus time graph (between the curve and the time axis) gives the displacement in a given interval of time. vx (m/s) t (sec)

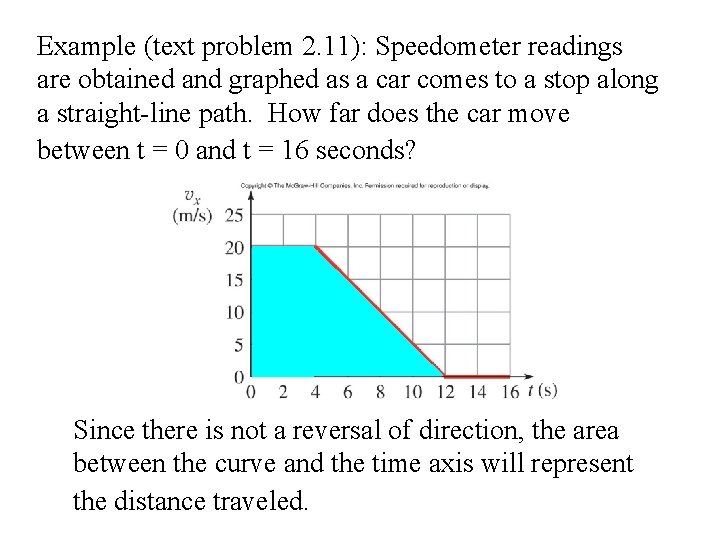
Example (text problem 2. 11): Speedometer readings are obtained and graphed as a car comes to a stop along a straight-line path. How far does the car move between t = 0 and t = 16 seconds? Since there is not a reversal of direction, the area between the curve and the time axis will represent the distance traveled.

Example continued: The rectangular portion has an area of Lw = (20 m/s)(4 s) = 80 m. The triangular portion has an area of ½bh = ½(8 s) (20 m/s) = 80 m. Thus, the total area is 160 m. This is the distance traveled by the car.

Acceleration: Rate of Change of Velocity These have interpretation s similar to vav and v.

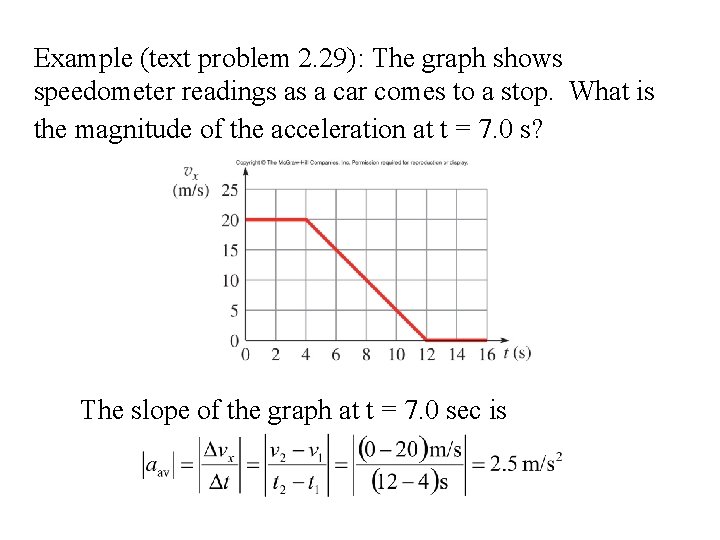
Example (text problem 2. 29): The graph shows speedometer readings as a car comes to a stop. What is the magnitude of the acceleration at t = 7. 0 s? The slope of the graph at t = 7. 0 sec is

Motion Along a Line With Constant Acceleration For constant acceleration the kinematic equations are: Also:

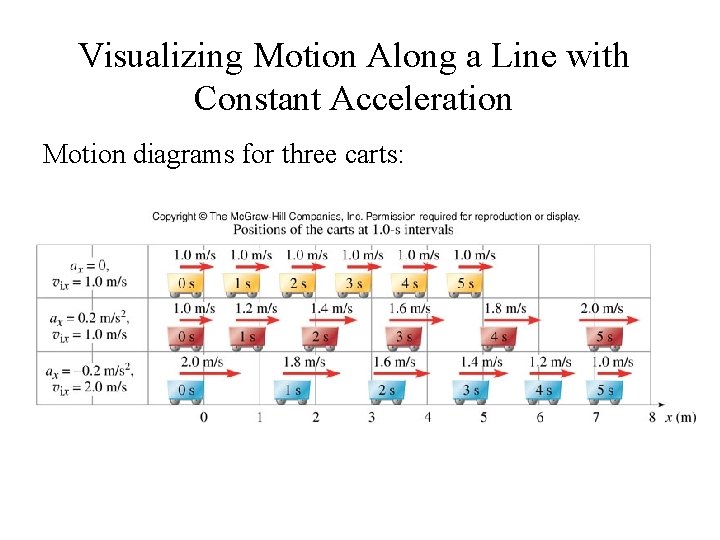
Visualizing Motion Along a Line with Constant Acceleration Motion diagrams for three carts:

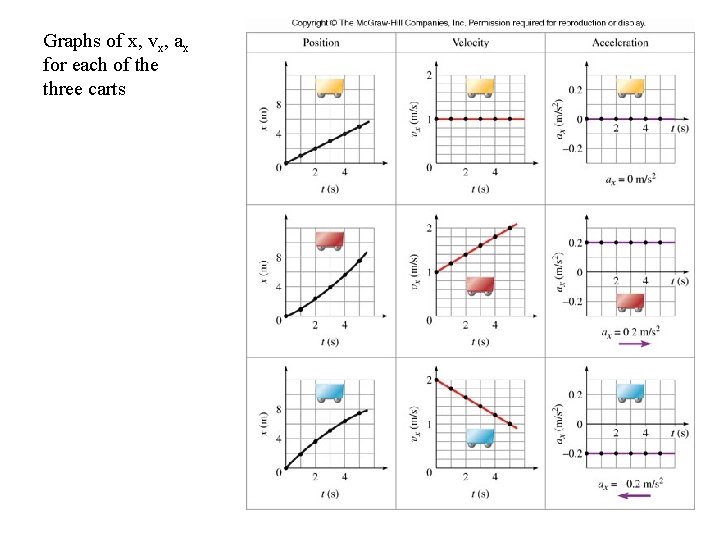
Graphs of x, vx, ax for each of the three carts

Free Fall A stone is dropped from the edge of a cliff; if air resistance can be ignored, we say the stone is in free fall. The magnitude of the acceleration of the stone is afree fall = g = 9. 80 m/s 2, this acceleration is always directed toward the Earth.

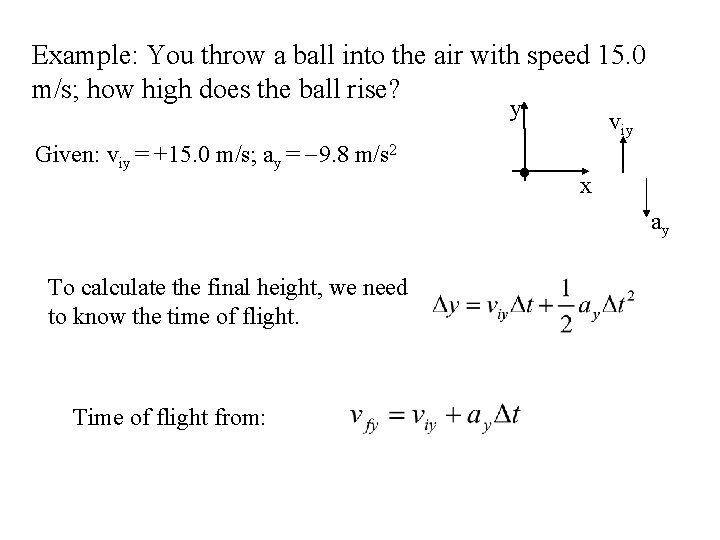
Example: You throw a ball into the air with speed 15. 0 m/s; how high does the ball rise? y Given: viy = +15. 0 m/s; ay = 9. 8 m/s 2 viy x ay To calculate the final height, we need to know the time of flight. Time of flight from:

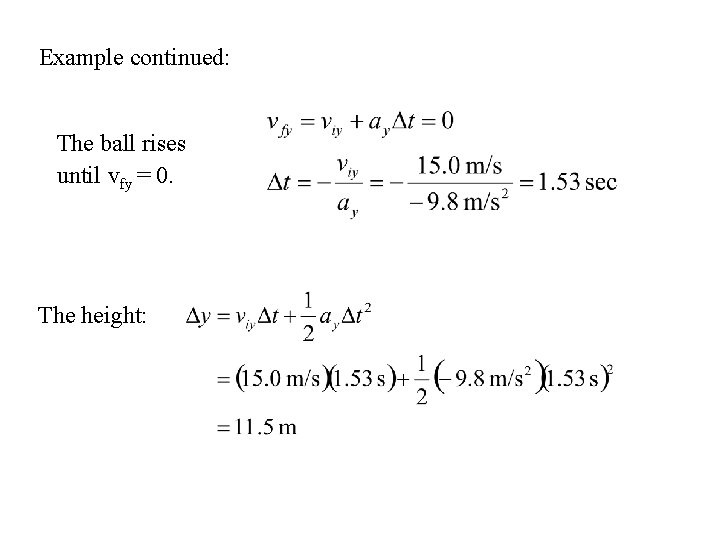
Example continued: The ball rises until vfy = 0. The height:

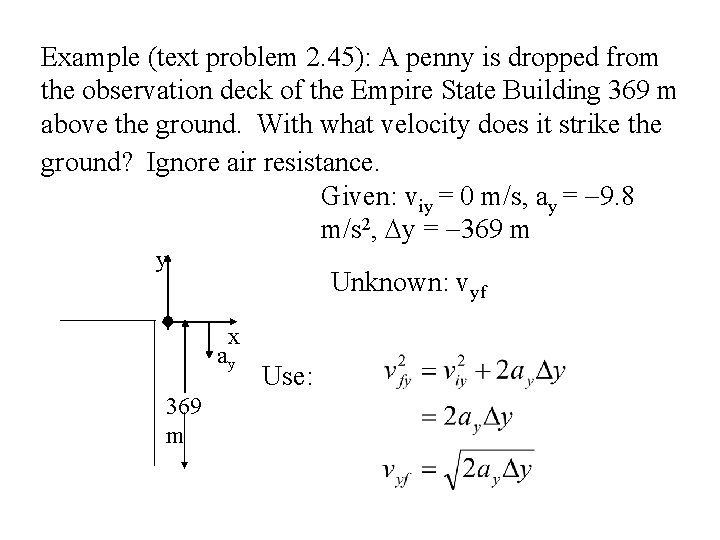
Example (text problem 2. 45): A penny is dropped from the observation deck of the Empire State Building 369 m above the ground. With what velocity does it strike the ground? Ignore air resistance. Given: viy = 0 m/s, ay = 9. 8 m/s 2, y = 369 m y Unknown: vyf x ay 369 m Use:

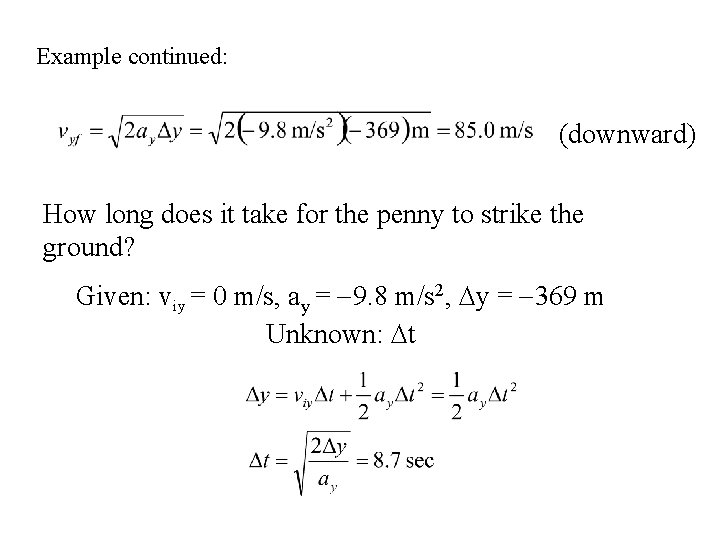
Example continued: (downward) How long does it take for the penny to strike the ground? Given: viy = 0 m/s, ay = 9. 8 m/s 2, y = 369 m Unknown: t

Summary • Position • Displacement Versus Distance • Velocity Versus Speed • Acceleration • Instantaneous Values Versus Average Values • The Kinematic Equations • Graphical Representations of Motion • Free fall