Chapter 2 2 Optical Fiber Waveguide 1 Optical

Chapter 2. 2 Optical Fiber Waveguide 1

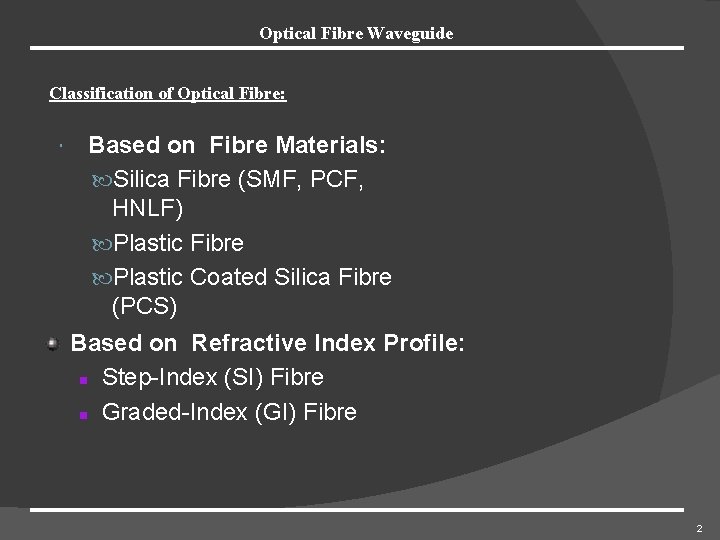
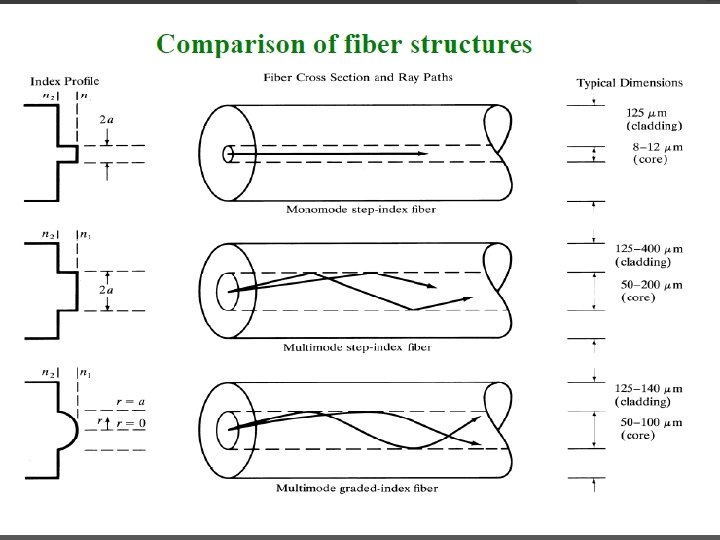
Optical Fibre Waveguide Classification of Optical Fibre: Based on Fibre Materials: Silica Fibre (SMF, PCF, HNLF) Plastic Fibre Plastic Coated Silica Fibre (PCS) Based on Refractive Index Profile: n Step-Index (SI) Fibre n Graded-Index (GI) Fibre 2

Optical Fibre Waveguide SINGLE MODE FIBRE: • Only one propagation mode (due to smaller core diameter). • Mode of propagation is typically the HE 11 Mode ( lowest order mode in optical fiber • Core thickness is about 2 to 10 Micron. ADVANTAGES: Larger Bandwidth due to low intermodal dispersion. DISADVANTAGES: Spatially incoherent optical sources won’t couple efficiently into single-mode fibres. 3

Optical Fibre Waveguide MULTI MODE FIBRES: • Can support a few propagation modes. • Core thickness is about 50 Micron. ADVANTAGES: • Able to use spatially incoherent optical sources. • Larger numerical aperture. • Easier to align and couple optical source. • Lower tolerance requirements on fibre connectors. DISADVANTAGES: • Lesser data rates. • Suffer from modal dispersion. 4

5

Assignment 1 (dateline 5/3/2013) 6

Step-Index (SI) Fibre n Graded-Index (GI) Fibre n 7

What are the differences between step index and graded index fibers? 8

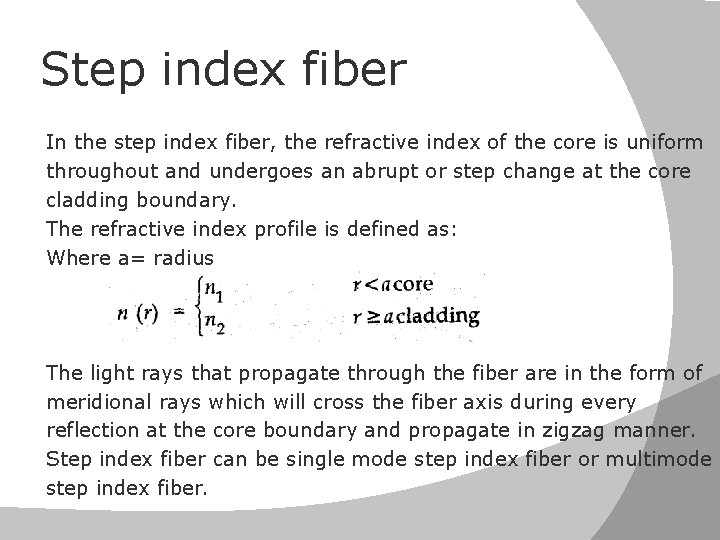
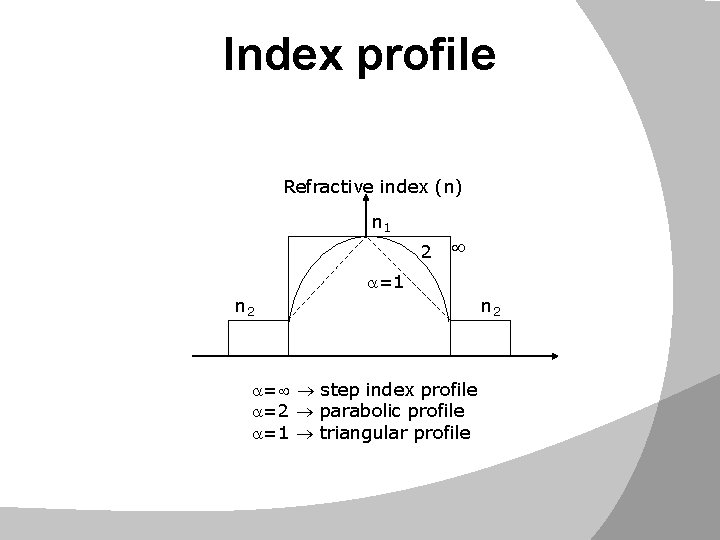
Step index fiber In the step index fiber, the refractive index of the core is uniform throughout and undergoes an abrupt or step change at the core cladding boundary. The refractive index profile is defined as: Where a= radius The light rays that propagate through the fiber are in the form of meridional rays which will cross the fiber axis during every reflection at the core boundary and propagate in zigzag manner. Step index fiber can be single mode step index fiber or multimode step index fiber. 9

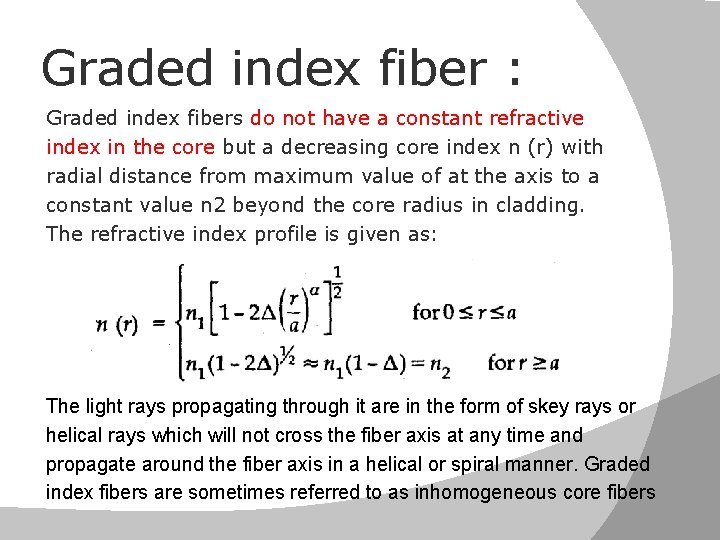
Graded index fiber : Graded index fibers do not have a constant refractive index in the core but a decreasing core index n (r) with radial distance from maximum value of at the axis to a constant value n 2 beyond the core radius in cladding. The refractive index profile is given as: The light rays propagating through it are in the form of skey rays or helical rays which will not cross the fiber axis at any time and propagate around the fiber axis in a helical or spiral manner. Graded index fibers are sometimes referred to as inhomogeneous core fibers 10

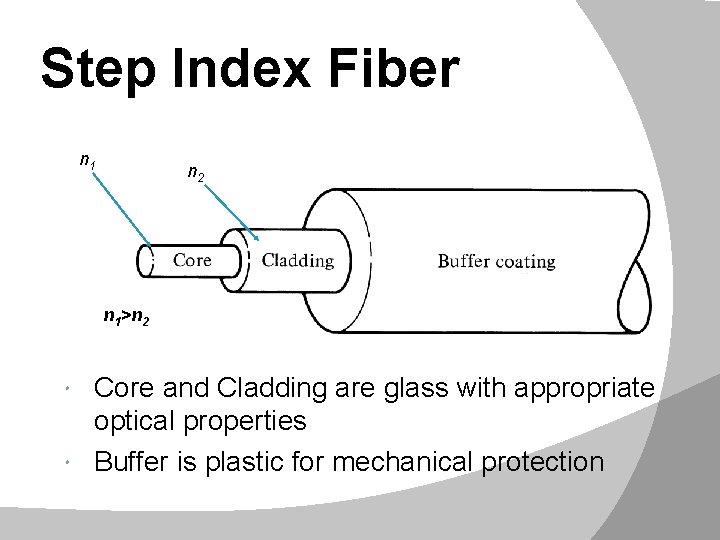
Step Index Fiber n 1 n 2 n 1>n 2 Core and Cladding are glass with appropriate optical properties Buffer is plastic for mechanical protection

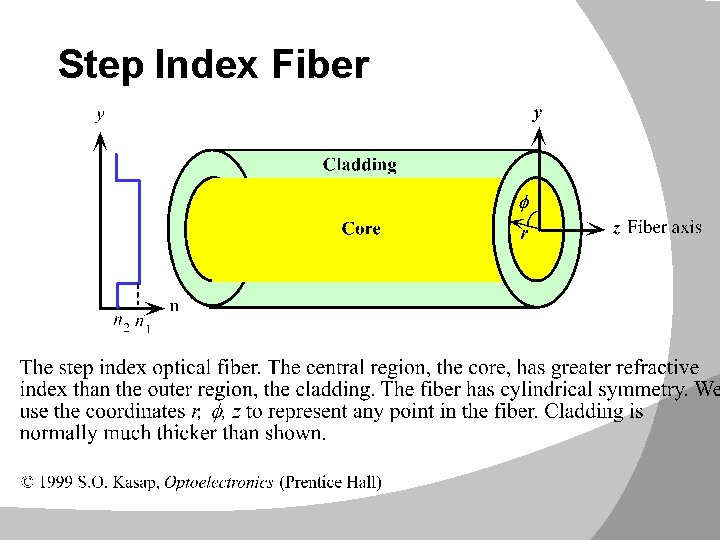
Step Index Fiber

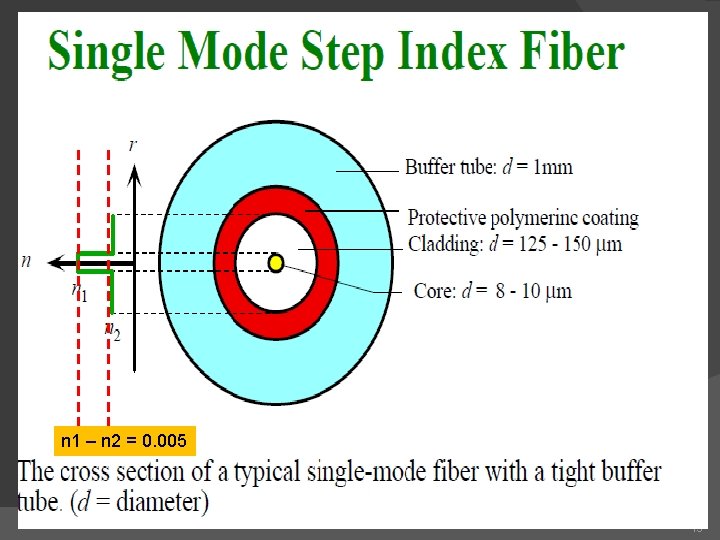
n 1 – n 2 = 0. 005 13

14

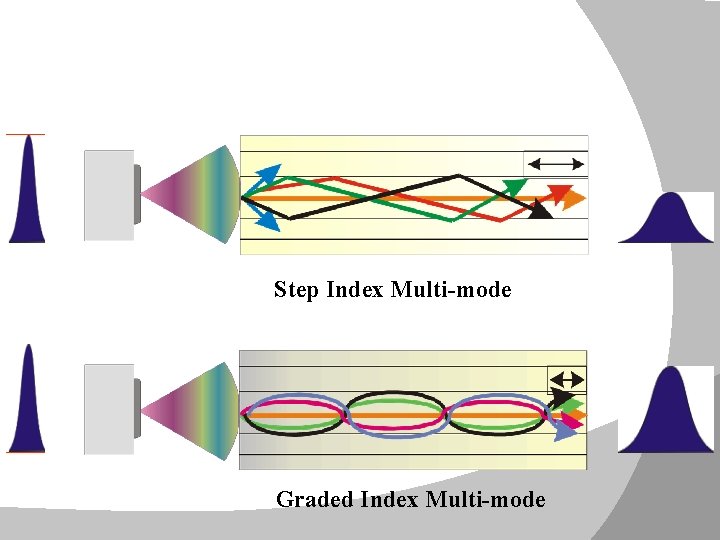
Step Index Multi-mode Graded Index Multi-mode

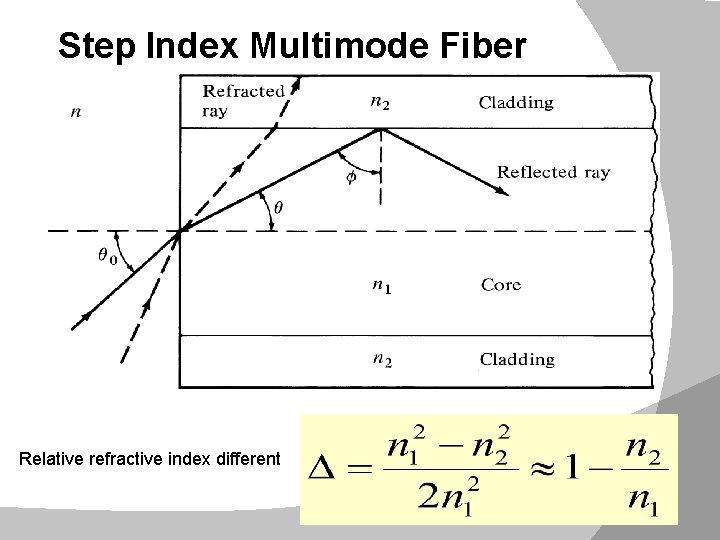
Step Index Multimode Fiber Relative refractive index different

Optical Fibre Waveguide 17

Index profile Refractive index (n) n 1 2 =1 n 2 = step index profile =2 parabolic profile =1 triangular profile 18

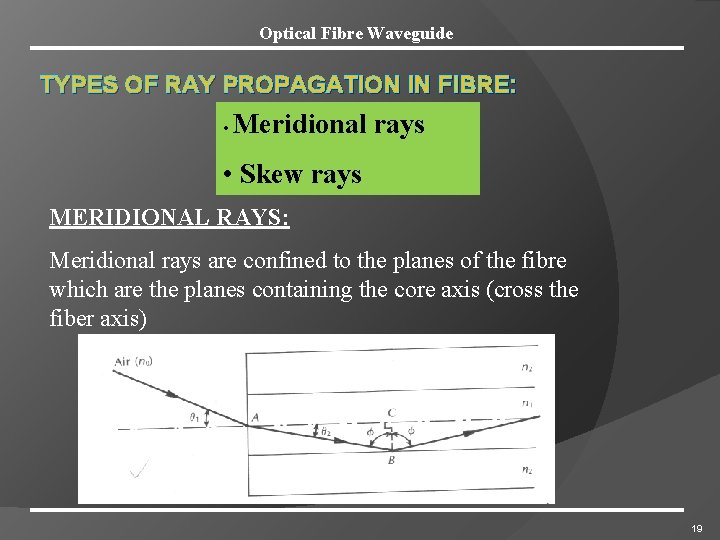
Optical Fibre Waveguide TYPES OF RAY PROPAGATION IN FIBRE: • Meridional rays • Skew rays MERIDIONAL RAYS: Meridional rays are confined to the planes of the fibre which are the planes containing the core axis (cross the fiber axis) 19

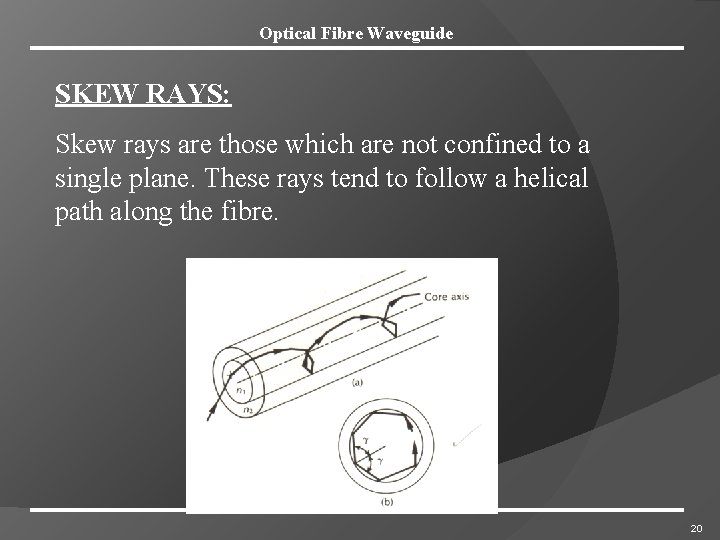
Optical Fibre Waveguide SKEW RAYS: Skew rays are those which are not confined to a single plane. These rays tend to follow a helical path along the fibre. 20

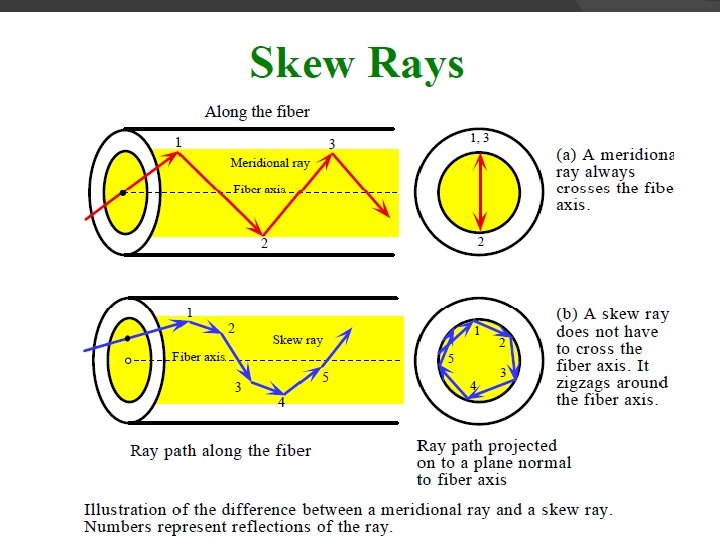
21

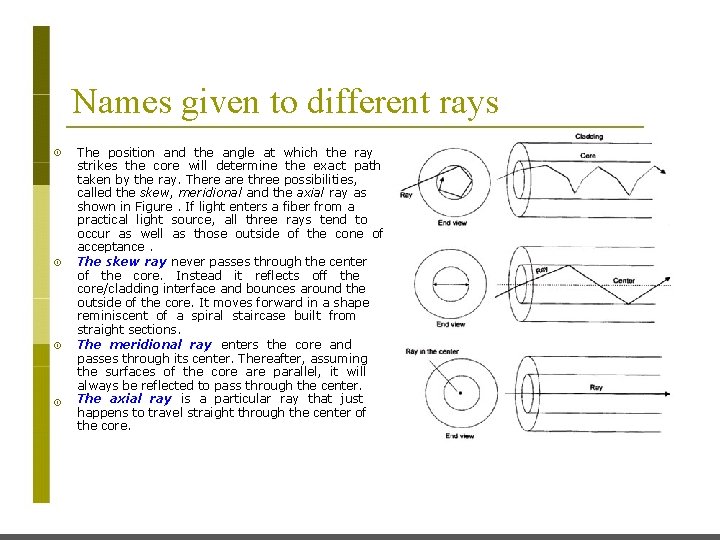
Names given to different rays The position and the angle at which the ray strikes the core will determine the exact path taken by the ray. There are three possibilities, called the skew, meridional and the axial ray as shown in Figure. If light enters a fiber from a practical light source, all three rays tend to occur as well as those outside of the cone of acceptance. The skew ray never passes through the center of the core. Instead it reflects off the core/cladding interface and bounces around the outside of the core. It moves forward in a shape reminiscent of a spiral staircase built from straight sections. The meridional ray enters the core and passes through its center. Thereafter, assuming the surfaces of the core are parallel, it will always be reflected to pass through the center. The axial ray is a particular ray that just happens to travel straight through the center of the core.

Optical Fibre Waveguide The cutoff condition A mode is referred to as being cut off when it is no longer bound to the core of the fiber. Since an optical fiber can support EM radiation if and only if the wavelength is much smaller than the fiber core diameter, the cutoff condition can be presented as where V is the normalized cutoff frequency or V-number, and a is the fiber radius. If k is the wave propagation constant along the transverse direction, then the normalized frequency parameter is given as u = relative reflective index diferential The normalized propagation constant is given as 23

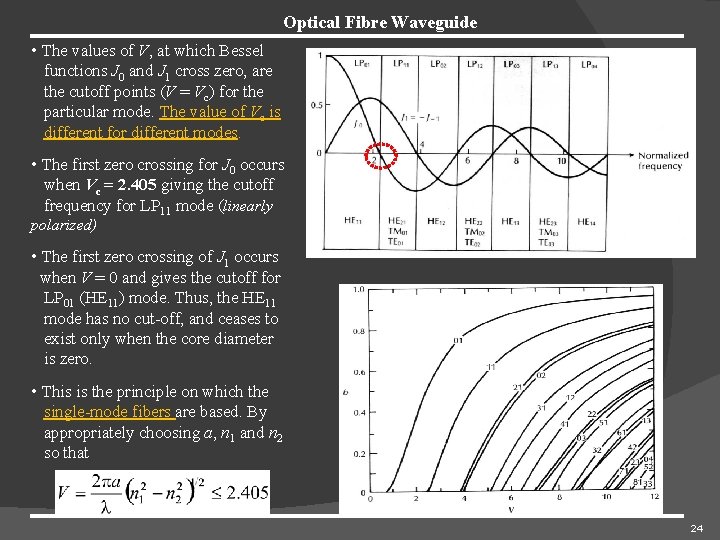
Optical Fibre Waveguide • The values of V, at which Bessel functions J 0 and J 1 cross zero, are the cutoff points (V = Vc) for the particular mode. The value of Vc is different for different modes. • The first zero crossing for J 0 occurs when Vc = 2. 405 giving the cutoff frequency for LP 11 mode (linearly polarized) • The first zero crossing of J 1 occurs when V = 0 and gives the cutoff for LP 01 (HE 11) mode. Thus, the HE 11 mode has no cut-off, and ceases to exist only when the core diameter is zero. • This is the principle on which the single-mode fibers are based. By appropriately choosing a, n 1 and n 2 so that 24

MODE What is mode? The propagation of light along a waveguide can be described in terms of a set of guided electromagnetic waves called the modes of the waveguide. 25

MODE • However, in optical fibers, the core-cladding boundary conditions lead to a coupling between the electric and the magnetic field components. This gives rise to hybrid modes, which makes optical waveguide analysis more complex than metallic waveguide analysis. • • Hybrid modes are the mixture of TE and TM modes that can be traveled through the optical fiber. • The hybrid modes are designated as HE or EH modes, depending on whether the transverse electric field (the E-field) or the transverse magnetic field (the H-field) is larger for that mode. • The two lowest order modes are designated by HE 11 and TE 01 modes, where the subscripts refer to possible modes of propagation of the optical field. 26

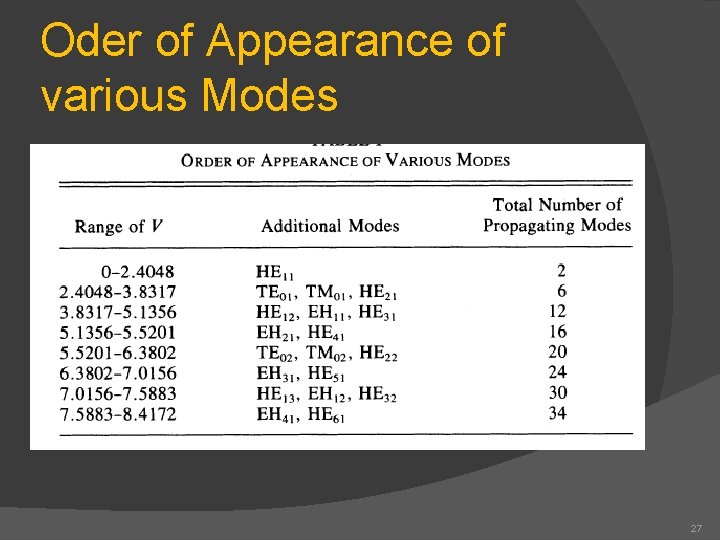
Oder of Appearance of various Modes 27

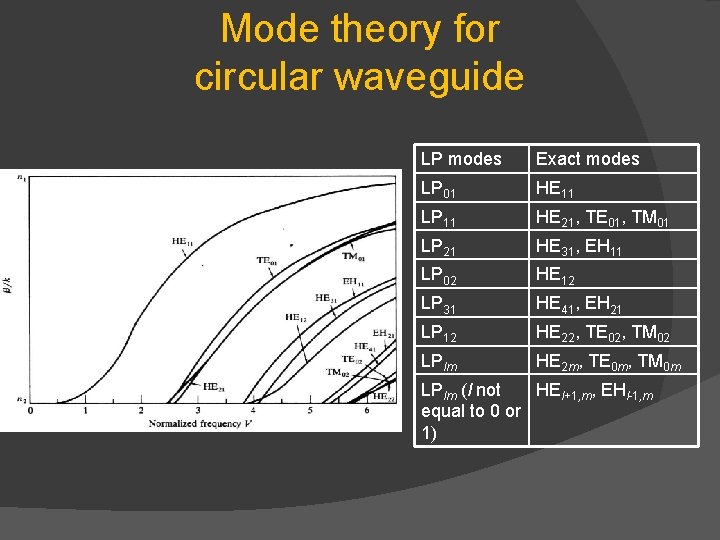
Mode theory for circular waveguide LP modes Exact modes LP 01 HE 11 LP 11 HE 21, TE 01, TM 01 LP 21 HE 31, EH 11 LP 02 HE 12 LP 31 HE 41, EH 21 LP 12 HE 22, TE 02, TM 02 LPlm HE 2 m, TE 0 m, TM 0 m LPlm (l not HEl+1, m, EHl-1, m equal to 0 or 1)

LP 02 LP 31

Optical Fibre Waveguide Number of modes M: Step-index fibers The parameter V can be related to the number of modes M in a multimode fiber when M is large. A ray incident on the end of a fiber will be accepted if it lies within the angle defined by the numerical aperture (NA) as NA = (n 12 – n 22)1/2. The practical value of is small; i. e. sin . The solid acceptance angle of the fiber is then = 2 = (n 12 – n 22). The number of modes per unit solid angle emanating from a laser or a waveguide is given by 2 A/ 2 where A is the area the mode leaving or entering, and in this case, is the core cross-section a 2. Total number of guided modes (mode volume) for a step index fiber is given by The factor 2 comes from the fact that the plane wave can have two polarization orientations. Graded-index Fibers The total number of guided modes supported by graded index fibres is given as: For = 2, the total modes supported is half the number supported by a step index fiber with the same V value. Graded index fibers accept less light compared to step index fibers with the same relative refractive index difference. 30

Important equations 31

Question A multimode step-index fibre has a relative RI difference of 1. 5% and a core RI of 1. 495. The number of modes propagating at a wavelength of 1330 nm is 1100. Estimate the diameter of the fibre core. 32

Solution 33

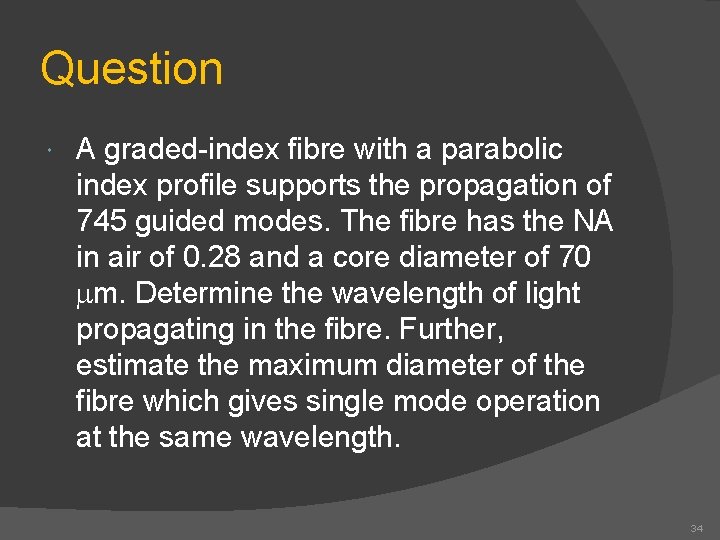
Question A graded-index fibre with a parabolic index profile supports the propagation of 745 guided modes. The fibre has the NA in air of 0. 28 and a core diameter of 70 m. Determine the wavelength of light propagating in the fibre. Further, estimate the maximum diameter of the fibre which gives single mode operation at the same wavelength. 34

Solution 35

Cutoff wavelength What is Cutoff wavelength? 36

Cutoff wavelength 37

Questionwavelength Determine Cutoff wavelength c for a fiber with a 3 -micrometer core diameter, a core index of refraction of 1. 545, and a cladding index of refraction of 1. 510. Vc =2. 405 38

Solutionwavelength Determine Cutoff wavelength c for a fiber with a 3 -micrometer core diameter, a core index of refraction of 1. 545, and a cladding index of refraction of 1. 510. 39

Questionwavelength Determine the cutoff wavelength for a step index fiber to exhibit single-mode. operation when the core refractive index and radius are 1. 46 and 4. 5 um, respectively, with the relative index differences being 0. 25%, Vc = 2. 405. 40

Solutionwavelength Determine the cutoff wavelength for a step index fiber to exhibit single-mode. operation when the core refractive index and radius are 1. 46 and 4. 5 um, respectively, with the relative index differences being 0. 25%, Vc = 2. 405. Answer 1214 nm 41

Optical Fibre Waveguide Mode Theory for Circular Waveguides • The propagation of light along a waveguide can be described in terms of a set of guided electromagnetic waves called the modes of the waveguide. • These guided modes are referred to as the bound or trapped modes of the waveguide. • Each guided mode is a pattern of electric and magnetic field distributions that is repeated along the fiber at equal intervals. • Only a certain discrete number of modes are capable of propagating along the guide. • These modes are those electromagnetic waves that satisfy the homogeneous wave equation in the fiber, and the boundary condition at the waveguide surfaces. 42

Optical Fibre Waveguide • To understand the detailed understanding of the optical power propagation mechanism in a fiber, it is necessary to solve Maxwell’s equations subject to the cylindrical boundary conditions at the interface between the core and the cladding of the fiber. • When solving Maxwell’s equations for hollow metallic waveguides, only transverse electric (TE) modes and transverse magnetic (TM) modes are found. • However, in optical fibers, the core-cladding boundary conditions lead to a coupling between the electric and the magnetic field components. This gives rise to hybrid modes, which makes optical waveguide analysis more complex than metallic waveguide analysis. • The hybrid modes are designated as HE or EH modes, depending on whether the transverse electric field (the E-field) or the transverse magnetic field (the H-field) is larger for that mode. • The two lowest order modes are designated by HE 11 and TE 01 modes, where the subscripts refer to possible modes of propagation of the optical field. 43

Optical Fibre Waveguide • Although theory of light propagation in optical fibers is well understood, a complete description of the guided and radiation modes is rather complex since it involves six-component hybrid electromagnetic fields that have very involved mathematical expressions. • A simplification of these expressions can be carried out, in practice, since fibers are usually constructed so that n 1 – n 2 1. • With this assumption, only four field components need to be considered, and their expressions become significantly simpler. • The field components are called linearly polarized (LP) modes, and are labeled as LPjm where j and m are integers designating mode solutions. • In this scheme, the fundamental LP 01 mode corresponds to an HE 11 mode. • Although the analysis required for even these simplifications is still fairly complex, this is key to understand the principles of optical fiber operation. 44

Optical Fibre Waveguide • Maxwell’s equations finally show that, in addition to supporting a finite number of guided modes, the optical fiber waveguide has an infinite continuum of radiation modes that are not trapped in the core and guided by the fiber, but are still solutions of the same boundary-value problem. • The radiation field basically results from the optical power that is outside the fiber acceptance angle being refracted out of the core. • Because of the finite radius of the cladding, some of this radiation gets trapped in the cladding, thereby causing cladding modes to appear. • As the core and cladding modes propagate along the fiber, mode coupling occurs between the cladding modes and the higher order core modes. • This coupling occurs because the electric fields of the guided core modes are not completely confined to the core, but extend partially into the cladding, and likewise for the cladding modes. • A diffusion of power back and forth between the core and the cladding modes thus occurs, which generally results in a loss of power from the core modes. • In practice, the cladding modes will be suppressed by a lossy coating which covers the fiber or they will scatter out of the fiber after traveling a certain distance because of the roughness on the cladding surface. 45

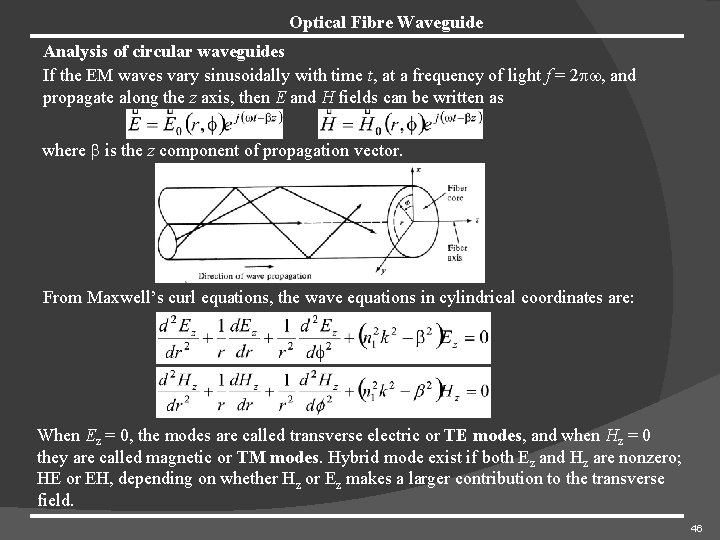
Optical Fibre Waveguide Analysis of circular waveguides If the EM waves vary sinusoidally with time t, at a frequency of light f = 2 , and propagate along the z axis, then E and H fields can be written as where is the z component of propagation vector. From Maxwell’s curl equations, the wave equations in cylindrical coordinates are: When Ez = 0, the modes are called transverse electric or TE modes, and when Hz = 0 they are called magnetic or TM modes. Hybrid mode exist if both Ez and Hz are nonzero; HE or EH, depending on whether Hz or Ez makes a larger contribution to the transverse field. 46

Optical Fibre Waveguide • In addition to the bound and refracted modes, a third category of modes, called leaky modes, is present in optical fibers. • These leaky modes are only partially confined to the core region, and attenuate by continuously radiating their power out of the core as they propagate along the fiber. This power radiation out of the waveguide results from a quantum mechanical phenomenon known as the tunneling effect. • It is essentially based on the upper and lower bounds that the boundary conditions for the solutions of Maxwell’s equations impose on the propagation factor . A mode remains guided as long as satisfies the condition n 2 k < < n 1 k where n 1 and n 2 are the refractive indices of the core and the cladding, respectively, and k = 2 /. • The boundary condition between truly guided modes and leaky modes is defined by the cutoff condition = n 2 k. As soon as becomes smaller than n 2 k, power leaks out of the core into the cladding region. • Leaky modes can carry significant amount of optical power in short fibers. Most of these modes disappear after a few centimeters, but a few have sufficiently low losses to persist in fiber lengths of a kilometer. 47

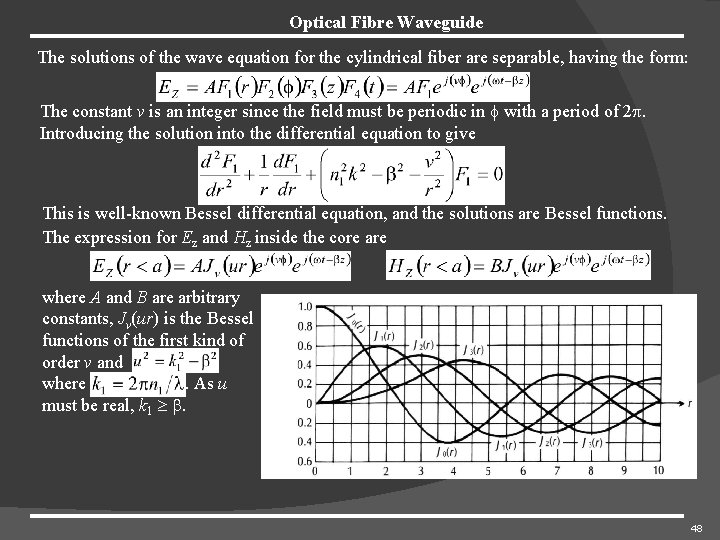
Optical Fibre Waveguide The solutions of the wave equation for the cylindrical fiber are separable, having the form: The constant v is an integer since the field must be periodic in with a period of 2. Introducing the solution into the differential equation to give This is well-known Bessel differential equation, and the solutions are Bessel functions. The expression for Ez and Hz inside the core are where A and B are arbitrary constants, Jv(ur) is the Bessel functions of the first kind of order v and where. As u must be real, k 1 . 48

Optical Fibre Waveguide Outside of the core, where C and D are arbitrary constants, Kv(wr) are the modified Bessel functions of the second kind, and where. . Since Kv(wr) must go to zero as r , so w > 0, which implies that k 2. The permissible range of for bound solutions is 49