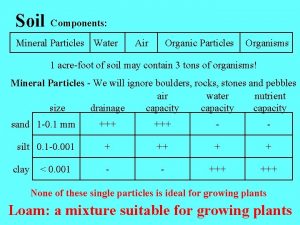
Chapter 14 Systems of Identical Particles Identical particles





- Slides: 49

Chapter 14 Systems of Identical Particles

Identical particles 14. A. 1 • In reality the postulates of quantum mechanics formulated by us previously are not sufficient when we are dealing with systems consisting of many identical particles • Identical particles are particles with exactly the same intrinsic properties (mass, spin, charge, etc. ) • Example: all electrons are identical; an electron is not identical to a proton or to a positron; etc. • If a system consists of two or more identical particles there should be no change in its properties or its evolution if the roles of any two particles are exchanged

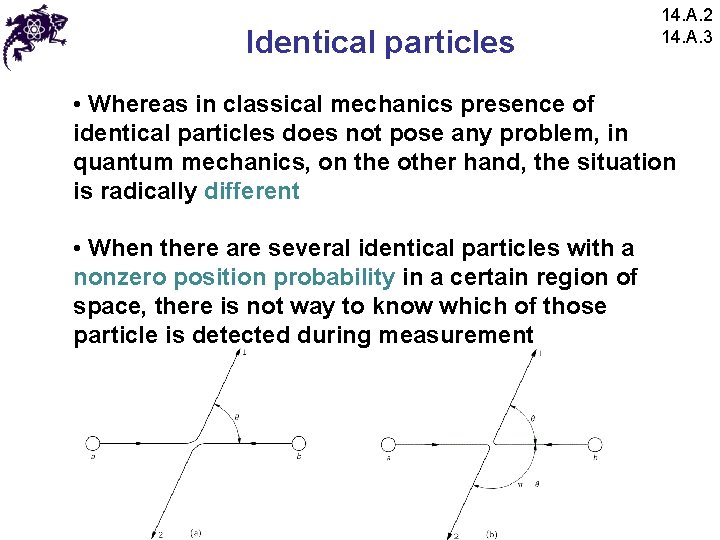
Identical particles 14. A. 2 14. A. 3 • Whereas in classical mechanics presence of identical particles does not pose any problem, in quantum mechanics, on the other hand, the situation is radically different • When there are several identical particles with a nonzero position probability in a certain region of space, there is not way to know which of those particle is detected during measurement

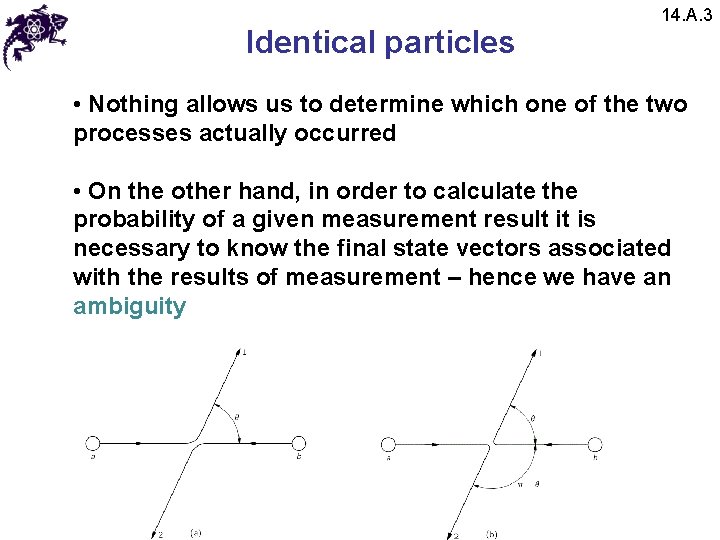
Identical particles 14. A. 3 • Nothing allows us to determine which one of the two processes actually occurred • On the other hand, in order to calculate the probability of a given measurement result it is necessary to know the final state vectors associated with the results of measurement – hence we have an ambiguity

Exchange degeneracy 14. A. 3 • Let us consider a system consisting of two identical particles with spin ½ and concentrate on the spin degrees of freedom • After performing a measurement on this system we learn that the z spin component of one particle is ћ/2, and that of the other one is –ћ/2 • Labelling the particles 1 and 2, denoting their spins S 1 and S 2, we introduce an orthonormal basis of the spin state space: • The physical state considered here can be described by either of these two orthogonal kets:

Exchange degeneracy 14. A. 3 • These two kets span a 2 D subspace containing normalized vectors of the following form: • Any ket belonging to this subspace can represent the observed state, which is the essence of the exchange degeneracy • On the other hand application of postulates of quantum mechanics can lead to physical predictions that will depend on a specific ket chosen • The exchange degeneracy thus has to be removed to avoid this ambiguity

Exchange degeneracy 14. A. 3 • Let us consider another example: 3 identical particles • If we consider them separately, they will be described by three separate state spaces: • The state space of the 3 -particle system is: • Now, let us consider a set of observables comprising a CSCO in E(1): • This set of observables will also comprise a CSCO in E(2) and E(3):

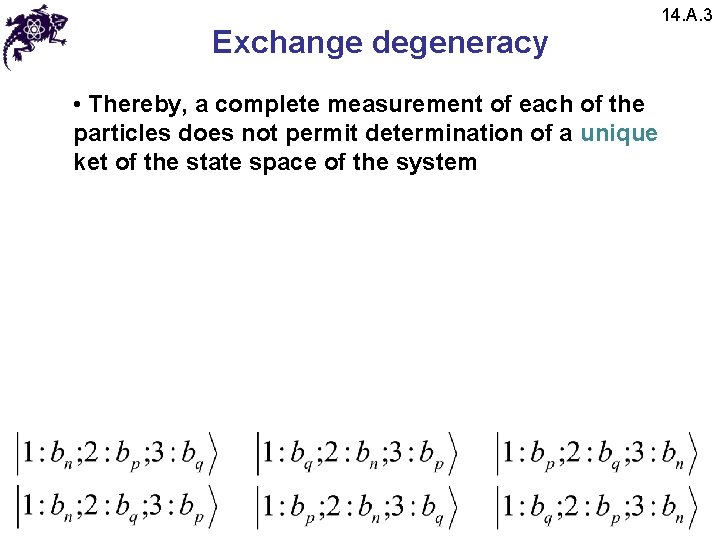
Exchange degeneracy 14. A. 3 • B(1), B(2), B(3) will have the same spectrum: • Using this let us construct an orthonormal basis in E: • Since the particles are identical, we cannot measure B(1), B(2), B(3) but we can measure B for each of the three particles, e. g. bn, bp and bq. • The state of the system after this measurement can be represented by any one of the kets of the subspace in E spanned by the following six kets:

Exchange degeneracy • Thereby, a complete measurement of each of the particles does not permit determination of a unique ket of the state space of the system 14. A. 3

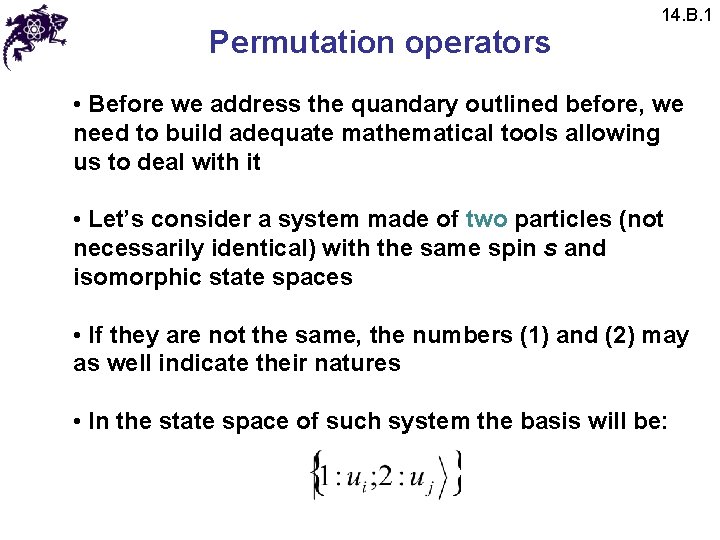
Permutation operators 14. B. 1 • Before we address the quandary outlined before, we need to build adequate mathematical tools allowing us to deal with it • Let’s consider a system made of two particles (not necessarily identical) with the same spin s and isomorphic state spaces • If they are not the same, the numbers (1) and (2) may as well indicate their natures • In the state space of such system the basis will be:

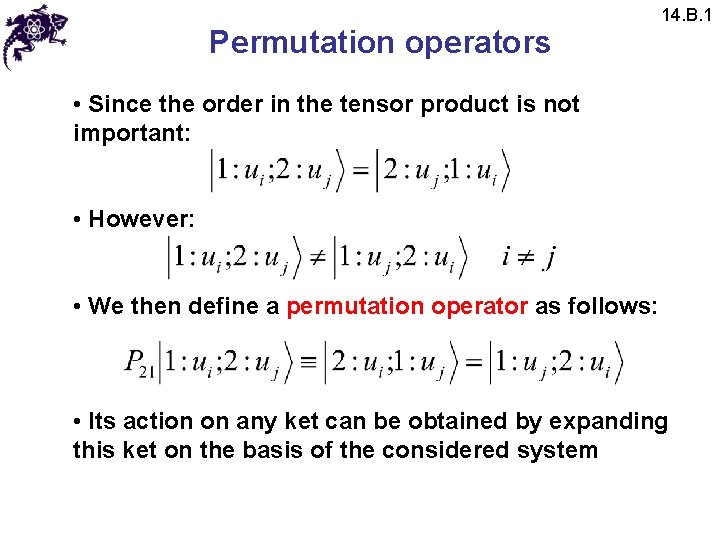
Permutation operators 14. B. 1 • Since the order in the tensor product is not important: • However: • We then define a permutation operator as follows: • Its action on any ket can be obtained by expanding this ket on the basis of the considered system

Permutation operators • Since: • The permutation operator P 21 is its own inverse: • Also, it is Hermitian and unitary: 14. B. 1

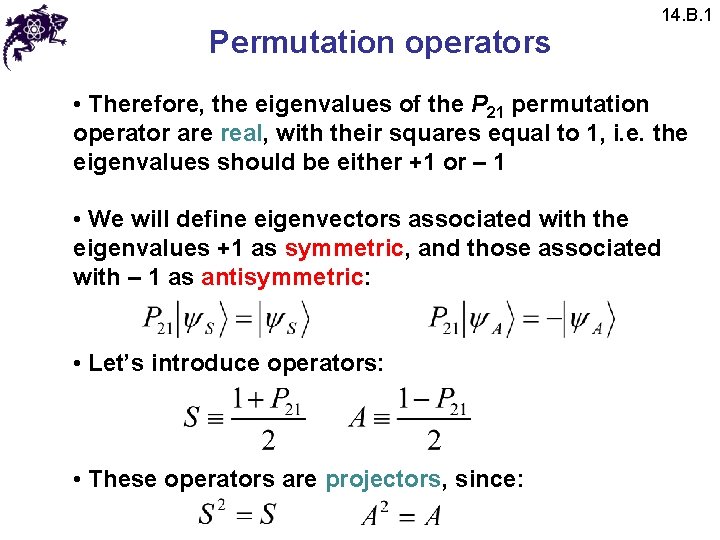
Permutation operators 14. B. 1 • Therefore, the eigenvalues of the P 21 permutation operator are real, with their squares equal to 1, i. e. the eigenvalues should be either +1 or – 1 • We will define eigenvectors associated with the eigenvalues +1 as symmetric, and those associated with – 1 as antisymmetric: • Let’s introduce operators: • These operators are projectors, since:

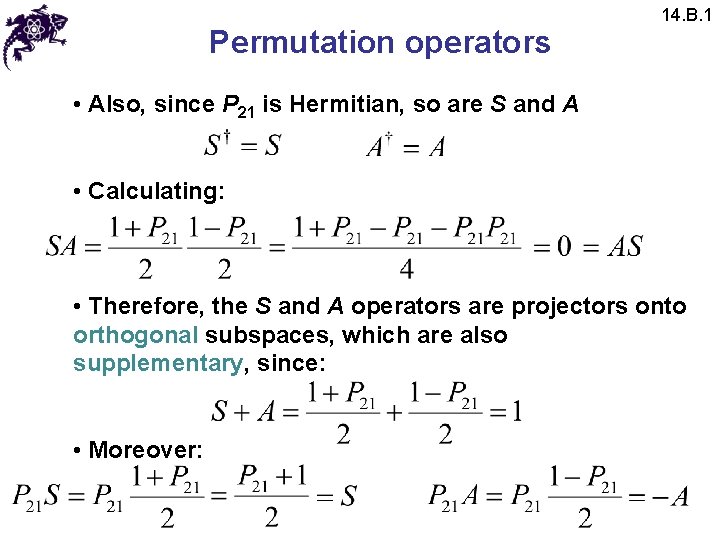
Permutation operators 14. B. 1 • Also, since P 21 is Hermitian, so are S and A • Calculating: • Therefore, the S and A operators are projectors onto orthogonal subspaces, which are also supplementary, since: • Moreover:

Permutation operators • Therefore: • Because of this, S is called a symmetrizer and A – antisymmetrizer • Similarly: 14. B. 1

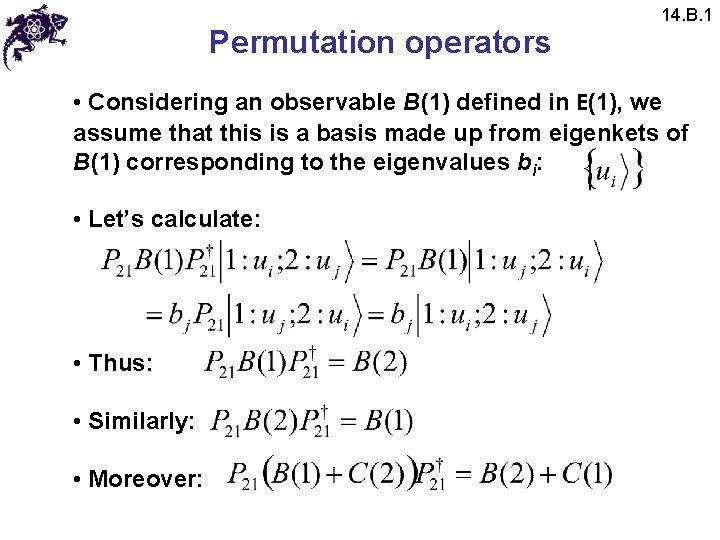
Permutation operators 14. B. 1 • Considering an observable B(1) defined in E(1), we assume that this is a basis made up from eigenkets of B(1) corresponding to the eigenvalues bi: • Let’s calculate: • Thus: • Similarly: • Moreover:

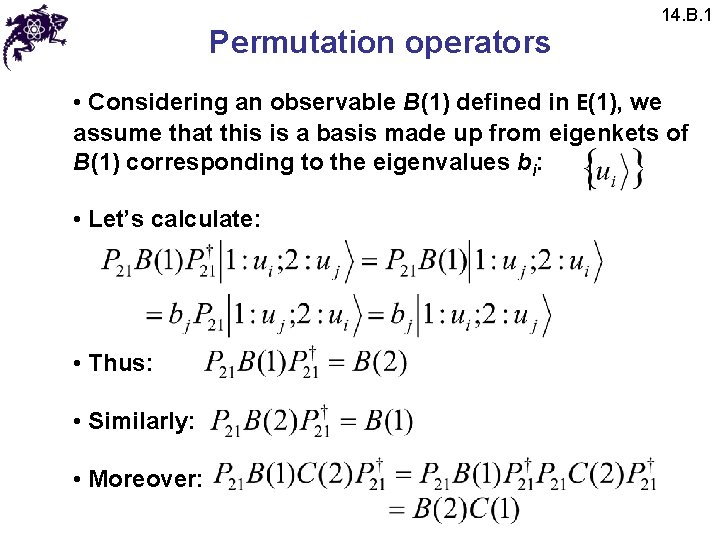
Permutation operators 14. B. 1 • Considering an observable B(1) defined in E(1), we assume that this is a basis made up from eigenkets of B(1) corresponding to the eigenvalues bi: • Let’s calculate: • Thus: • Similarly: • Moreover:

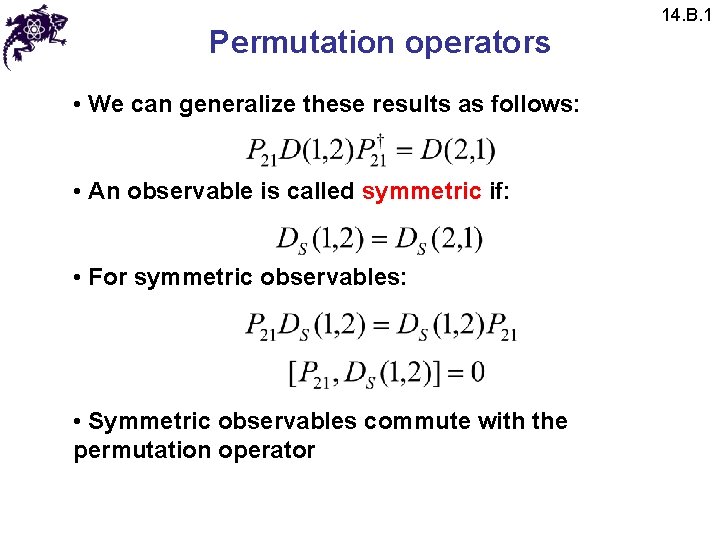
Permutation operators • We can generalize these results as follows: • An observable is called symmetric if: • For symmetric observables: • Symmetric observables commute with the permutation operator 14. B. 1

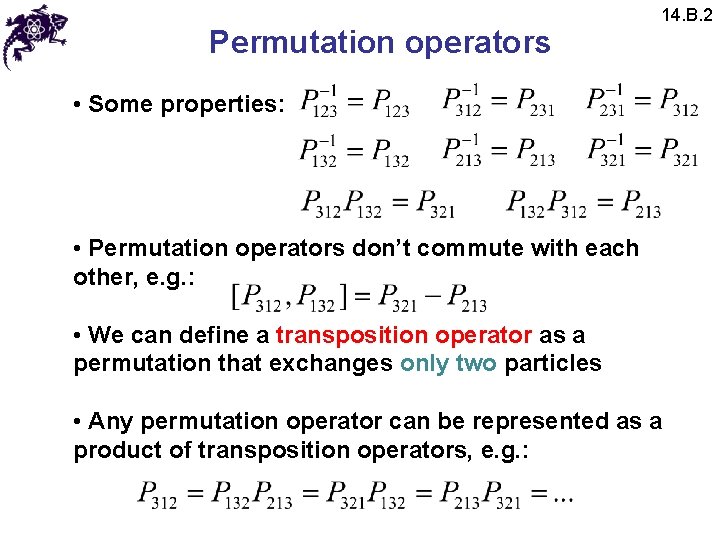
Permutation operators 14. B. 2 • Let’s consider a system made of three particles (not necessarily identical) with the same spin s and isomorphic state spaces • In the state space of such system the basis will be: • We then define a permutation operator as follows: • For example: • Obviously:

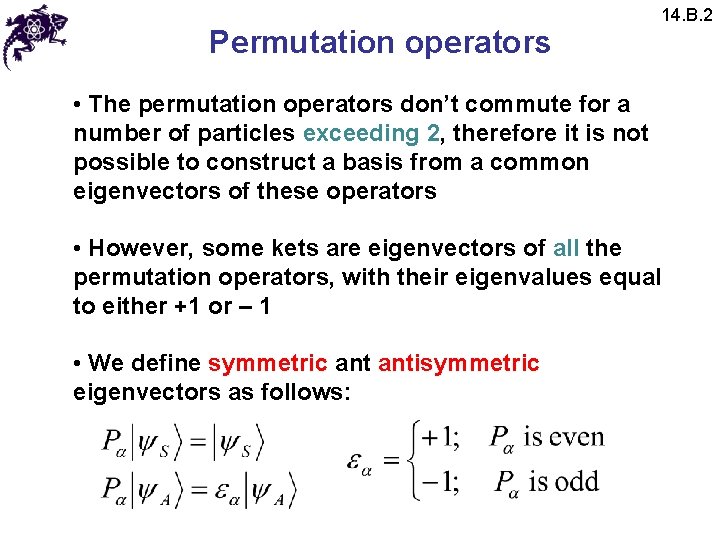
Permutation operators 14. B. 2 • Some properties: • Permutation operators don’t commute with each other, e. g. : • We can define a transposition operator as a permutation that exchanges only two particles • Any permutation operator can be represented as a product of transposition operators, e. g. :

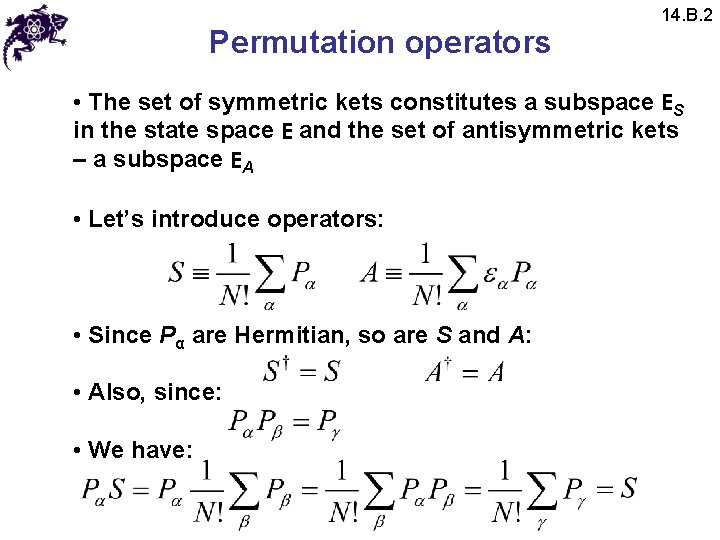
Permutation operators 14. B. 2 • The permutation operators don’t commute for a number of particles exceeding 2, therefore it is not possible to construct a basis from a common eigenvectors of these operators • However, some kets are eigenvectors of all the permutation operators, with their eigenvalues equal to either +1 or – 1 • We define symmetric antisymmetric eigenvectors as follows:

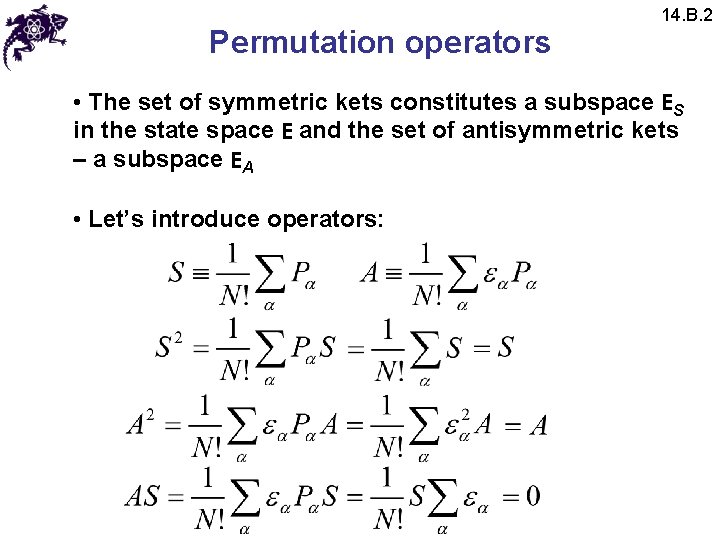
Permutation operators 14. B. 2 • The set of symmetric kets constitutes a subspace ES in the state space E and the set of antisymmetric kets – a subspace EA • Let’s introduce operators: • Since Pα are Hermitian, so are S and A: • Also, since: • We have:

Permutation operators 14. B. 2 • The set of symmetric kets constitutes a subspace ES in the state space E and the set of antisymmetric kets – a subspace EA • Let’s introduce operators: • Since Pα are Hermitian, so are S and A: • Also, since: • We have:

Permutation operators 14. B. 2 • The set of symmetric kets constitutes a subspace ES in the state space E and the set of antisymmetric kets – a subspace EA • Let’s introduce operators:

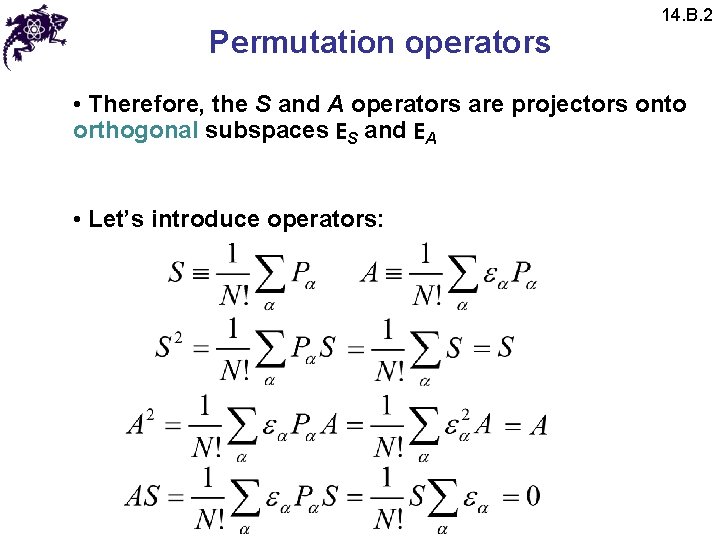
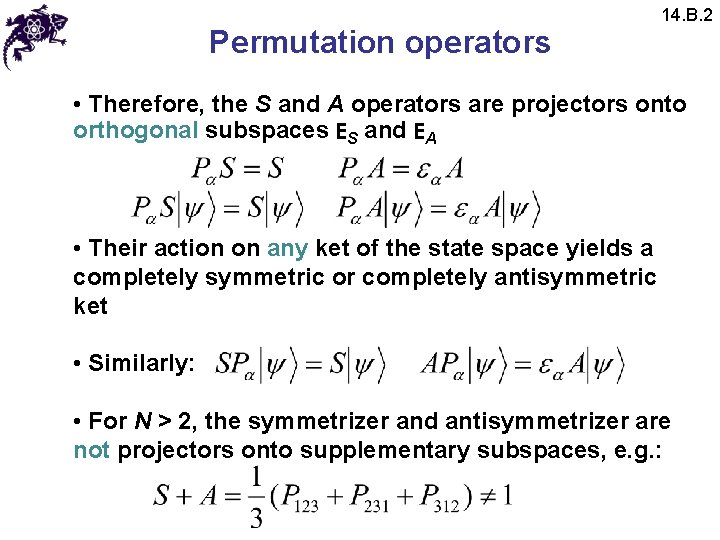
Permutation operators 14. B. 2 • Therefore, the S and A operators are projectors onto orthogonal subspaces ES and EA • Let’s introduce operators:

Permutation operators 14. B. 2 • Therefore, the S and A operators are projectors onto orthogonal subspaces ES and EA • Their action on any ket of the state space yields a completely symmetric or completely antisymmetric ket • Similarly: • For N > 2, the symmetrizer and antisymmetrizer are not projectors onto supplementary subspaces, e. g. :

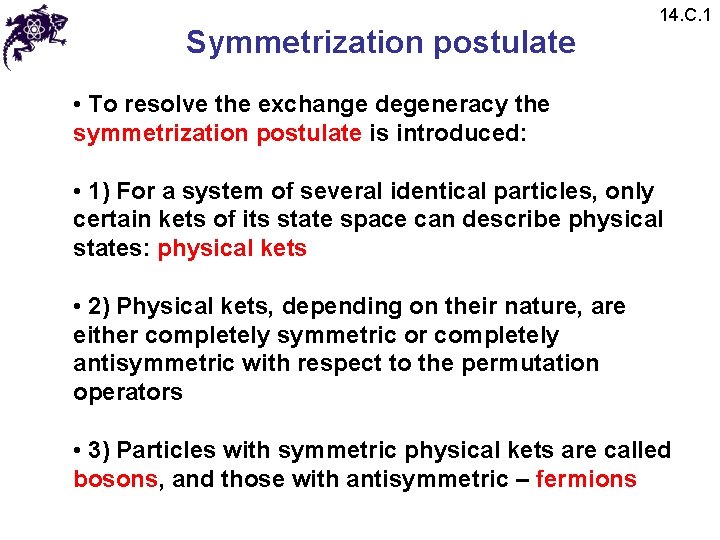
Symmetrization postulate 14. C. 1 • To resolve the exchange degeneracy the symmetrization postulate is introduced: • 1) For a system of several identical particles, only certain kets of its state space can describe physical states: physical kets • 2) Physical kets, depending on their nature, are either completely symmetric or completely antisymmetric with respect to the permutation operators • 3) Particles with symmetric physical kets are called bosons, and those with antisymmetric – fermions

Symmetrization postulate Satyendra Nath Bose (1894 – 1974) 14. C. 1 Enrico Fermi (1901– 1954) • 3) Particles with symmetric physical kets are called bosons, and those with antisymmetric – fermions

Symmetrization postulate 14. C. 1 • The symmetrization postulate limits the state space for a system of identical particles • This state space is not the tensor product of individual state spaces of the constituent particles • It is either ES or EA, depending on whether the identical particles are bosons or fermions • Current empirical rule allocates particles with integral spins to bosons (photons, mesons, etc. ) and with half-integral spins – to fermions (electrons, protons, neutrons, etc. )

Removal of exchange degeneracy 14. C. 2 • Let us recall: • Projections onto ES or EA of various kets of the state space are all collinear, producing corresponding physical kets (i. e. kets associated with a given physical state): • Thus the ambiguity of the exchange degeneracy is removed • If for a given set of states a projection is zero, then such physical state is impossible

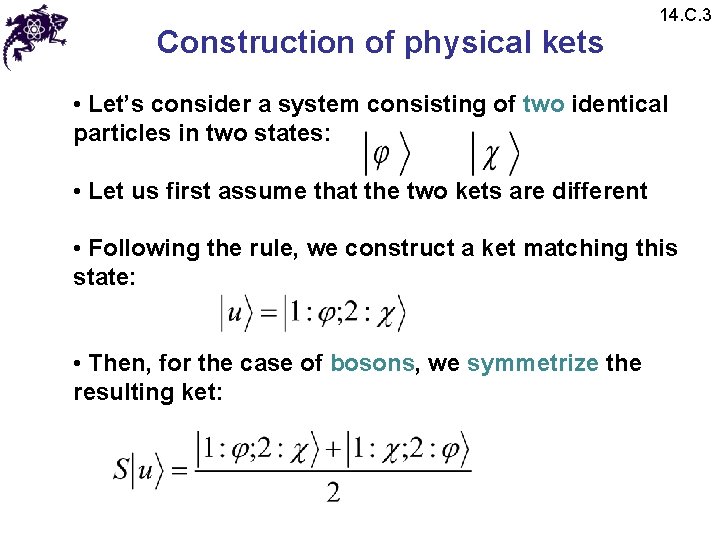
Construction of physical kets 14. C. 3 • Based on the preceding discussion one can formulate the rule for constructing a unique physical ket corresponding to a given physical state of a system of N identical particles: • 1) Number the particles arbitrarily and construct a ket corresponding to a given physical state and to the numbers given to the particles • 2) Apply S or A to this ket, depending on whether the particles are bosons or fermions • 3) Normalize the resulting ket

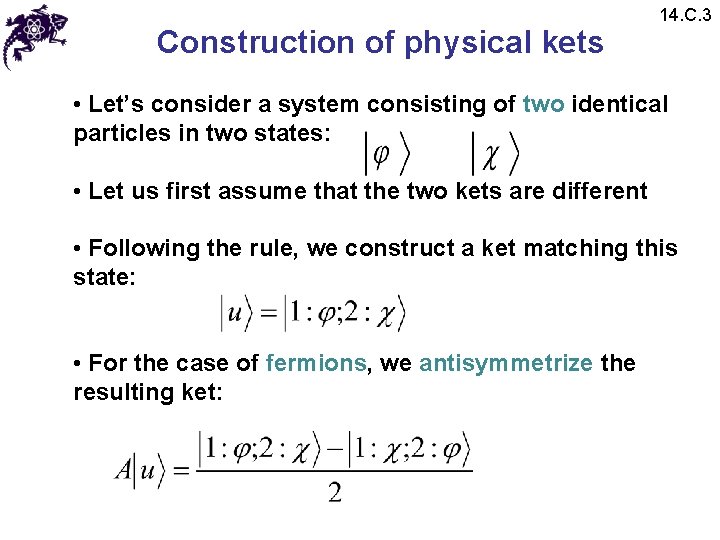
Construction of physical kets 14. C. 3 • Let’s consider a system consisting of two identical particles in two states: • Let us first assume that the two kets are different • Following the rule, we construct a ket matching this state: • Then, for the case of bosons, we symmetrize the resulting ket:

Construction of physical kets 14. C. 3 • Let’s consider a system consisting of two identical particles in two states: • Let us first assume that the two kets are different • Following the rule, we construct a ket matching this state: • For the case of fermions, we antisymmetrize the resulting ket:

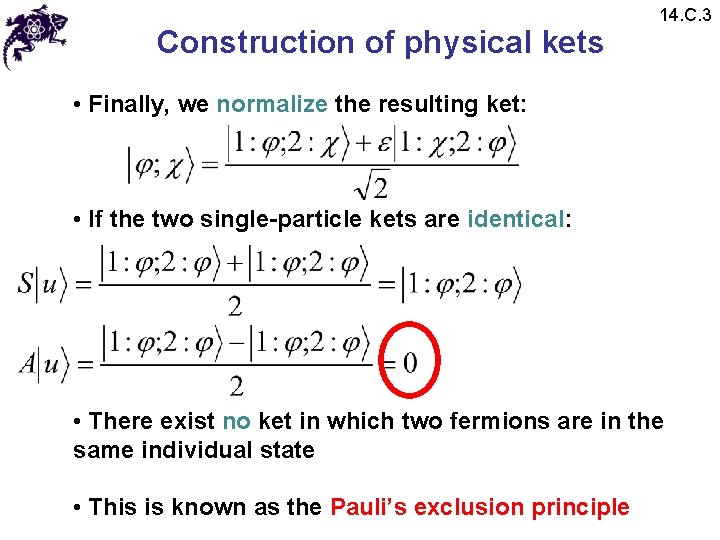
Construction of physical kets 14. C. 3 • Finally, we normalize the resulting ket: • If the two single-particle kets are identical: • There exist no ket in which two fermions are in the same individual state • This is known as the Pauli’s exclusion principle

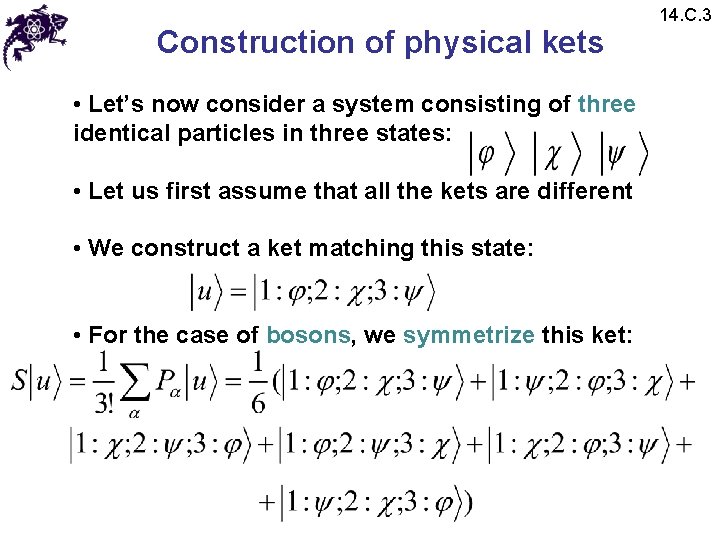
Construction of physical kets • Let’s now consider a system consisting of three identical particles in three states: • Let us first assume that all the kets are different • We construct a ket matching this state: • For the case of bosons, we symmetrize this ket: 14. C. 3

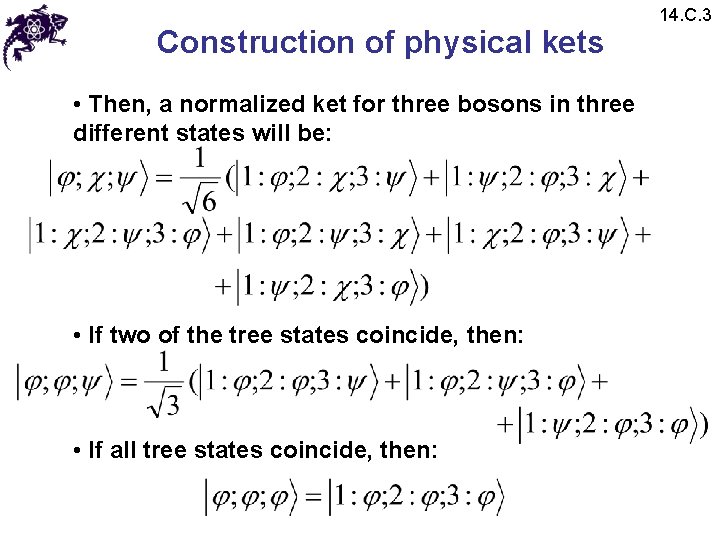
Construction of physical kets • Then, a normalized ket for three bosons in three different states will be: • If two of the tree states coincide, then: • If all tree states coincide, then: 14. C. 3

Construction of physical kets 14. C. 3 • For the case of fermions, we antisymmetrize this ket: John Clarke Slater (1900 – 1976) • This representation is called the Slater determinant • If two states in the Slater determinant coincide, it then contains two identical columns and thus is equal to zero – Pauli’s exclusion principle

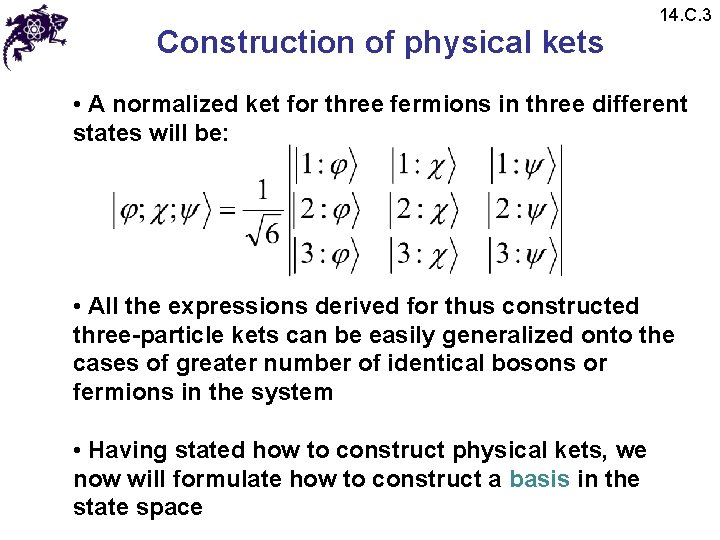
Construction of physical kets 14. C. 3 • A normalized ket for three fermions in three different states will be: • All the expressions derived for thus constructed three-particle kets can be easily generalized onto the cases of greater number of identical bosons or fermions in the system • Having stated how to construct physical kets, we now will formulate how to construct a basis in the state space

Construction of a basis in the state space 14. C. 3 • Let us consider a system of N identical particles, each of which represented by a basis in its own state space: • Now in the tensor product space E we put together a basis: • However, the physical state space of the system is not E but either of the two subspaces, ES or EA • Thus, to construct a basis in the physical state space one has to apply S or A to the kets of the basis in E:

Construction of a basis in the state space 14. C. 3 • By doing so we obtain a set of kets spanning ES or E A: • If such ket belongs, e. g. , to ES then: • And: • However, the kets in this sum are not independent • In order to construct a basis consisting of independent vectors a change of notation has to be made

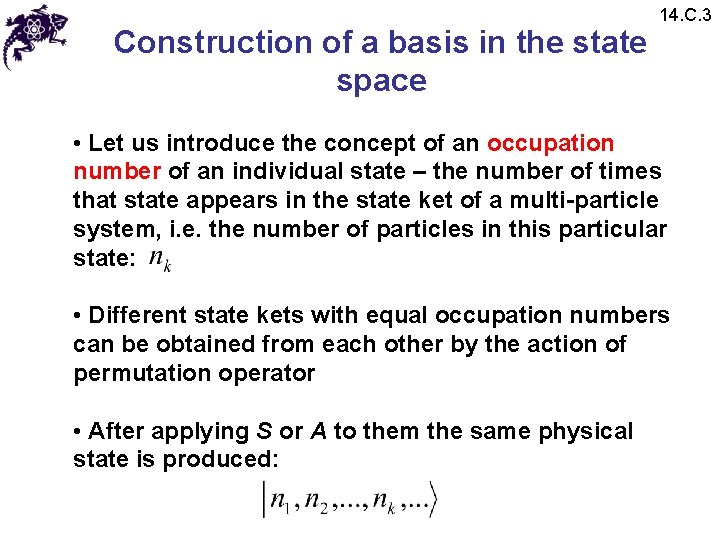
Construction of a basis in the state space 14. C. 3 • Let us introduce the concept of an occupation number of an individual state – the number of times that state appears in the state ket of a multi-particle system, i. e. the number of particles in this particular state: • Different state kets with equal occupation numbers can be obtained from each other by the action of permutation operator • After applying S or A to them the same physical state is produced:

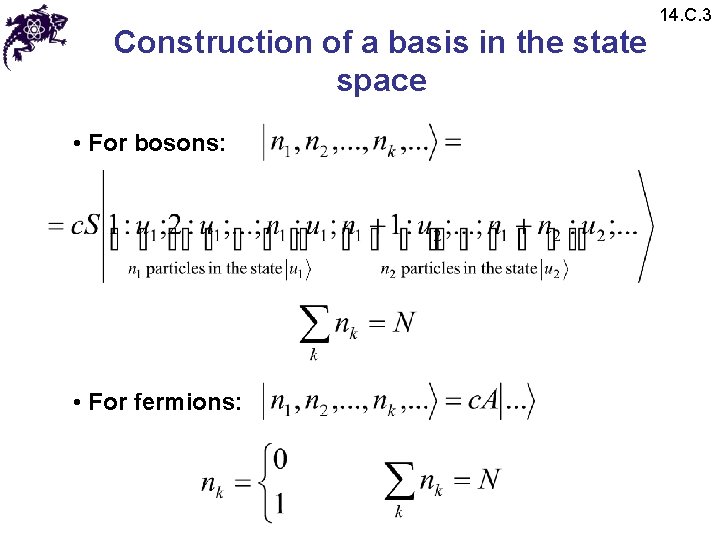
Construction of a basis in the state space • For bosons: • For fermions: 14. C. 3

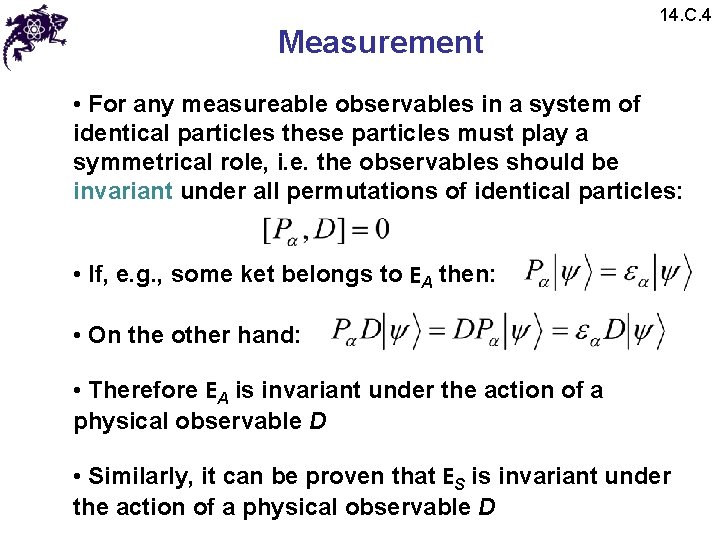
Measurement 14. C. 4 • For any measureable observables in a system of identical particles these particles must play a symmetrical role, i. e. the observables should be invariant under all permutations of identical particles: • If, e. g. , some ket belongs to EA then: • On the other hand: • Therefore EA is invariant under the action of a physical observable D • Similarly, it can be proven that ES is invariant under the action of a physical observable D

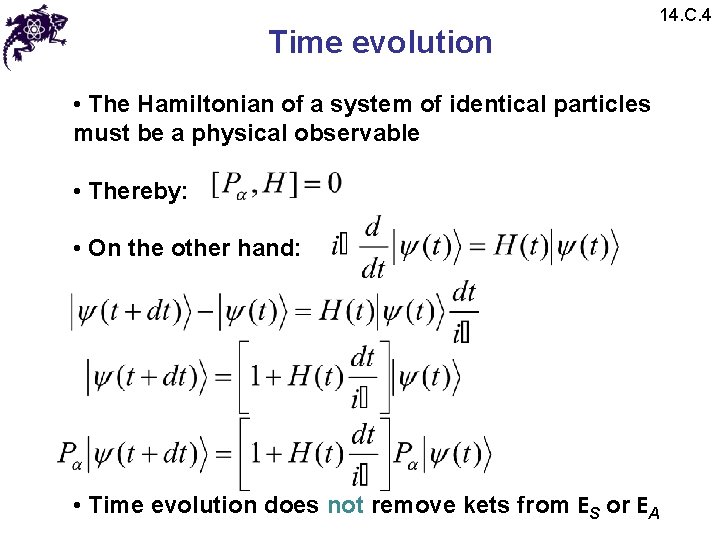
Time evolution 14. C. 4 • The Hamiltonian of a system of identical particles must be a physical observable • Thereby: • On the other hand: • Time evolution does not remove kets from ES or EA

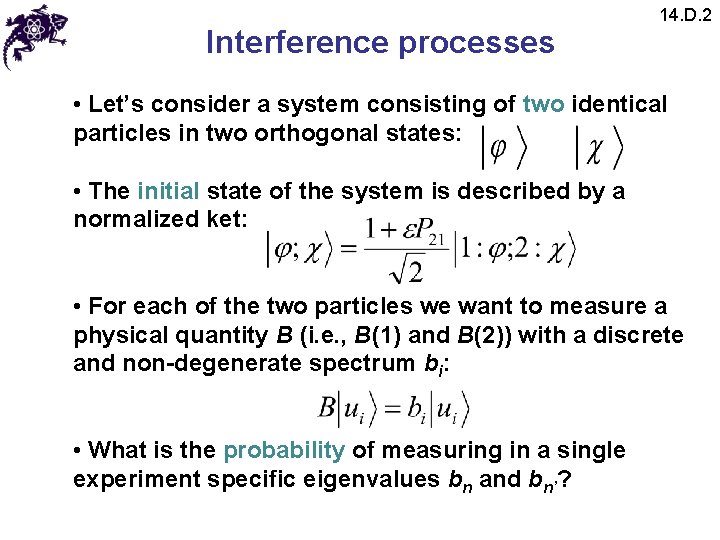
Interference processes 14. D. 2 • Let’s consider a system consisting of two identical particles in two orthogonal states: • The initial state of the system is described by a normalized ket: • For each of the two particles we want to measure a physical quantity B (i. e. , B(1) and B(2)) with a discrete and non-degenerate spectrum bi: • What is the probability of measuring in a single experiment specific eigenvalues bn and bn’?

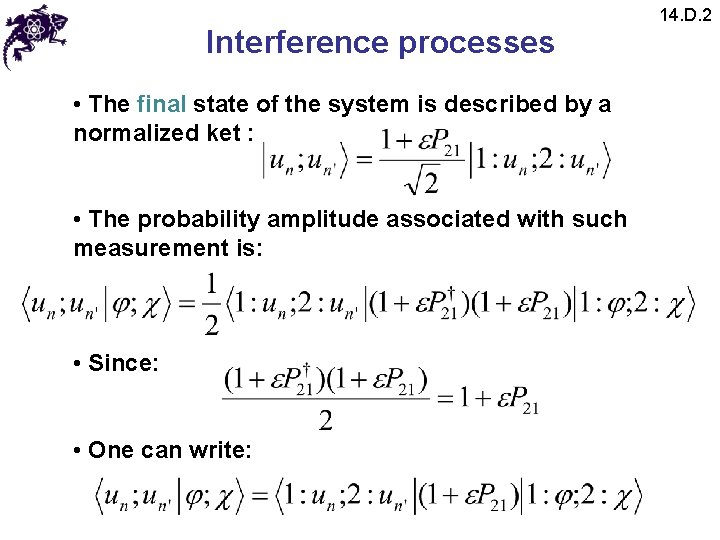
Interference processes • The final state of the system is described by a normalized ket : • The probability amplitude associated with such measurement is: • Since: • One can write: 14. D. 2

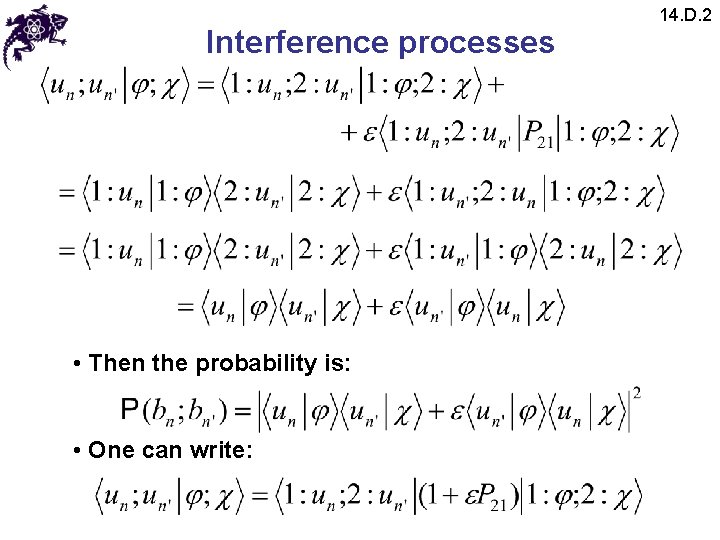
Interference processes • Then the probability is: • One can write: 14. D. 2

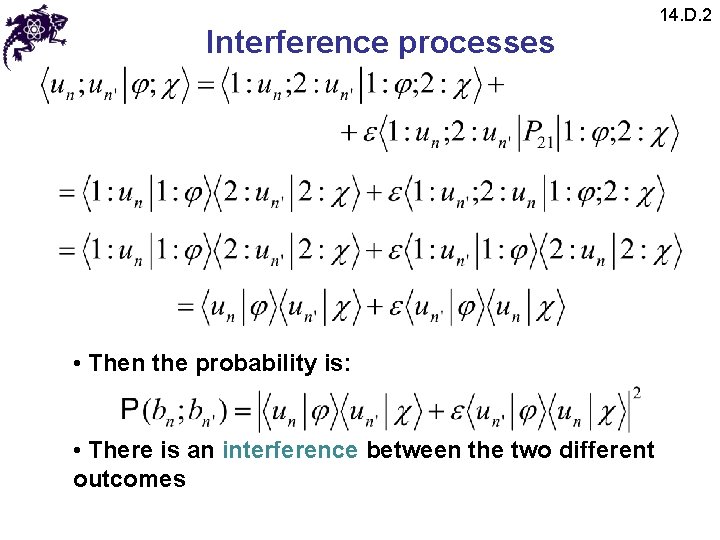
Interference processes • Then the probability is: • There is an interference between the two different outcomes 14. D. 2

Interference processes • Then the probability is: • There is an interference between the two different outcomes 14. D. 2
 Identical by descent vs identical by state
Identical by descent vs identical by state Identical particles
Identical particles Systems of particles
Systems of particles Ions charged particles in solution
Ions charged particles in solution Decision support systems and intelligent systems
Decision support systems and intelligent systems Principles of complex systems for systems engineering
Principles of complex systems for systems engineering Embedded systems vs cyber physical systems
Embedded systems vs cyber physical systems Elegant systems
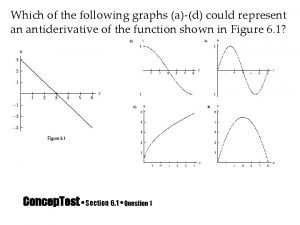
Elegant systems Which of the following graphs are identical?
Which of the following graphs are identical? Pop thats their warmup
Pop thats their warmup A solid object with two identical ends and flat sides
A solid object with two identical ends and flat sides Rita and holly are identical twins
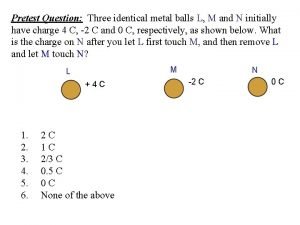
Rita and holly are identical twins You have 5 identical metal balls
You have 5 identical metal balls Identical vs fraternal twins
Identical vs fraternal twins Abab rhyme scheme example
Abab rhyme scheme example Fraternal twins pedigree chart
Fraternal twins pedigree chart Pedigree chart identical twins
Pedigree chart identical twins What is nurture in psychology
What is nurture in psychology Free verse vs prose
Free verse vs prose Twins with different hair color
Twins with different hair color Charles's law
Charles's law Rita and holly are identical twins
Rita and holly are identical twins It takes ten identical pieces to form a circular track
It takes ten identical pieces to form a circular track Similar sire similar scion
Similar sire similar scion Refomulator
Refomulator Identical system
Identical system You are given two carts a and b
You are given two carts a and b Csi handwriting analysis
Csi handwriting analysis Distribution of n identical objects
Distribution of n identical objects Identical objects into distinct bins
Identical objects into distinct bins A pattern is made from four identical squares
A pattern is made from four identical squares Rita and holly are identical twins
Rita and holly are identical twins Verb form of identical
Verb form of identical Identical resistors
Identical resistors Abcdefgh is a cuboid angle edh 64
Abcdefgh is a cuboid angle edh 64 Identical product
Identical product Shannon is making identical balloon arrangements
Shannon is making identical balloon arrangements The fan blade is slowing down. what are the signs of
The fan blade is slowing down. what are the signs of Rita and holly are identical twins
Rita and holly are identical twins A solid object with two identical ends and flat sides
A solid object with two identical ends and flat sides In the indicator diagram ta tb tc td
In the indicator diagram ta tb tc td Groups of restaurants identical in market
Groups of restaurants identical in market A moving freight car runs into an identical
A moving freight car runs into an identical Make your own pedigree
Make your own pedigree Which process produces only identical offspring
Which process produces only identical offspring Momentum practice problems
Momentum practice problems When a pair of identical lamps are connected in parallel
When a pair of identical lamps are connected in parallel The rapid manufacture of large numbers of identical objects
The rapid manufacture of large numbers of identical objects The creation of genetically identical offspring
The creation of genetically identical offspring Plasma particles
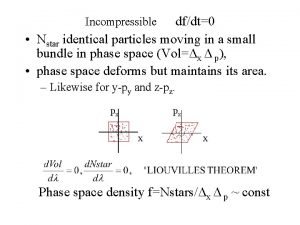
Plasma particles