Chapter 11 States of Matter Liquids and Solids




- Slides: 21

Chapter 11 States of Matter; Liquids and Solids 8– 1 John A. Schreifels Chemistry 211 1

Overview • Changes of State – Phase transitions – Phase Diagrams • Liquid State – Properties of Liquids; Surface tension and viscosity – Intermolecular forces; explaining liquid properties • Solid State – – – Classification of Solids by Type of Attraction between Units Crystalline solids; crystal lattices and unit cells Structures of some crystalline solids Calculations Involving Unit-Cell Dimensions Determining the Crystal Structure by X-ray Diffraction John A. Schreifels Chemistry 211 8– 2 2

Comparison of Gases, Liquids and Solids – Gases are compressible fluids. Their molecules are widely separated. – Liquids are relatively incompressible fluids. Their molecules are more tightly packed. – Solids are nearly incompressible and rigid. Their molecules or ions are in close contact and do not move. 8– 3 Figure 11. 2 States of Matter John A. Schreifels Chemistry 211 3

Phase Transitions • Melting: change of a solid to a liquid. H 2 O(s) H 2 O(l) • Freezing: change a liquid to a solid. H 2 O(l) H 2 O(s) • Vaporization: change of a solid or liquid to a gas. Change of solid to vapor often called sublimation. H 2 O(l) H 2 O(g) or H 2 O(s) H 2 O(g) • Condensation: change of a gas to a H 2 O(g) H 2 O(l) liquid or solid. Change of a gas to a or H 2 O(g) H 2 O(s) solid often called deposition. 8– 4 John A. Schreifels Chemistry 211 4

Vapor Pressure • In a sealed container, some of a liquid evaporates to establish a pressure in the vapor phase. • Vapor pressure: partial pressure of the vapor over the liquid measured at equilibrium and at some temperature. • Dynamic equilibrium 8– 5 John A. Schreifels Chemistry 211 5

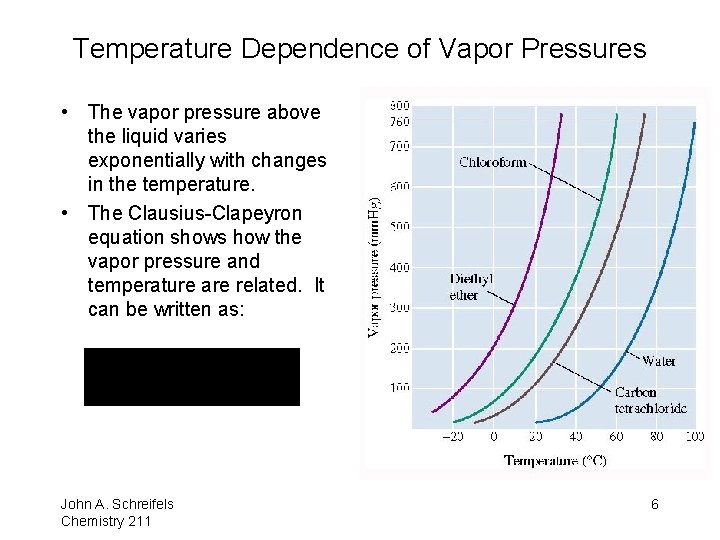
Temperature Dependence of Vapor Pressures • The vapor pressure above the liquid varies exponentially with changes in the temperature. • The Clausius-Clapeyron equation shows how the vapor pressure and temperature are related. It can be written as: 8– 6 John A. Schreifels Chemistry 211 6

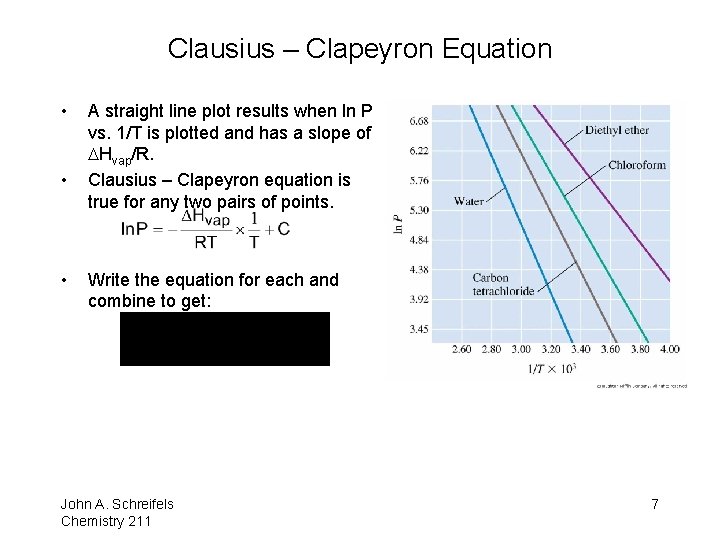
Clausius – Clapeyron Equation • • • A straight line plot results when ln P vs. 1/T is plotted and has a slope of Hvap/R. Clausius – Clapeyron equation is true for any two pairs of points. Write the equation for each and combine to get: 8– 7 John A. Schreifels Chemistry 211 7

Using the Clausius – Clapeyron Equation • Boiling point the temperature at which the vapor pressure of a liquid is equal to the pressure of the external atmosphere. • Normal boiling point the temperature at which the vapor pressure of a liquid is equal to atmospheric pressure (1 atm). E. g. Determine normal boiling point of chloroform if its heat of vaporization is 31. 4 k. J/mol and it has a vapor pressure of 190. 0 mm. Hg at 25. 0°C. E. g. 2. The normal boiling point of benzene is 80. 1°C; at 26. 1°C it has a vapor pressure of 100. 0 mm. Hg. What is the heat of vaporization? John A. Schreifels Chemistry 211 8 8– 8

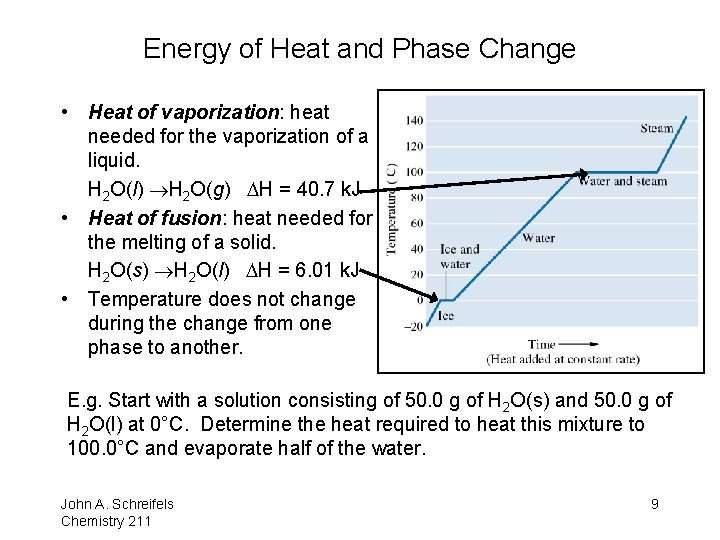
Energy of Heat and Phase Change • Heat of vaporization: heat needed for the vaporization of a liquid. H 2 O(l) H 2 O(g) H = 40. 7 k. J • Heat of fusion: heat needed for the melting of a solid. H 2 O(s) H 2 O(l) H = 6. 01 k. J • Temperature does not change during the change from one phase to another. E. g. Start with a solution consisting of 50. 0 g of H 2 O(s) and 50. 0 g of H 2 O(l) at 0°C. Determine the heat required to heat this mixture to 8– 9 100. 0°C and evaporate half of the water. John A. Schreifels Chemistry 211 9

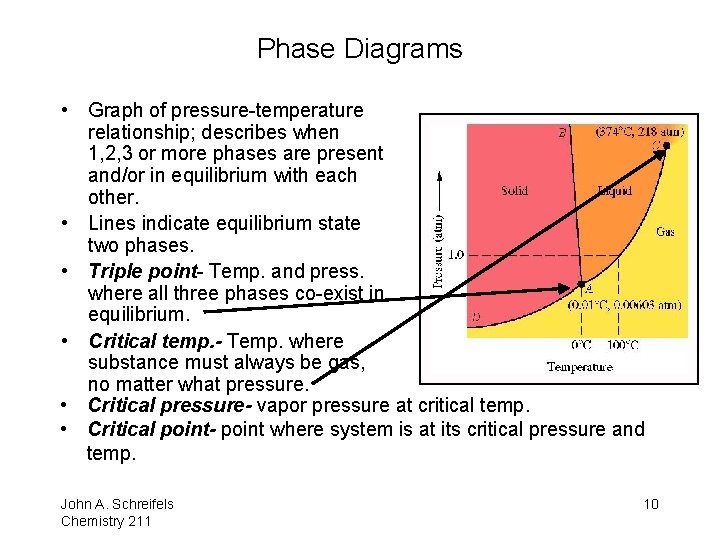
Phase Diagrams • Graph of pressure-temperature relationship; describes when 1, 2, 3 or more phases are present and/or in equilibrium with each other. • Lines indicate equilibrium state two phases. • Triple point- Temp. and press. where all three phases co-exist in equilibrium. • Critical temp. - Temp. where substance must always be gas, no matter what pressure. • Critical pressure- vapor pressure at critical temp. • Critical point- point where system is at its critical pressure and temp. John A. Schreifels Chemistry 211 8– 10 10

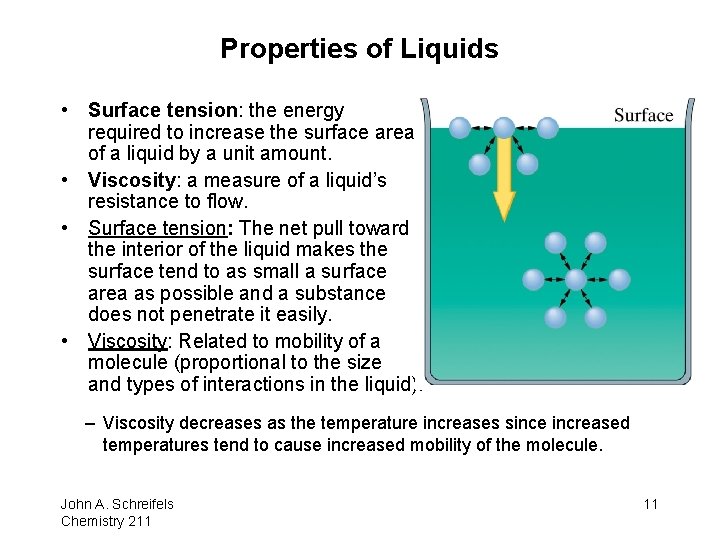
Properties of Liquids • Surface tension: the energy required to increase the surface area of a liquid by a unit amount. • Viscosity: a measure of a liquid’s resistance to flow. • Surface tension: The net pull toward the interior of the liquid makes the surface tend to as small a surface area as possible and a substance does not penetrate it easily. • Viscosity: Related to mobility of a molecule (proportional to the size and types of interactions in the liquid). – Viscosity decreases as the temperature increases since increased temperatures tend to cause increased mobility of the molecule. John A. Schreifels Chemistry 211 8– 11 11

Intermolecular Forces • Intermolecular forces: attractions and repulsions between molecules that hold them together. • Intermolecular forces (van der Waals forces) hold molecules together in liquid and solid phases. – Ion-dipole force: interaction between an ion and partial charges in a polar molecule. – Dipole-dipole force: attractive force between polar molecules with positive end of one molecule is aligned with negative side of other. – London dispersion Forces: interactions between instantaneously formed electric dipoles on neighboring polar or nonpolar molecules. – Polarizability: ease with which electron cloud of some substance can be distorted by presence of some electric field (such as another dipolar substance). Related to size of atom or molecule. Small atoms and molecules less easily polarized. 8– 12 John A. Schreifels Chemistry 211 12

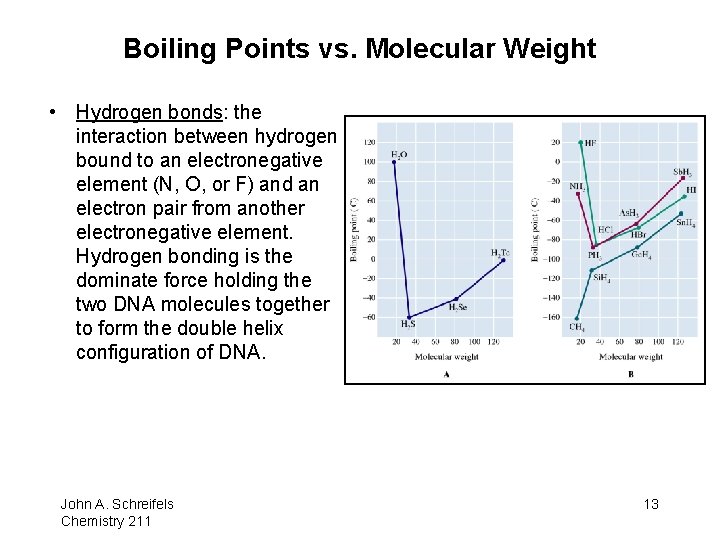
Boiling Points vs. Molecular Weight • Hydrogen bonds: the interaction between hydrogen bound to an electronegative element (N, O, or F) and an electron pair from another electronegative element. Hydrogen bonding is the dominate force holding the two DNA molecules together to form the double helix configuration of DNA. 8– 13 John A. Schreifels Chemistry 211 13

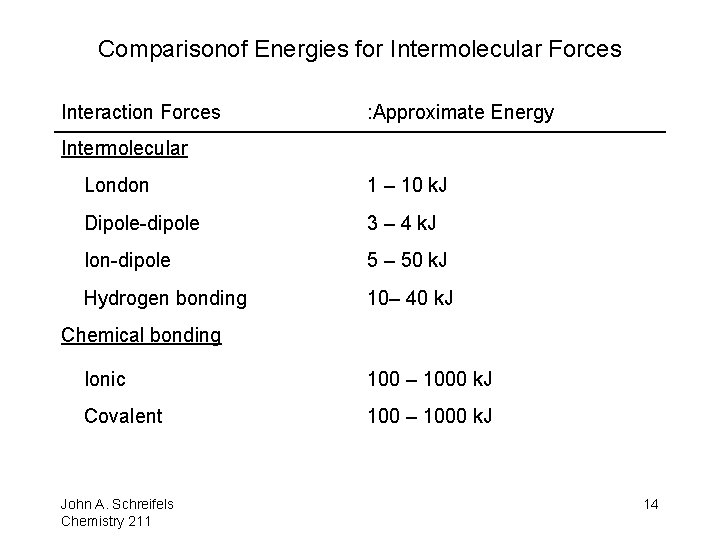
Comparisonof Energies for Intermolecular Forces Interaction Forces : Approximate Energy Intermolecular London 1 – 10 k. J Dipole-dipole 3 – 4 k. J Ion-dipole 5 – 50 k. J Hydrogen bonding 10– 40 k. J Chemical bonding Ionic 100 – 1000 k. J Covalent 100 – 1000 k. J 8– 14 John A. Schreifels Chemistry 211 14

Structure of Solids • Types of solids: – Crystalline – a well defined arrangement of atoms; this arrangement is often seen on a macroscopic level. • Ionic solids – ionic bonds hold the solids in a regular three dimensional arrangement. • Molecular solid – solids like ice that are held together by intermolecular forces. • Covalent network – a solid consists of atoms held together in large networks or chains by covalent networks. • Metallic – similar to covalent network except with metals. Provides high conductivity. – Amorphous – atoms are randomly arranged. No order exists 8– 15 in the solid. John A. Schreifels Chemistry 211 15

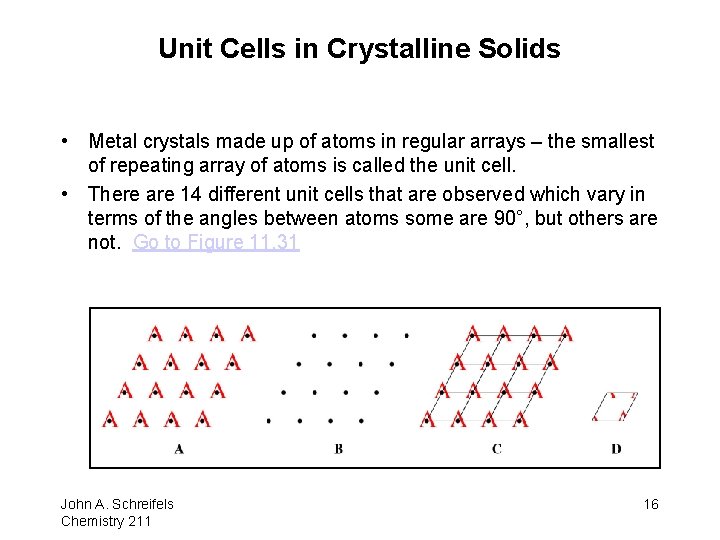
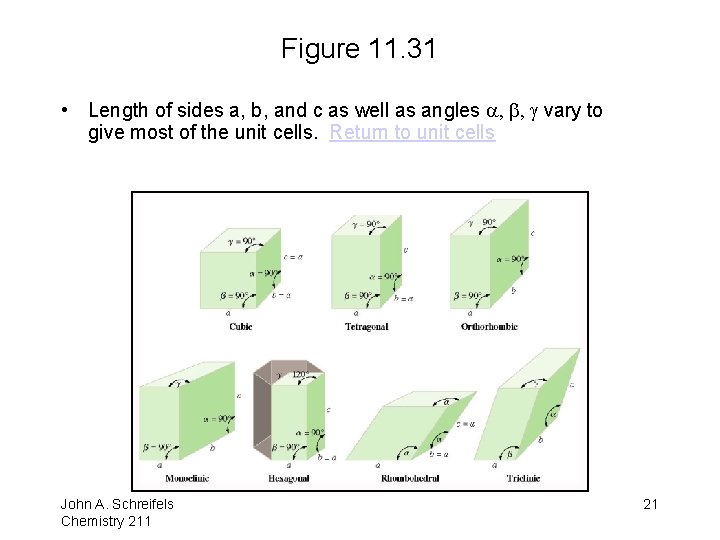
Unit Cells in Crystalline Solids • Metal crystals made up of atoms in regular arrays – the smallest of repeating array of atoms is called the unit cell. • There are 14 different unit cells that are observed which vary in terms of the angles between atoms some are 90°, but others are not. Go to Figure 11. 31 8– 16 John A. Schreifels Chemistry 211 16

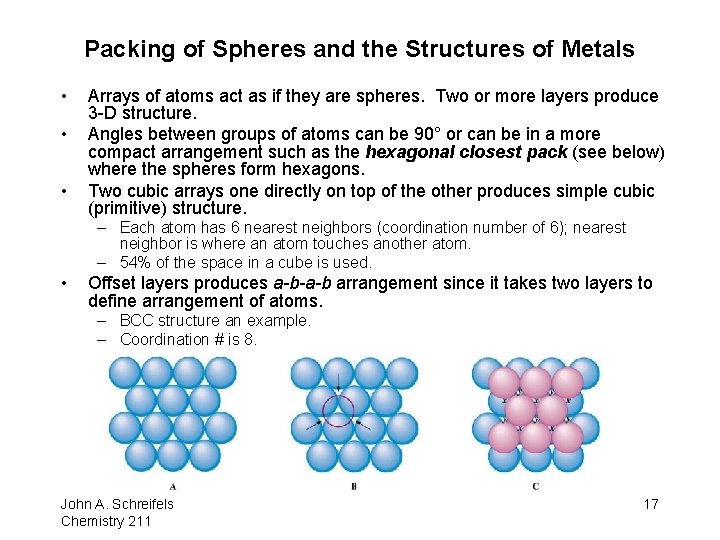
Packing of Spheres and the Structures of Metals • • • Arrays of atoms act as if they are spheres. Two or more layers produce 3 -D structure. Angles between groups of atoms can be 90° or can be in a more compact arrangement such as the hexagonal closest pack (see below) where the spheres form hexagons. Two cubic arrays one directly on top of the other produces simple cubic (primitive) structure. – Each atom has 6 nearest neighbors (coordination number of 6); nearest neighbor is where an atom touches another atom. – 54% of the space in a cube is used. • Offset layers produces a-b-a-b arrangement since it takes two layers to define arrangement of atoms. – BCC structure an example. – Coordination # is 8. 8– 17 John A. Schreifels Chemistry 211 17

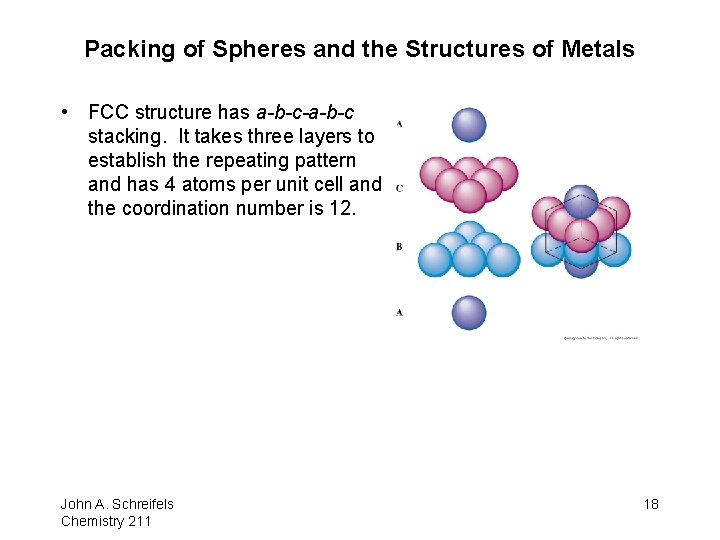
Packing of Spheres and the Structures of Metals • FCC structure has a-b-c-a-b-c stacking. It takes three layers to establish the repeating pattern and has 4 atoms per unit cell and the coordination number is 12. 8– 18 John A. Schreifels Chemistry 211 18

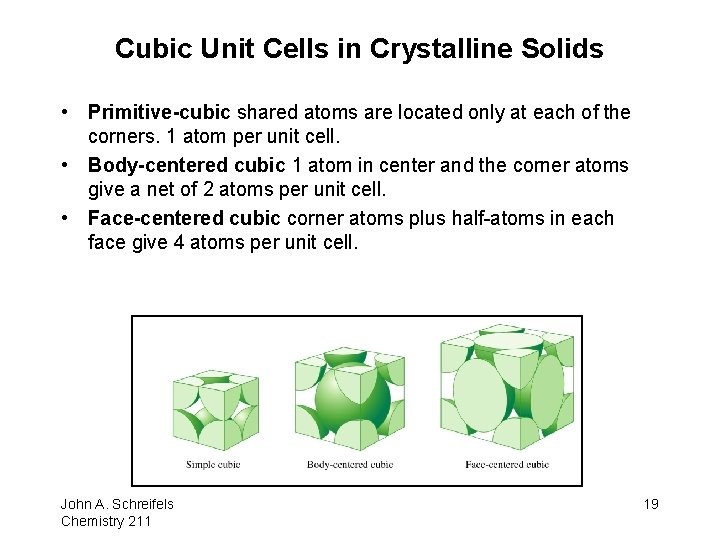
Cubic Unit Cells in Crystalline Solids • Primitive-cubic shared atoms are located only at each of the corners. 1 atom per unit cell. • Body-centered cubic 1 atom in center and the corner atoms give a net of 2 atoms per unit cell. • Face-centered cubic corner atoms plus half-atoms in each face give 4 atoms per unit cell. 8– 19 John A. Schreifels Chemistry 211 19

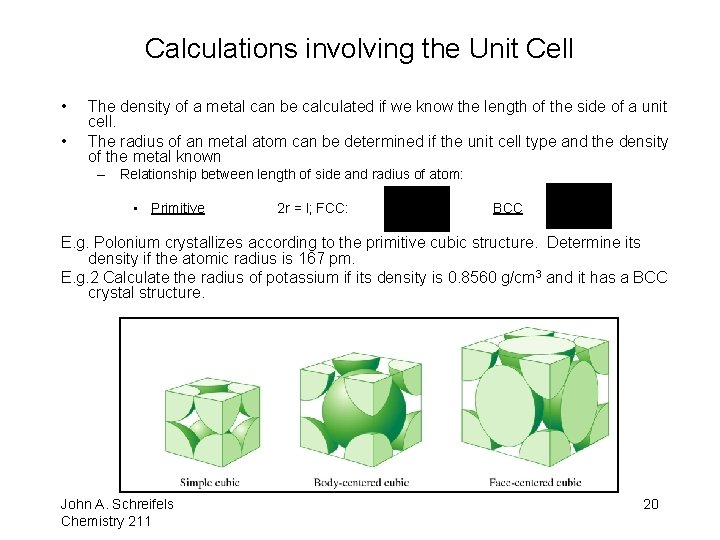
Calculations involving the Unit Cell • • The density of a metal can be calculated if we know the length of the side of a unit cell. The radius of an metal atom can be determined if the unit cell type and the density of the metal known – Relationship between length of side and radius of atom: • Primitive 2 r = l; FCC: BCC E. g. Polonium crystallizes according to the primitive cubic structure. Determine its density if the atomic radius is 167 pm. E. g. 2 Calculate the radius of potassium if its density is 0. 8560 g/cm 3 and it has a BCC crystal structure. 8– 20 John A. Schreifels Chemistry 211 20

Figure 11. 31 • Length of sides a, b, and c as well as angles a, b, g vary to give most of the unit cells. Return to unit cells 8– 21 John A. Schreifels Chemistry 211 21