Arshak Grigoryan Project for Math Modeling PredatorPrey model

Arshak Grigoryan Project for Math. Modeling

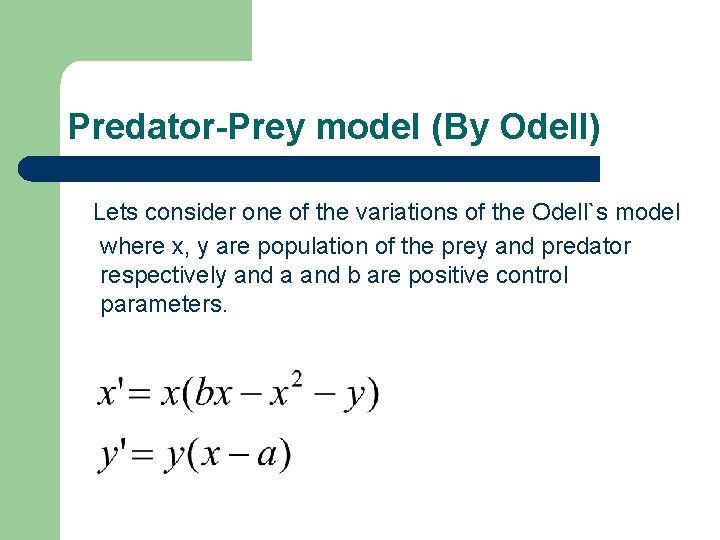
Predator-Prey model (By Odell) Lets consider one of the variations of the Odell`s model where x, y are population of the prey and predator respectively and a and b are positive control parameters.

Bifurcation We are already familiar with the bifurcation in one dimensional systems. In this example we consider two dimensional system with two parameters a and b. And on this model I would like to present Hopf bifurcation.

Bifurcation Definition. If the phase portrait changes its topological structure as parameters are varied, we say that a bifurcation has occurred. Examples include changes in the number or stability of fixed points, closed orbits or saddle connections as a parameter is varied.

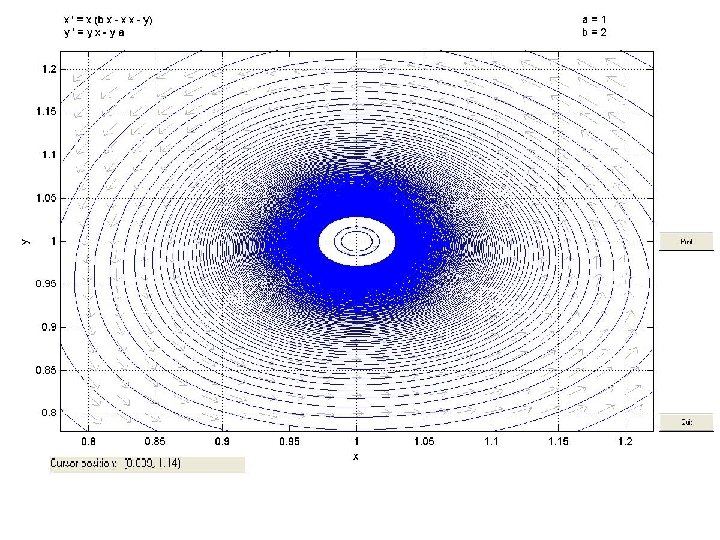
Closed Orbit and Limit Cycle Closed Orbit: If a phase point starting anywhere else would circulate around the origin and eventually return to its starting point. Limit cycle: A limit cycle is an isolated closed trajectory. Isolated means that neighboring trajectories are not closed; they spiral either toward or away from the limit cycle.

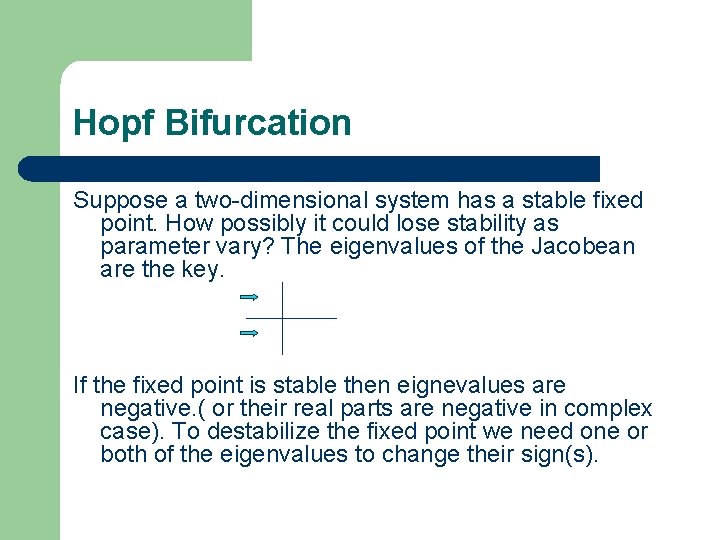
Hopf Bifurcation Suppose a two-dimensional system has a stable fixed point. How possibly it could lose stability as parameter vary? The eigenvalues of the Jacobean are the key. If the fixed point is stable then eignevalues are negative. ( or their real parts are negative in complex case). To destabilize the fixed point we need one or both of the eigenvalues to change their sign(s).

Predator-Prey Now let's investigate our system. Lets look at the equilibrium solutions of the system. To find them we need solutions of the system

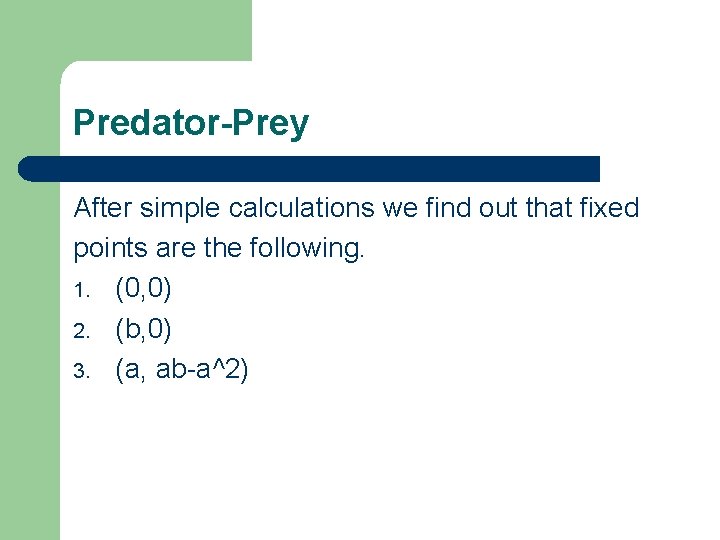
Predator-Prey After simple calculations we find out that fixed points are the following. 1. (0, 0) 2. (b, 0) 3. (a, ab-a^2)

Predator-Prey Now we will need Jacobean of the system in order to analyze stability of equilibrium solutions.

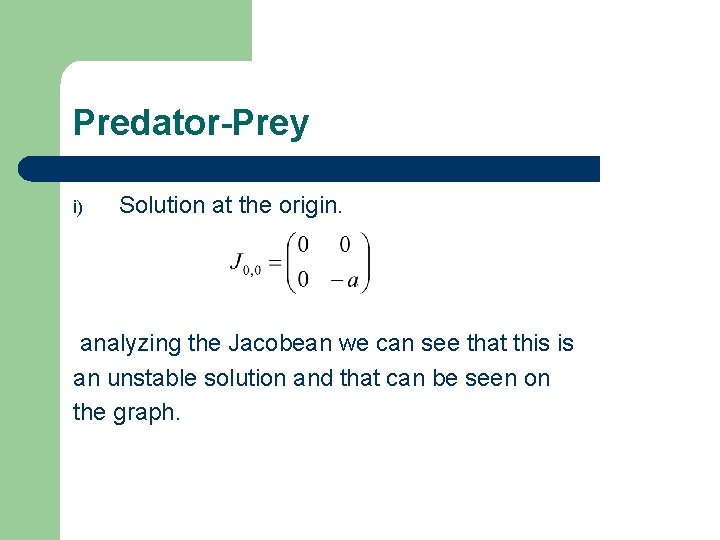
Predator-Prey i) Solution at the origin. analyzing the Jacobean we can see that this is an unstable solution and that can be seen on the graph.

Equilibrium at (b, 0) ii) Thus eigenvalues are and. We can see that first eigenvalue always negative and when b<a second eigenvalue is negative also and for that reason solution will be stable.

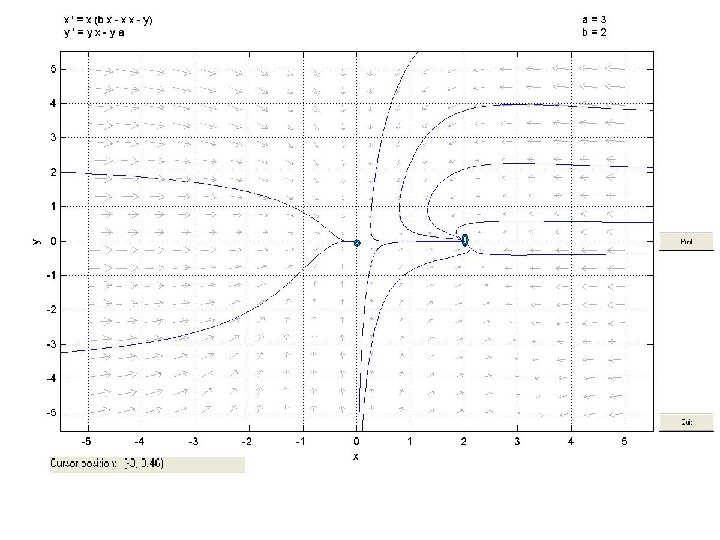
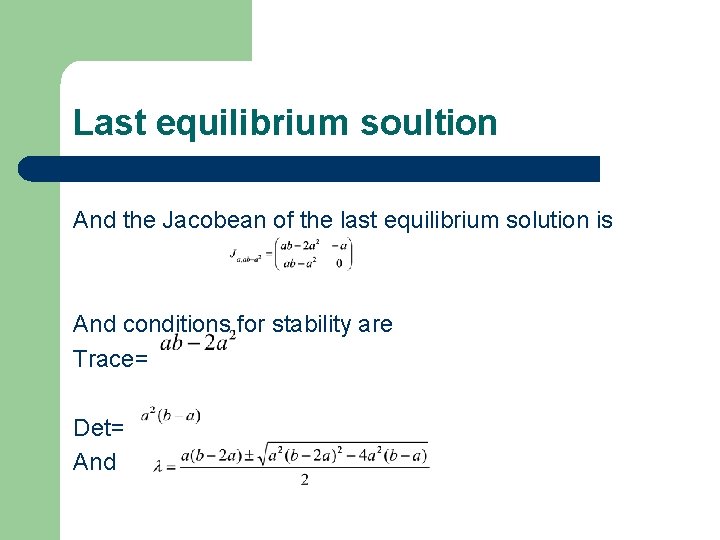
Last equilibrium soultion And the Jacobean of the last equilibrium solution is And conditions for stability are Trace= Det= And

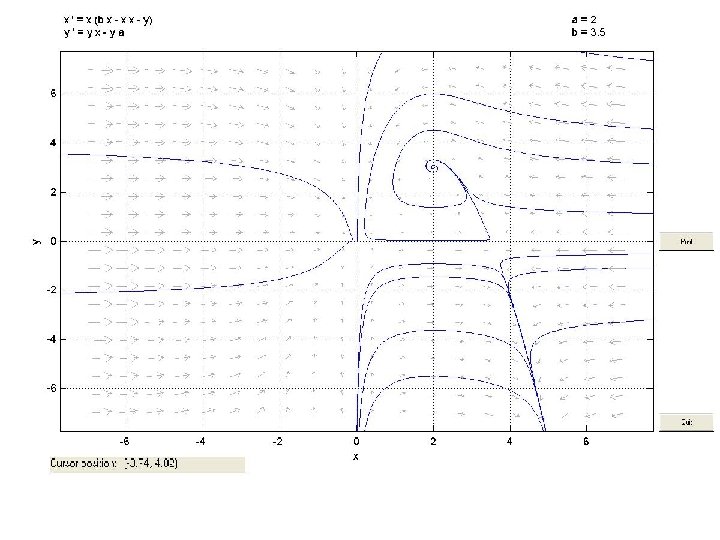
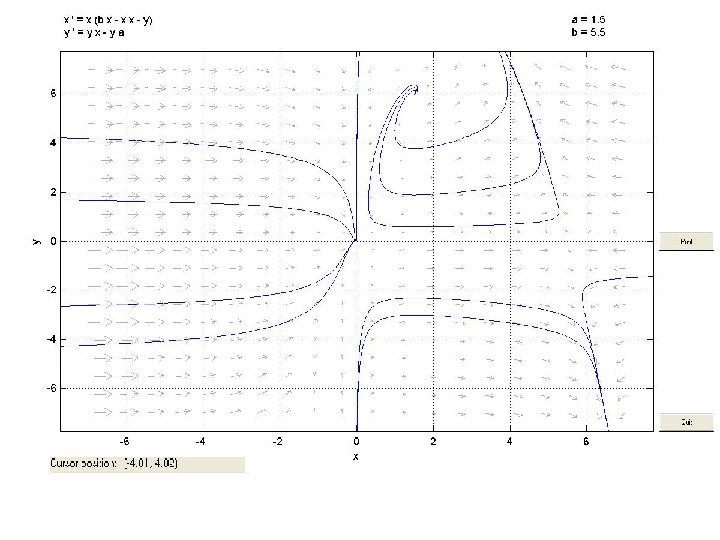
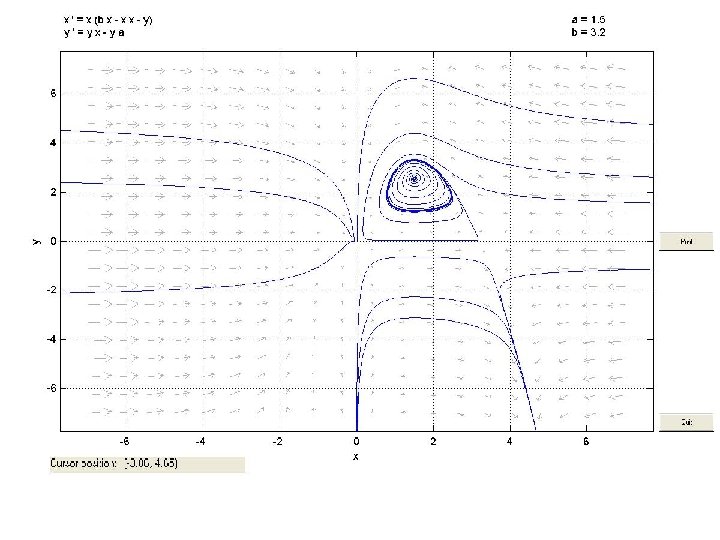
Last equilibrium solution Now we clearly can see that change of the sign of eigenvalues occur at b=2 a and that is the point where Hopf bifurcation happens. So the stability of the solution we need a<b<2 a. We can see all this on the graphs.