Arithmetic Sequences Series Honors PreCalc 10 2 Arithmetic

Arithmetic Sequence • Difference between successive terms is a constant • The constant is

• Each term in the arithmetic sequence is found by adding the common

Example 2 • Find an explicit formula for the nth term of the arithmetic

Example 3: The formula for nth term of an arithmetic sequences can be used

Arithmetic Mean • If two non consecutive terms of an arithmetic sequence are known,

Example 4: • Write an arithmetic sequence that has four arithmetic means between 4.

Arithmetic Series • The indicated sum of the terms of an arithmetic sequence •

Example 5: Find the indicated sum of each arithmetic series a) -5 + 2

Example 6: • A video game tournament, in which gamers compete in multiple games

Example 7: • Carter has been collecting baseball cards since his father gave him

Slides: 11

Download presentation

Arithmetic Sequences & Series Honors Pre-Calc 10. 2

Arithmetic Sequence • Difference between successive terms is a constant • The constant is referred to as the common difference, denoted d Example 1: Determine the common difference and find the next four terms of the arithmetic sequence. 244, 187, 130, …

• Each term in the arithmetic sequence is found by adding the common difference to the preceding term. Therefore, the recursive formula below can be used. • However, we most likely will be using an explicit formula instead. • Continuing to use the formula above from n=1 to additional values will get us the following explicit formula.

Example 2 • Find an explicit formula for the nth term of the arithmetic sequence 12, 21, 30, … • First find the common difference • Sub a 1 and d into formula for nth term

Example 3: The formula for nth term of an arithmetic sequences can be used to find specific terms w/in the sequence. a) Find the 68 th term of the arithmetic sequence 25, 17, 9, … b) Find the first term of the arithmetic sequence for which a 25 = 139 and d = ¾. *if d value is not given, we need to find that first!

Arithmetic Mean • If two non consecutive terms of an arithmetic sequence are known, the terms between them can be found. These missing terms are known as the arithmetic means.

Example 4: • Write an arithmetic sequence that has four arithmetic means between 4. 3 and 12. 8. • First find the common difference • Then determine the arithmetic means

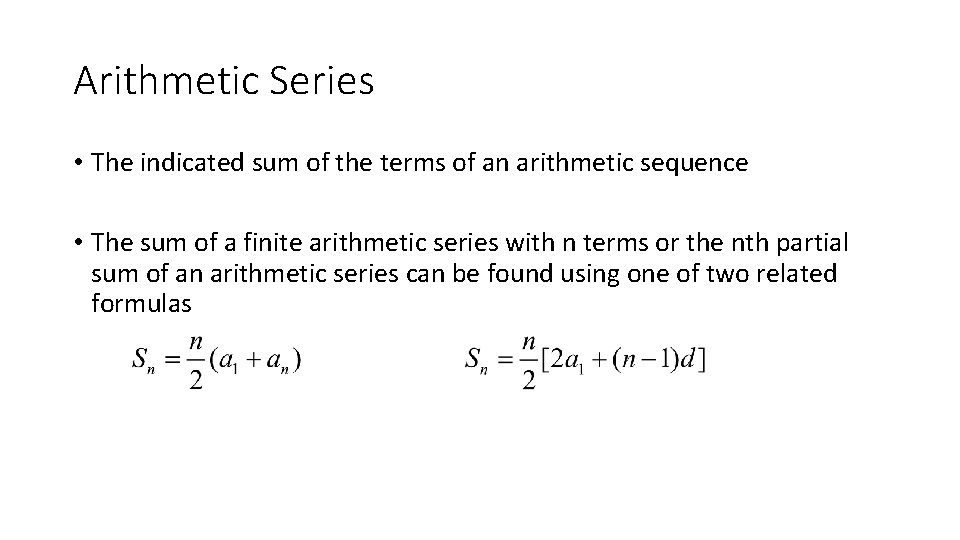
Arithmetic Series • The indicated sum of the terms of an arithmetic sequence • The sum of a finite arithmetic series with n terms or the nth partial sum of an arithmetic series can be found using one of two related formulas

Example 5: Find the indicated sum of each arithmetic series a) -5 + 2 + 9 + … + 317 b) The 28 th partial sum of 27 + 14 + 1 + … c)

Example 6: • A video game tournament, in which gamers compete in multiple games and accumulate an overall score, pay the top 20 finishers. First place receives $5000, second place receives $4800, third place receives $4600 and so on. How much total prize money is awarded?

Example 7: • Carter has been collecting baseball cards since his father gave him a 20 -card collection. During each month, Carter’s father gives him 5 more cards than the previous month. In how many months will Carter reach 1000 cards?