Arithmetic Sequences Dr Shildneck Arithmetic Sequences An arithmetic

Arithmetic Sequences Dr. Shildneck

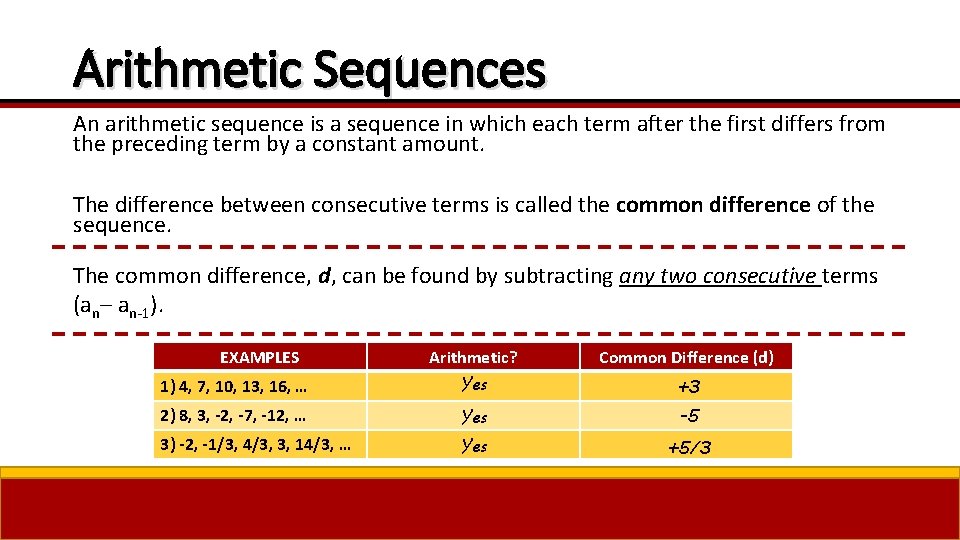
Arithmetic Sequences An arithmetic sequence is a sequence in which each term after the first differs from the preceding term by a constant amount. The difference between consecutive terms is called the common difference of the sequence. The common difference, d, can be found by subtracting any two consecutive terms (an– an-1). EXAMPLES Arithmetic? Common Difference (d) 1) 4, 7, 10, 13, 16, … Yes +3 2) 8, 3, -2, -7, -12, … Yes -5 3) -2, -1/3, 4/3, 3, 14/3, … Yes +5/3

Arithmetic Functions An arithmetic function with domain = {all positive integers} and range = {an} has a graph that consists of (only) points that lie on a straight line. Thus, an arithmetic sequence can be thought of as a linear function whose domain is the set of all positive integers. Furthermore, the common difference, d, is the rate of change of the function. Thus, d, is also the slope of the arithmetic/linear function.

Finding the Equation of an Arithmetic Function 1. Find the “slope” (the common difference) 2. Compare each term value to its input (position) in the sequence. 3. Write an equations (y = mx + b) that makes each input result in the appropriate term. (b is the adjustment that needs to be made) [Example 1] Write the equation for the sequence -3, 1, 5, 9, 13, 17, 21, 25, …

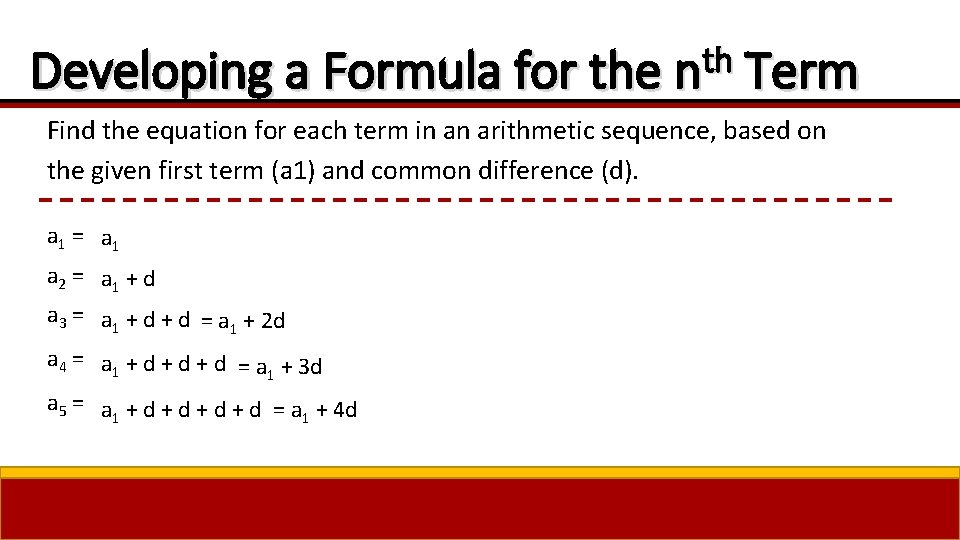
th Developing a Formula for the n Term Find the equation for each term in an arithmetic sequence, based on the given first term (a 1) and common difference (d). a 1 = a 1 a 2 = a 1 + d a 3 = a 1 + d = a + 2 d 1 a 4 = a 1 + d + d = a + 3 d 1 a 5 = a + d + d = a + 4 d 1 1

th Developing a Formula for the n Term Now looking at the pattern below, how might we write an equation for the nth term (an) based on the first term (a 1) and the common difference (d). a 1 = a 1 Hint: Compare the “n” to the number of d’s required to get the nth term. a 2 = a 1 + d a 3 = a 1 + 2 d a 4 = a 1 + 3 d a 5 = a 1 + 4 d What do you notice about n and the number of d’s you need for each term?

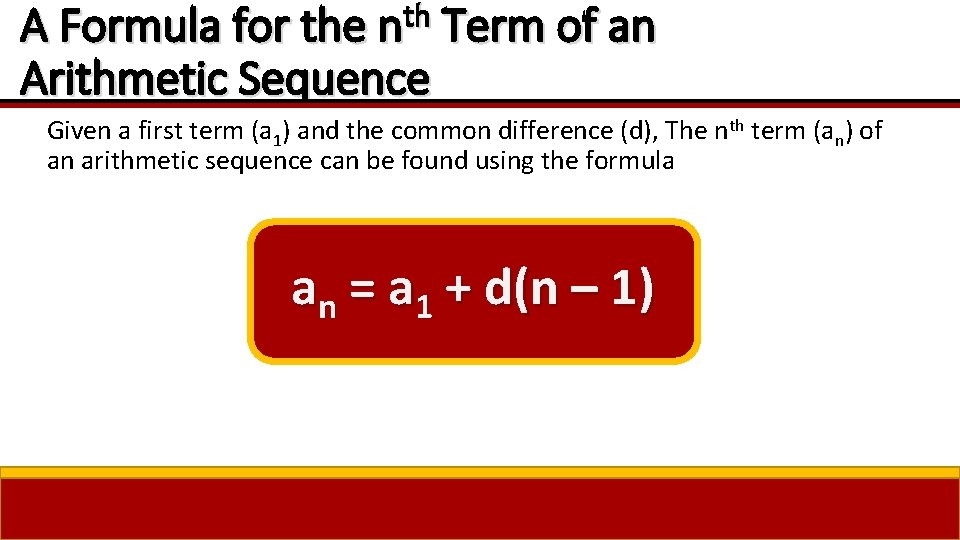
th A Formula for the n Arithmetic Sequence Term of an Given a first term (a 1) and the common difference (d), The nth term (an) of an arithmetic sequence can be found using the formula an = a 1 + d(n – 1)

Examples [Example 2] Find the 8 th term of the arithmetic sequence whose first term is 4 and common difference is -7.

Examples [Example 3] Find the nth term (formula) for the sequence. -2, 4, 10, 16, 22, 28, 34, 40, …

Examples [Example 4] Find the formula for the nth term of the sequence.

Examples [Example 5] Find the nth term of of an arithmetic sequence whose first term is 4 and fifth term in 40.

Examples [Example 6] Find the formula for the nth term of an arithmetic sequence whose 7 th term is 86 and 18 th term is 53.

ASSIGNMENT YOUR TEXT Page 605 #1 -25 odd