Algebraic Expressions Objectives 1 To evaluate algebraic expressions

Algebraic Expressions Objectives: 1) To evaluate algebraic expressions 2) To simplify algebraic expressions

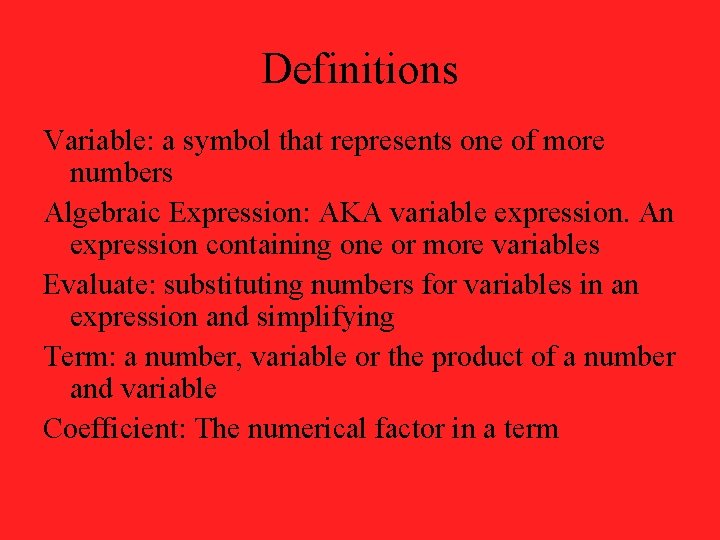
Definitions Variable: a symbol that represents one of more numbers Algebraic Expression: AKA variable expression. An expression containing one or more variables Evaluate: substituting numbers for variables in an expression and simplifying Term: a number, variable or the product of a number and variable Coefficient: The numerical factor in a term

Properties for Simplifying Algebraic Expressions Definition of Subtraction: a – b = a + (-b) Definition of Division a ÷ b = a/b = a 1/b, b ≠ 0 Distributive Property a(b + c) = ab + ac ; a(b – c) = ab – ac Zero Product Property 0 a=0

Properties for Simplifying Algebraic Expressions (continued) Multiplicative Inverse: -1 a = -a Opposite of a Sum: - (a + b) = -a + -b Opposite of a Difference - (a – b) = b – a

Properties for Simplifying Algebraic Expressions (continued) Opposite of a Product § -(ab) = -a b = a -b Opposite of an Opposite § -(-a) = a

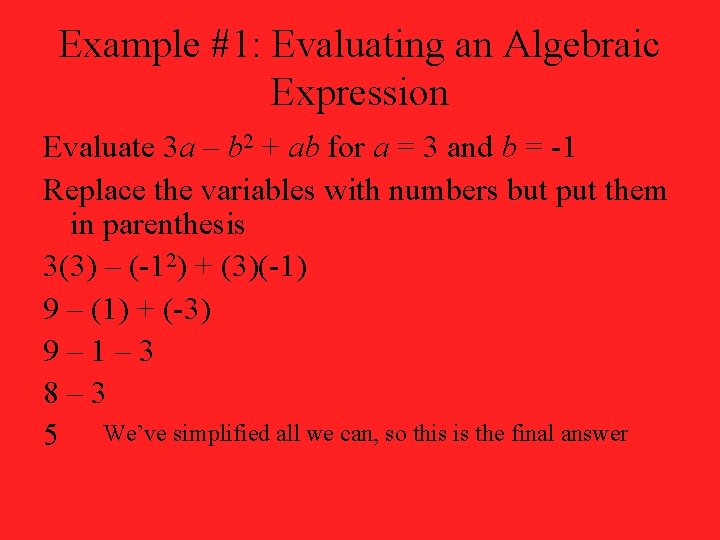
Example #1: Evaluating an Algebraic Expression Evaluate 3 a – b 2 + ab for a = 3 and b = -1 Replace the variables with numbers but put them in parenthesis 3(3) – (-12) + (3)(-1) 9 – (1) + (-3) 9– 1– 3 8– 3 5 We’ve simplified all we can, so this is the final answer

Example #2: Combining Like Terms a. 3 k – k § 2 k b. 5 z 2 – 10 z – 8 z 2 + z § 5 z 2 – 8 z 2 – 10 z + z § -3 z 2 – 9 z c. - (m + n) + 2(m – 3 n) § - m – n + 2 m – 6 n § - m + 2 m – n – 6 n § m – 7 n