6 5 Combining Probabilities Supplementary Section LEARNING GOAL

6. 5 Combining Probabilities (Supplementary Section) LEARNING GOAL Distinguish between independent and dependent events and between overlapping and non-overlapping events, and be able to calculate and either/or probabilities. Copyright © 2009 Pearson Education, Inc.

And Probabilities Suppose you toss two fair dice and want to know the probability that both will come up 4. For each die, the probability of a 4 is 1/6. We find the probability that both dice show a 4 by multiplying the individual probabilities: P(double 4’s) = P(4) × P(4) = × = 1 1 1 6 6 36 In general, we call the probability of event A and event B occurring an and probability (or joint probability). Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 2

And Probabilities Independent Events And Probability for Independent Events Two events are independent if the outcome of one event does not affect the probability of the other event. Consider two independent events A and B with probabilities P(A) and P(B). The probability that A and B occur together is P(A and B) = P(A) × P(B) This principle can be extended to any number of independent events. For example, the probability of A, B, and a third independent event C is P(A and B and C) = P(A) × P(B) × P(C) Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 3

EXAMPLE 1 Three Coins Suppose you toss three fair coins. What is the probability of getting three tails? Solution: Because coin tosses are independent, we multiply the probability of tails on each individual coin: 1 P(3 tails) = P(tails) × P(tails) = 2 coin 1 coin 2 coin 3 × 1 2 = 1 8 The probability that three tossed coins all land on tails is 1/8 (which we determined in Example 8 of Section 6. 2 with much more work). Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 4

And Probabilities Independent Events Dependent Events A batch of 15 memory cards contains 5 defective cards. What is the probability of getting a defect card on both the first and the second selection? P(2 defectives) = P(defective) × P(defective) = 5 15 × 4 14 Copyright © 2009 Pearson Education, Inc. = 0. 0952 Slide 6. 5 - 5
And Probability for Dependent Events Two events are dependent if the outcome of one event affects the probability of the other event. The probability that dependent events A and B occur together is P(A and B) = P(A) × P(B given A) where P(B given A) means the probability of event B given the occurrence of event A. This principle can be extended to any number of individual events. For example, the probability of dependent events A, B, and C is P(A and B and C) = P(A) × P(B given A) × P(C given A and B) Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 6

TECHNICAL NOTE P(B given A) is called a conditional probability. In some books, it is denoted P(B|A). Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 7

TIME OUT TO THINK Without doing calculations, compare the probability of drawing three hearts in a row when the card is replaced and the deck is shuffled after each draw to the probability of drawing three hearts in a row without replacement. Which probability is larger and why? Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 8

EXAMPLE 3 When Can We Treat Dependent Events as Independent Events? A polling organization has a list of 1, 000 people for a telephone survey. The pollsters know that 433 people out of the 1, 000 are members of the Democratic Party. Assuming that a person cannot be called more than once, what is the probability that the first two people called will be members of the Democratic Party? Solution: This problem involves an and probability for dependent events: Once a person is called, that person cannot be called again. The probability of calling a member of the Democratic Party on the first call is 433/1, 000. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 9

EXAMPLE 3 When Can We Treat Dependent Events as Independent Events? Solution: (cont. ) With that person removed from the calling pool, the probability of calling a member of the Democratic Party on the second call is 432/999. Therefore, the probability of calling two members of the Democratic Party on the first two calls is 432 433 × 999 = 0. 1872 1, 000 Copyright © 2009 Pearson Education, Inc. Slide 6. 5 -10

EXAMPLE 3 When Can We Treat Dependent Events as Independent Events? Solution: (cont. ) If we treat the two calls as independent events, the probability of calling a member of the Democratic Party is 433/1, 000 on both calls. Then the probability of calling two members of the Democratic Party is 433 1, 000 × 1000 = 0. 1875 which is nearly identical to the result assuming dependent events (previous slide). Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 11

EXAMPLE 3 When Can We Treat Dependent Events as Independent Events? Solution: (cont. ) In general, if relatively few items or people are selected from a large pool (in this case, 2 people out of 1, 000), then dependent events can be treated as independent events with very little error. A common guideline is that independence can be assumed when the sample size is less than 5% of the population size. This practice is commonly used by polling organizations. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 12

Either/Or Probabilities Non-Overlapping Events Figure 6. 14 Venn diagram for non-overlapping events. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 13

Either/Or Probability for Non-Overlapping Events Two events are non-overlapping if they cannot occur at the same time. If A and B are non-overlapping events, the probability that either A or B occurs is P(A or B) = P(A) + P(B) This principle can be extended to any number of nonoverlapping events. For example, the probability that either event A, event B, or event C occurs is P(A or B or C) = P(A) + P(B) + P(C) provided that A, B, and C are all non-overlapping events. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 14

EXAMPLE 5 Either/Or Dice Probability Suppose you roll a single die. What is the probability of getting an even number? Solution: The even outcomes of 2, 4, and 6 are non- overlapping because a single die can yield only one result. We know that P(2) = 1/6, P(4) = 1/6, and P(6) = 1/6. Therefore, the combined probability is P(2 or 4 or 6) = P(2) + P(4) + P(6) = 1 1 + + = 6 6 2 6 The probability of rolling an even number is 1/2. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 15

Either/Or Probabilities Non-Overlapping Events To improve tourism between France and the United States, the two governments form a committee consisting of 20 people: 2 American men, 4 French men, 6 American women, and 8 French women (as shown in Table 6. 9). If you meet one of these people at random, what is the probability that the person will be either a woman or a French person? Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 16
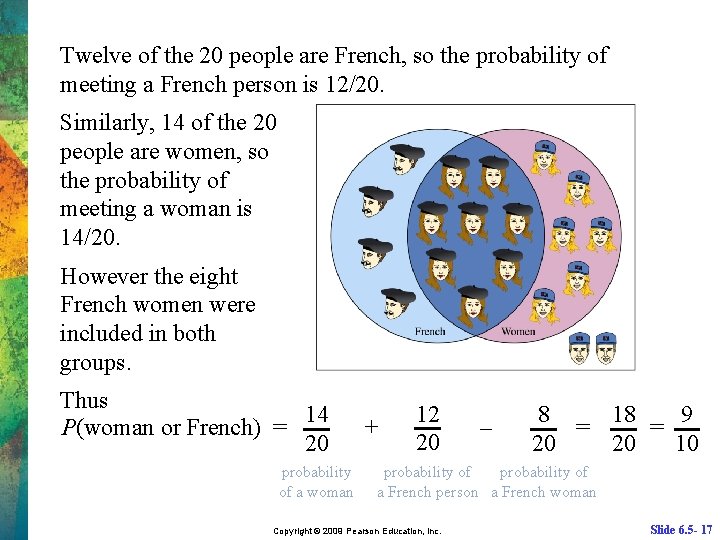
Twelve of the 20 people are French, so the probability of meeting a French person is 12/20. Similarly, 14 of the 20 people are women, so the probability of meeting a woman is 14/20. However the eight French women were included in both groups. Thus 14 P(woman or French) = 20 probability of a woman + 12 20 – 8 18 9 = = 20 20 10 probability of a French person a French woman Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 17

We say that meeting a woman and meeting a French person are overlapping (or non–mutually exclusive) events because both can occur at the same time. Either/Or Probability for Overlapping Events Two events A and B are overlapping if they can occur together. The probability that either A or B occurs is P(A or B) = P(A) + P(B) – P(A and B) The last term, P(A and B), corrects for the double counting of events in which A and B both occur together. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 18

TIME OUT TO THINK Are the events of being born on a Wednesday or being born in Las Vegas overlapping? Are the events of being born on a Wednesday or being born in March overlapping? Are the events of being born on a Wednesday or being born on a Friday overlapping? Explain. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 19
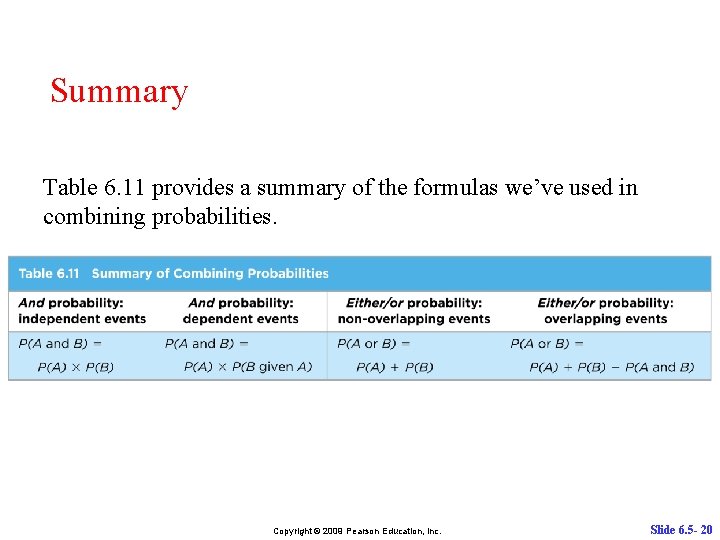
Summary Table 6. 11 provides a summary of the formulas we’ve used in combining probabilities. Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 20

The End Copyright © 2009 Pearson Education, Inc. Slide 6. 5 - 21
- Slides: 21