6 2 Solving Systems Using Substitution Hubarth Algebra

6 -2 Solving Systems Using Substitution Hubarth Algebra

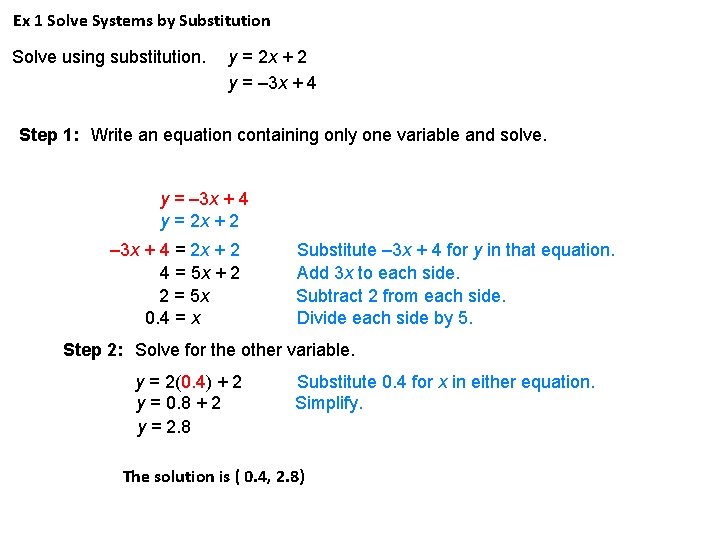
Ex 1 Solve Systems by Substitution Solve using substitution. y = 2 x + 2 y = – 3 x + 4 Step 1: Write an equation containing only one variable and solve. y = – 3 x + 4 y = 2 x + 2 – 3 x + 4 = 2 x + 2 4 = 5 x + 2 2 = 5 x 0. 4 = x Substitute – 3 x + 4 for y in that equation. Add 3 x to each side. Subtract 2 from each side. Divide each side by 5. Step 2: Solve for the other variable. y = 2(0. 4) + 2 y = 0. 8 + 2 y = 2. 8 Substitute 0. 4 for x in either equation. Simplify. The solution is ( 0. 4, 2. 8)

Ex 2 Using Substitution and the Distributive Property Solve using substitution. – 2 x + y = – 1 4 x + 2 y = 12 Step 1: Solve the first equation for y because it has a coefficient of 1. – 2 x + y = – 1 y = 2 x – 1 Add 2 x to each side. Step 2: Write an equation containing only one variable and solve. 4 x + 2 y = 12 Start with the other equation. 4 x + 2(2 x – 1) = 12 Substitute 2 x – 1 for y in that equation. 4 x + 4 x – 2 = 12 Use the Distributive Property. 8 x = 14 Combine like terms and add 2 to each side. x = 1. 75 Divide each side by 8. Step 3: Solve for y in the other equation. – 2(1. 75) + y = – 1 – 3. 5 + y = – 1 y = 2. 5 The solution is (1. 75, 2. 5) Substitute 1. 75 for x. Simplify. Add 3. 5 to each side.

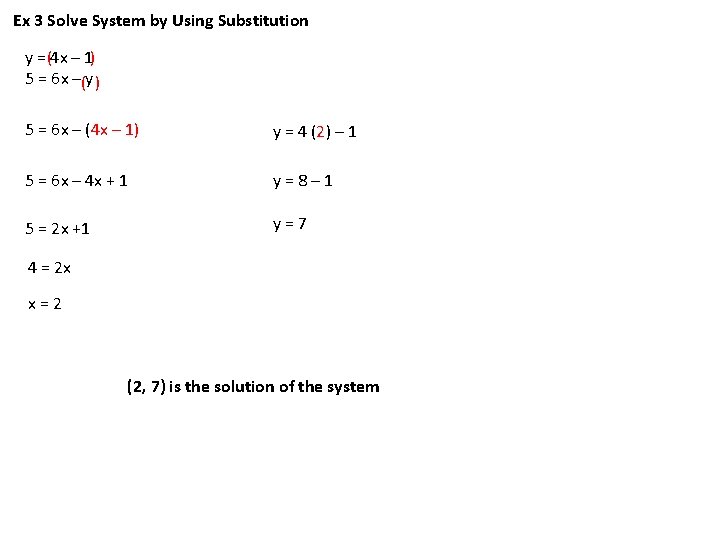
Ex 3 Solve System by Using Substitution y = (4 x – 1) 5 = 6 x –(y ) 5 = 6 x – (4 x – 1) y = 4 (2) – 1 5 = 6 x – 4 x + 1 y=8– 1 5 = 2 x +1 y=7 4 = 2 x x=2 (2, 7) is the solution of the system

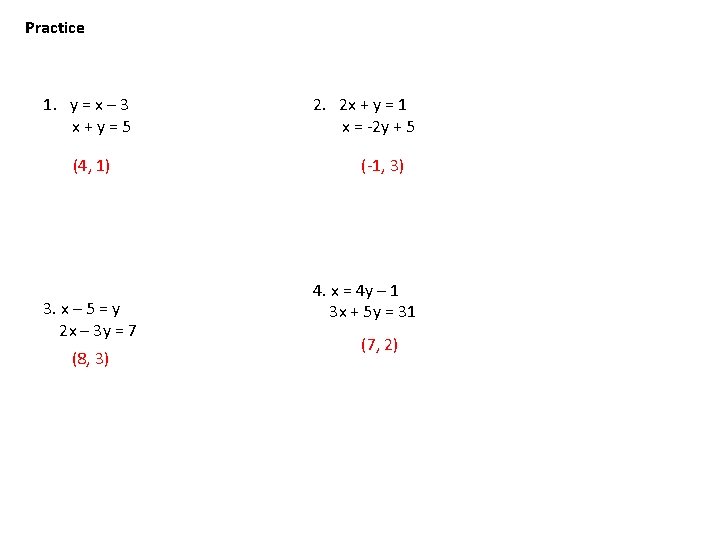
Practice 1. y = x – 3 x+y=5 (4, 1) 3. x – 5 = y 2 x – 3 y = 7 (8, 3) 2. 2 x + y = 1 x = -2 y + 5 (-1, 3) 4. x = 4 y – 1 3 x + 5 y = 31 (7, 2)