5 1 Rate of Change and Slope Rate

Rate of Change can be presented in many forms such as: We can use

Choosing the data from: Time and Distance 1 min 260 ft. 2 min 520

Choosing the data from: Time and Distance 1 min 260 ft. 3 min 780

Choosing the data from: Time and Distance 1 min 260 ft. 4 min 1040

NOTE: When we get the same slope, no matter what date points we get,

YOU TRY IT: Determine whether the following rate of change is constant in the

Choosing the data from: Gallons and Miles 1 g 28 m 3 g 84

Choosing the data from: Gallons and Miles 1 g 28 m. 5 g 140

Remember: Rate of Change can be presented in many forms: We can use the

Using the data for A and B and the definition of rate of change

Using the data for B and C and the definition of rate of change

Using the data for C and D and the definition of rate of change

Comparing the slopes of the three: However, we must find all the combination that

Finally: Finally we can conclude that the poles with the steepest path are poles

Class Work: Pages: 295 -297 Problems: 1, 4, 8, 9,

Remember: When we get the same slope, no matter what date points we get,

When we get the same slope, no matter what date points we get, we

We further use the concept of rise/run to find the slope: run rise Make

CONSTANT rate of change: due to the fact that a line is has no

YOU TRY IT: Provide the slope of the line that passes through the points

YOU TRY IT: (Solution) Using the given data A(1, 3) and B(5, 5) and

YOU TRY IT: (Solution) Choosing two points say: A(-5, 3) and B(1, 5) and

YOU TRY IT: (Solution) Choosing two points say: A(-1, 2) and B(-1, -1) and

THEREFORE: Horizontal ( slope of ZERO ) lines have a While vertical ( )

VIDEOS: Graphs https: //www. khanacademy. org/math/algebra/line ar-equations-and-inequalitie/slope-andintercepts/v/slope-and-rate-of-change

Class Work: Pages: 295 -297 Problems: As many as needed to master the concept

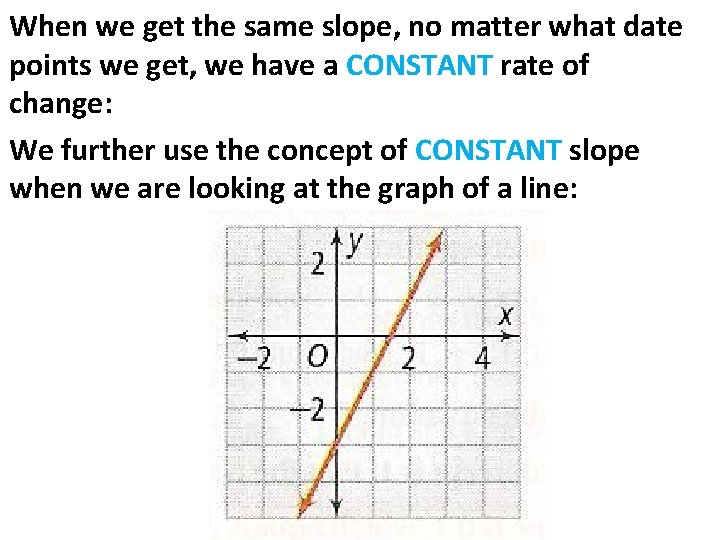
Slides: 33

Download presentation

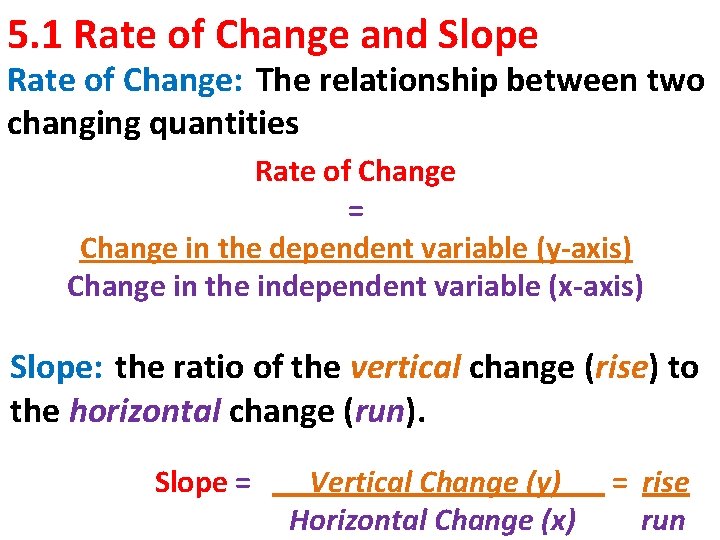
5. 1 Rate of Change and Slope Rate of Change: The relationship between two changing quantities Rate of Change = Change in the dependent variable (y-axis) Change in the independent variable (x-axis) Slope: the ratio of the vertical change (rise) to the horizontal change (run). Slope = Vertical Change (y) Horizontal Change (x) = rise run

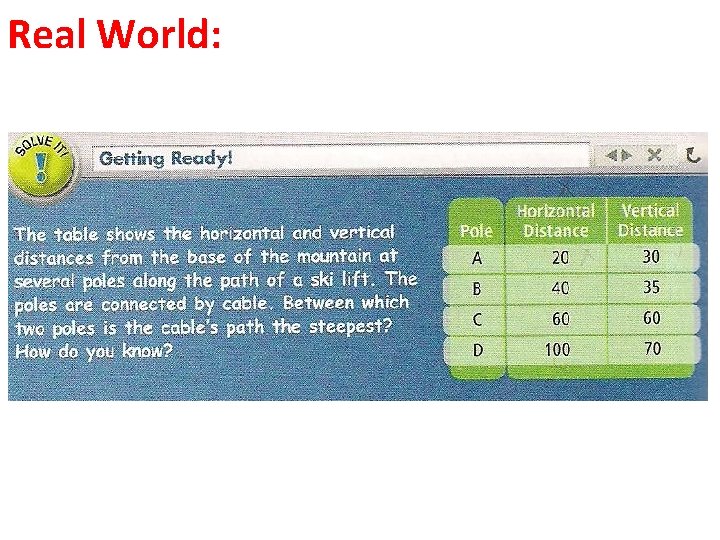
Real World:

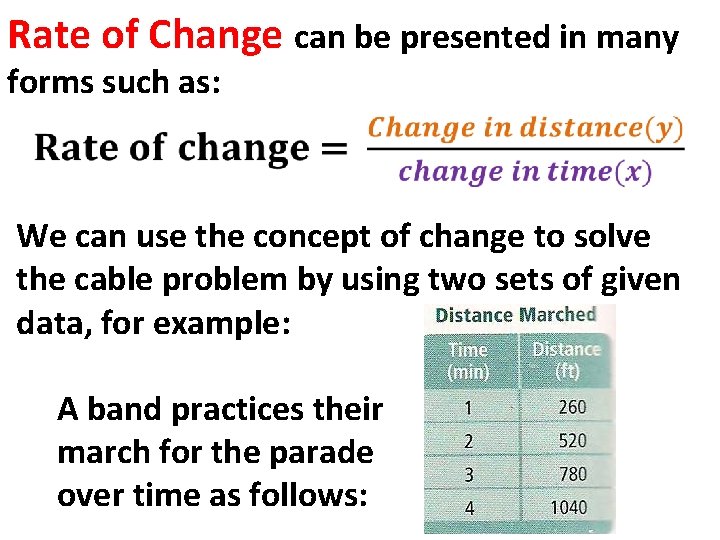
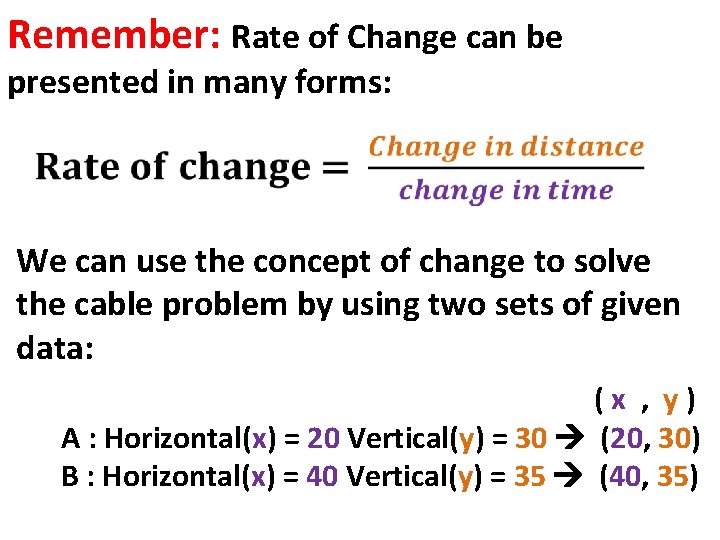
Rate of Change can be presented in many forms such as: We can use the concept of change to solve the cable problem by using two sets of given data, for example: A band practices their march for the parade over time as follows:

Choosing the data from: Time and Distance 1 min 260 ft. 2 min 520 ft. We have the following:

Choosing the data from: Time and Distance 1 min 260 ft. 3 min 780 ft. We have the following:

Choosing the data from: Time and Distance 1 min 260 ft. 4 min 1040 ft. We have the following:

NOTE: When we get the same slope, no matter what date points we get, we have a CONSTANT rate of change:

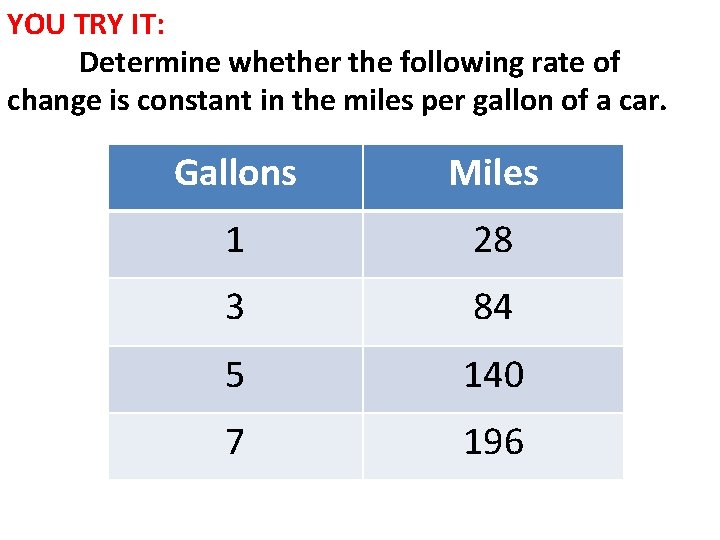
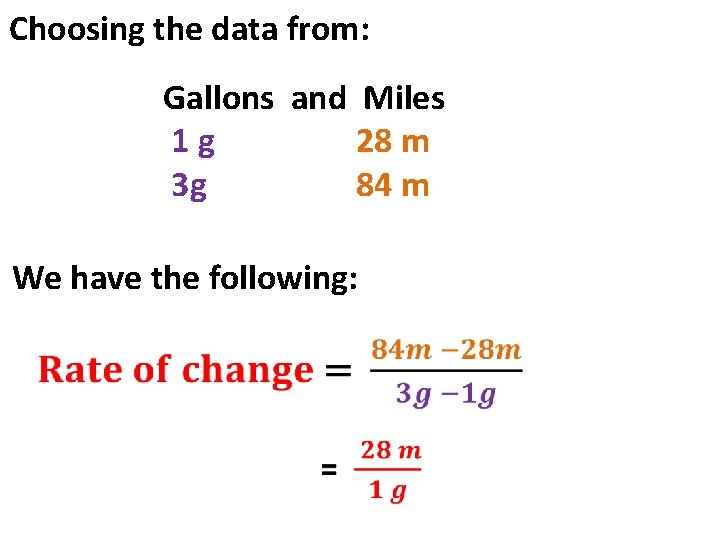
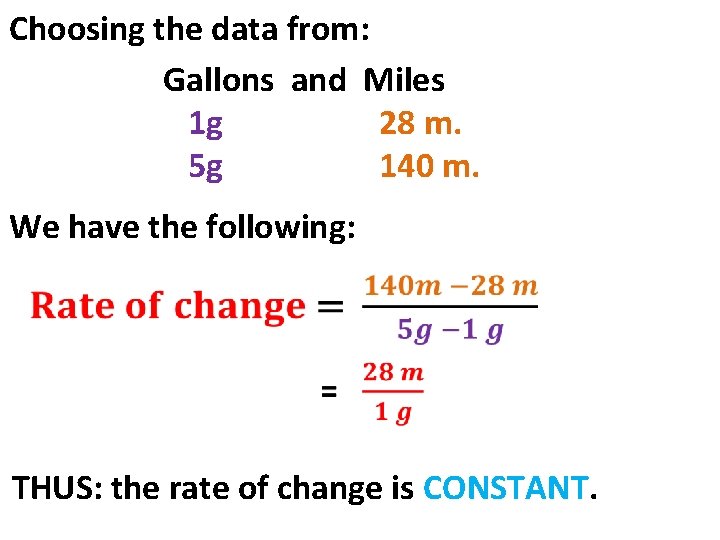
YOU TRY IT: Determine whether the following rate of change is constant in the miles per gallon of a car. Gallons Miles 1 28 3 84 5 140 7 196

Choosing the data from: Gallons and Miles 1 g 28 m 3 g 84 m We have the following:

Choosing the data from: Gallons and Miles 1 g 28 m. 5 g 140 m. We have the following: THUS: the rate of change is CONSTANT.

Once Again: Real World X Y

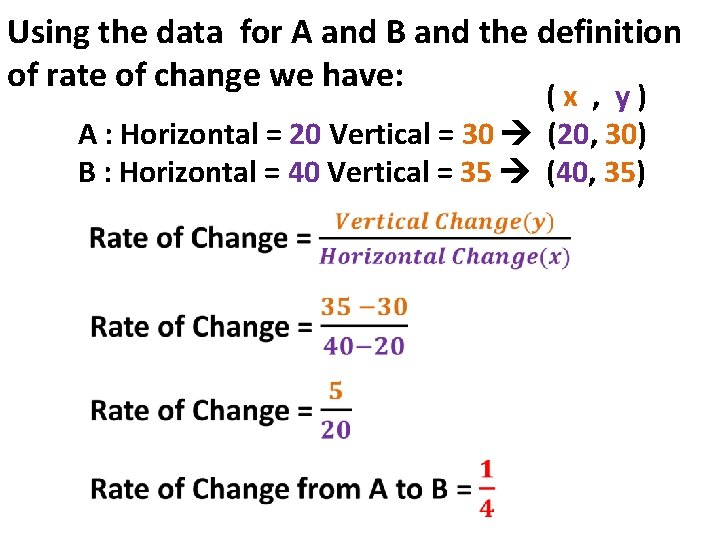
Remember: Rate of Change can be presented in many forms: We can use the concept of change to solve the cable problem by using two sets of given data: (x , y) A : Horizontal(x) = 20 Vertical(y) = 30 (20, 30) B : Horizontal(x) = 40 Vertical(y) = 35 (40, 35)

Using the data for A and B and the definition of rate of change we have: (x , y) A : Horizontal = 20 Vertical = 30 (20, 30) B : Horizontal = 40 Vertical = 35 (40, 35)

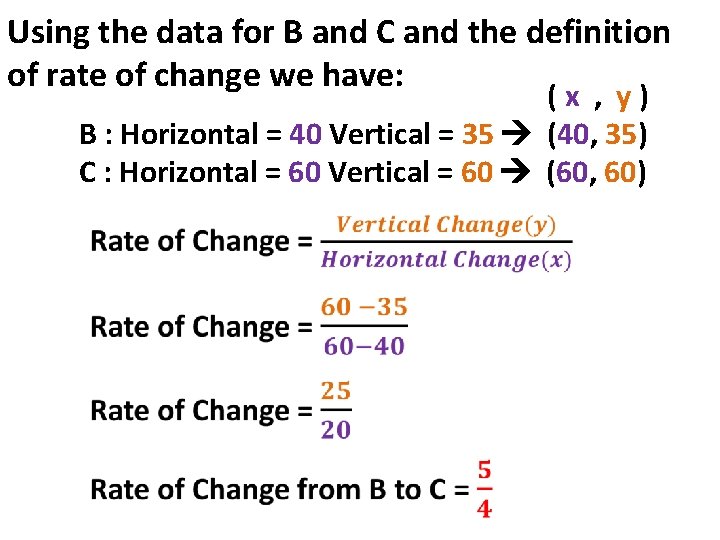
Using the data for B and C and the definition of rate of change we have: (x , y) B : Horizontal = 40 Vertical = 35 (40, 35) C : Horizontal = 60 Vertical = 60 (60, 60)

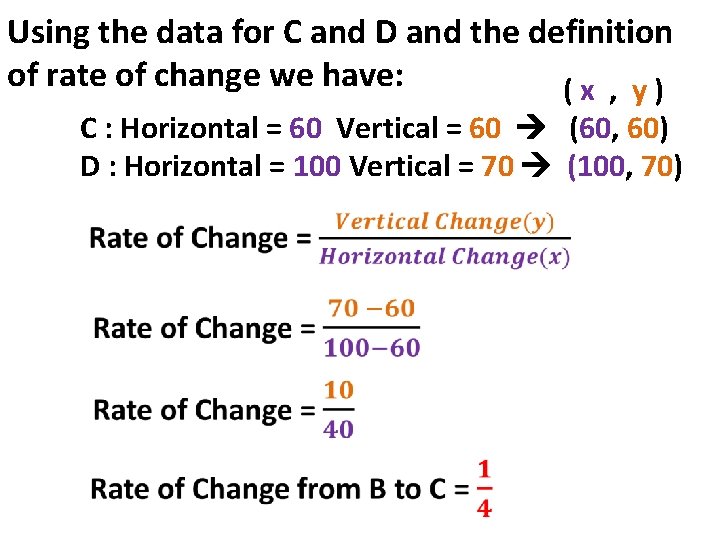
Using the data for C and D and the definition of rate of change we have: (x , y) C : Horizontal = 60 Vertical = 60 (60, 60) D : Horizontal = 100 Vertical = 70 (100, 70)

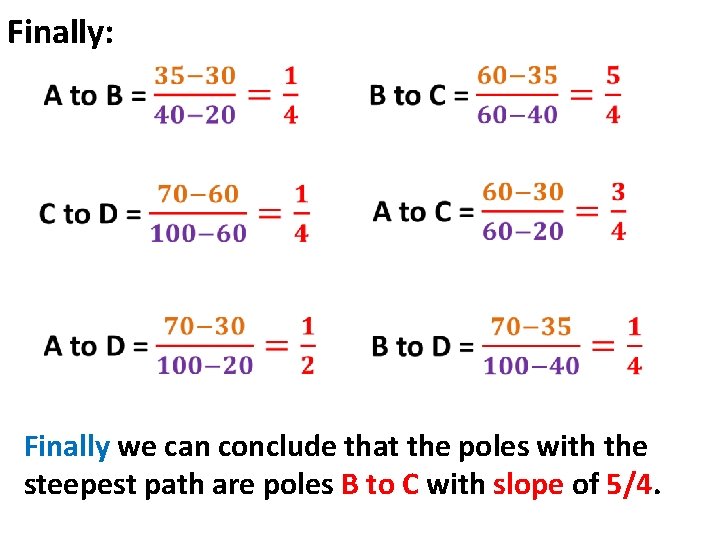
Comparing the slopes of the three: However, we must find all the combination that we can do. Try from A to C, from A to D and from B to C.

Finally: Finally we can conclude that the poles with the steepest path are poles B to C with slope of 5/4.

Class Work: Pages: 295 -297 Problems: 1, 4, 8, 9,

Remember: When we get the same slope, no matter what date points we get, we have a CONSTANT rate of change:

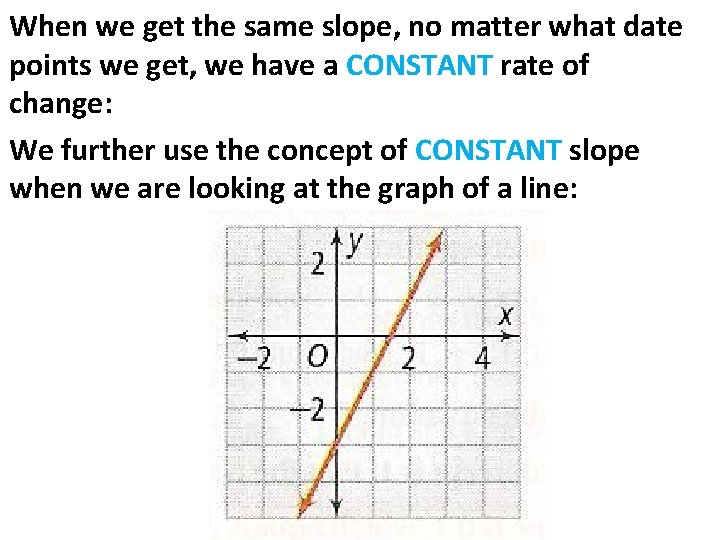
When we get the same slope, no matter what date points we get, we have a CONSTANT rate of change: We further use the concept of CONSTANT slope when we are looking at the graph of a line:

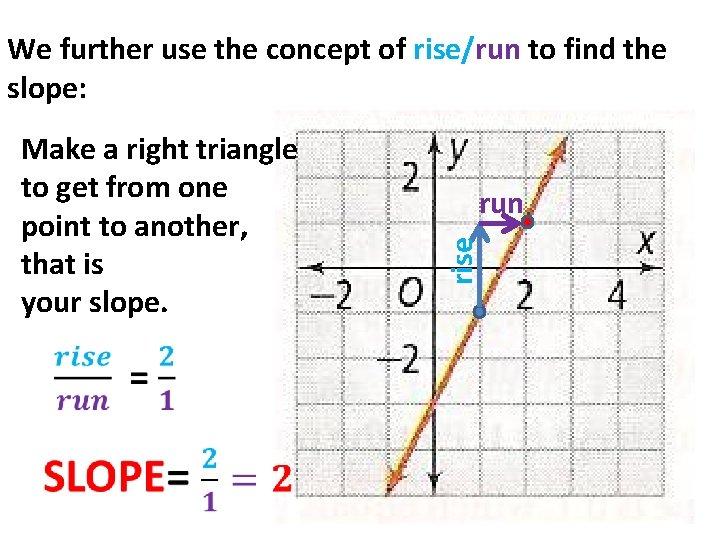
We further use the concept of rise/run to find the slope: run rise Make a right triangle to get from one point to another, that is your slope.

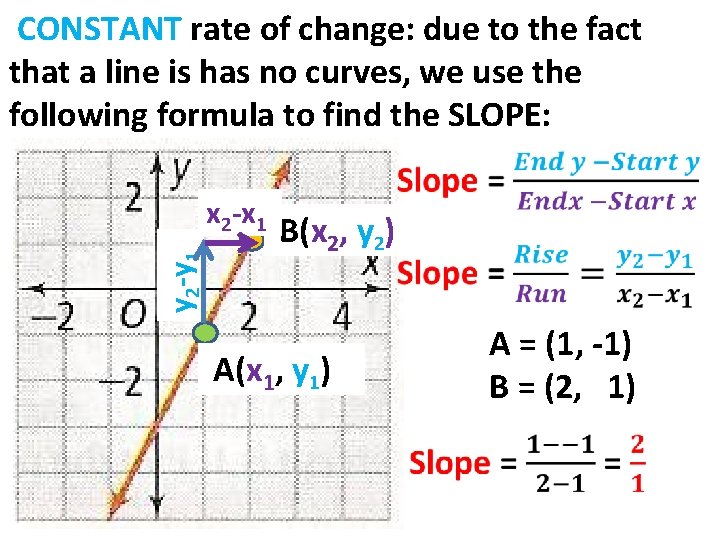
CONSTANT rate of change: due to the fact that a line is has no curves, we use the following formula to find the SLOPE: y 2 -y 1 x 2 -x 1 B(x 2, y 2) A(x 1, y 1) A = (1, -1) B = (2, 1)

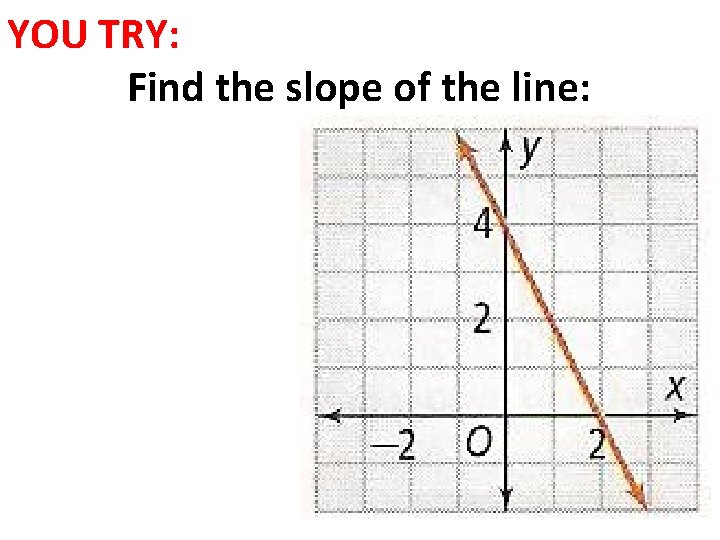
YOU TRY: Find the slope of the line:

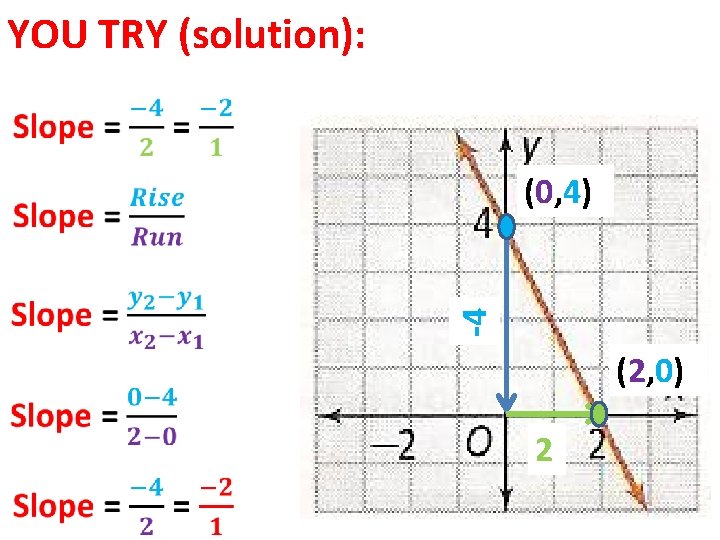
YOU TRY (solution): -4 (0, 4) (2, 0) 2

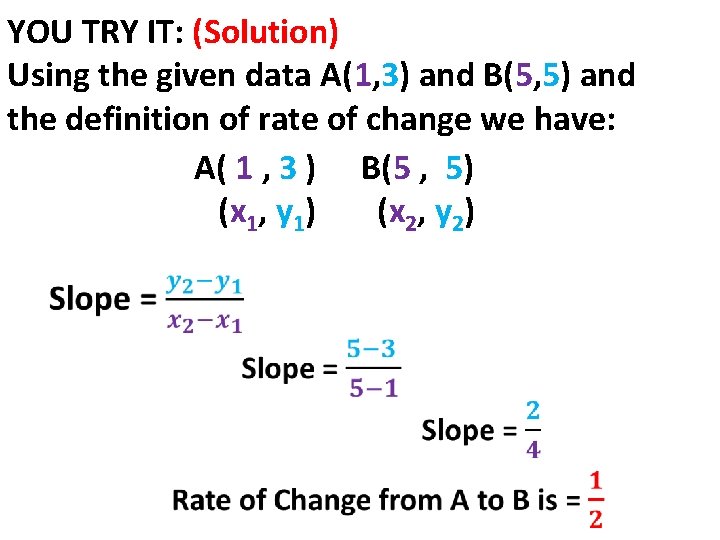
YOU TRY IT: Provide the slope of the line that passes through the points A(1, 3) and B(5, 5):

YOU TRY IT: (Solution) Using the given data A(1, 3) and B(5, 5) and the definition of rate of change we have: A( 1 , 3 ) B(5 , 5) (x 1, y 1) (x 2, y 2)

YOU TRY: Find the slope of the line:

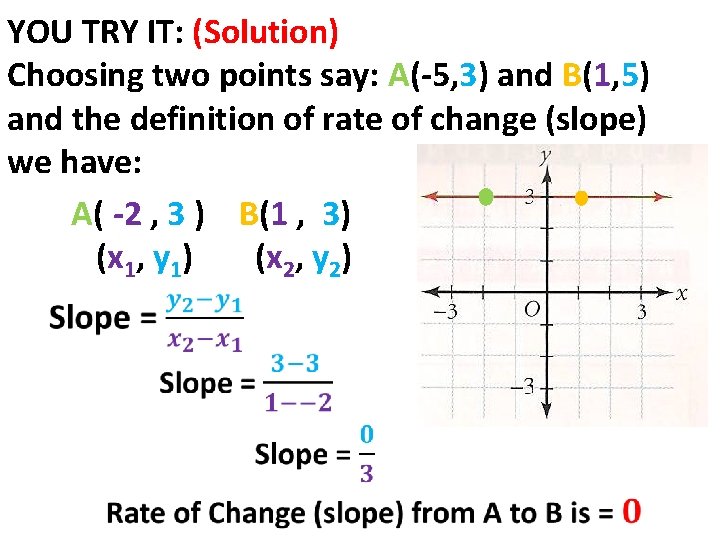
YOU TRY IT: (Solution) Choosing two points say: A(-5, 3) and B(1, 5) and the definition of rate of change (slope) we have: A( -2 , 3 ) B(1 , 3) (x 1, y 1) (x 2, y 2)

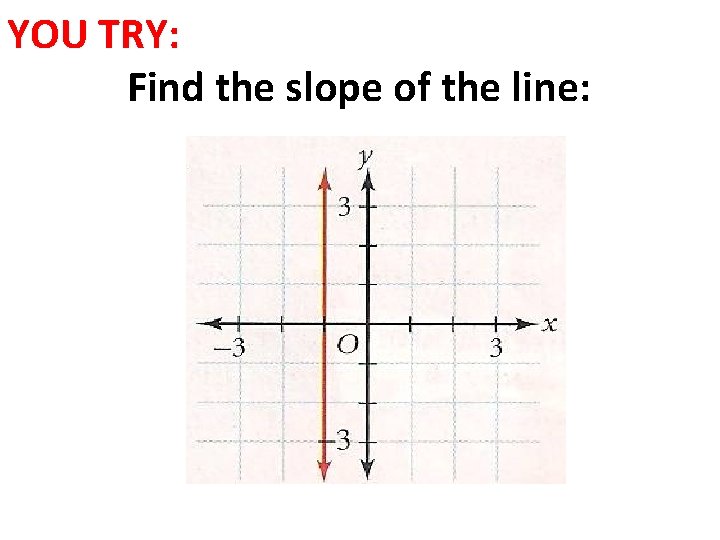
YOU TRY: Find the slope of the line:

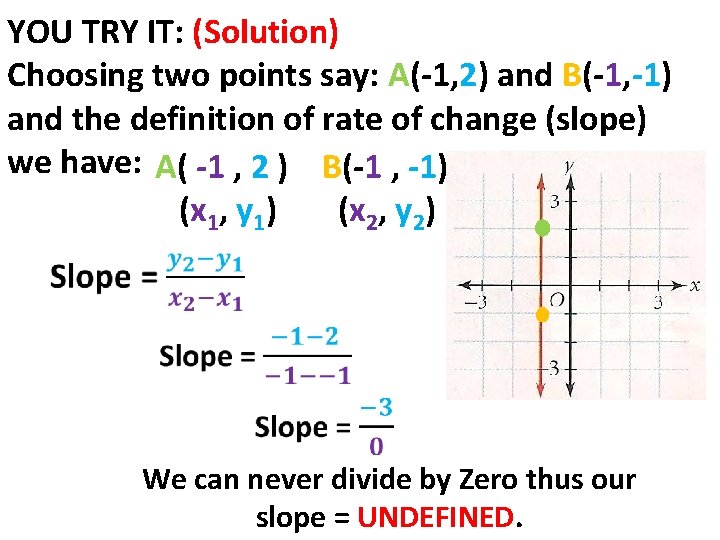
YOU TRY IT: (Solution) Choosing two points say: A(-1, 2) and B(-1, -1) and the definition of rate of change (slope) we have: A( -1 , 2 ) B(-1 , -1) (x 1, y 1) (x 2, y 2) We can never divide by Zero thus our slope = UNDEFINED.

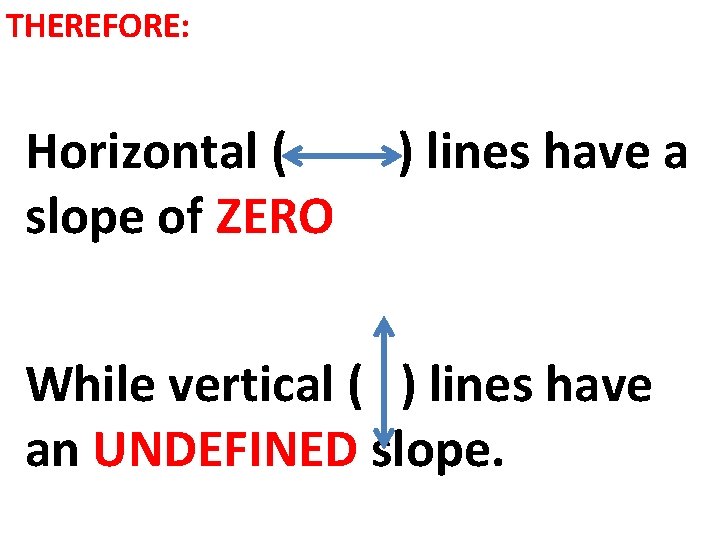
THEREFORE: Horizontal ( slope of ZERO ) lines have a While vertical ( ) lines have an UNDEFINED slope.

VIDEOS: Graphs https: //www. khanacademy. org/math/algebra/line ar-equations-and-inequalitie/slope-andintercepts/v/slope-and-rate-of-change

Class Work: Pages: 295 -297 Problems: As many as needed to master the concept