4 2 Quadratic Equations Objectives Solve quadratic equations

4. 2 Quadratic Equations Objectives: Solve quadratic equations. Use the discriminant to describe the roots of quadratic equations.
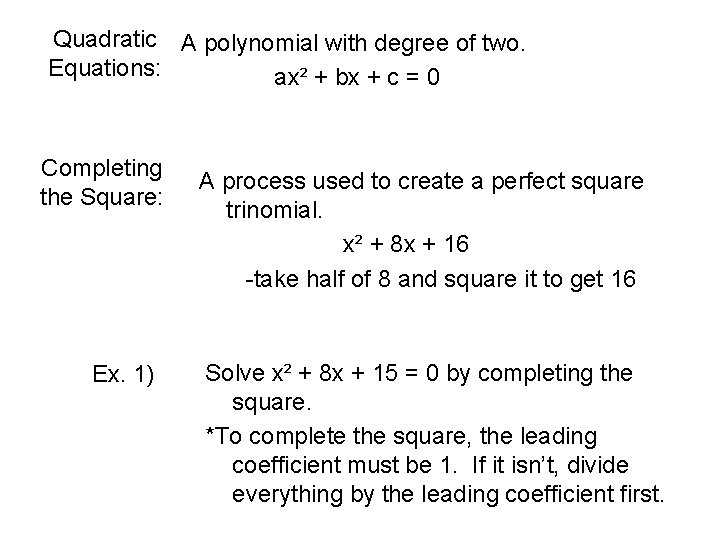
Quadratic A polynomial with degree of two. Equations: ax² + bx + c = 0 Completing the Square: Ex. 1) A process used to create a perfect square trinomial. x² + 8 x + 16 -take half of 8 and square it to get 16 Solve x² + 8 x + 15 = 0 by completing the square. *To complete the square, the leading coefficient must be 1. If it isn’t, divide everything by the leading coefficient first.
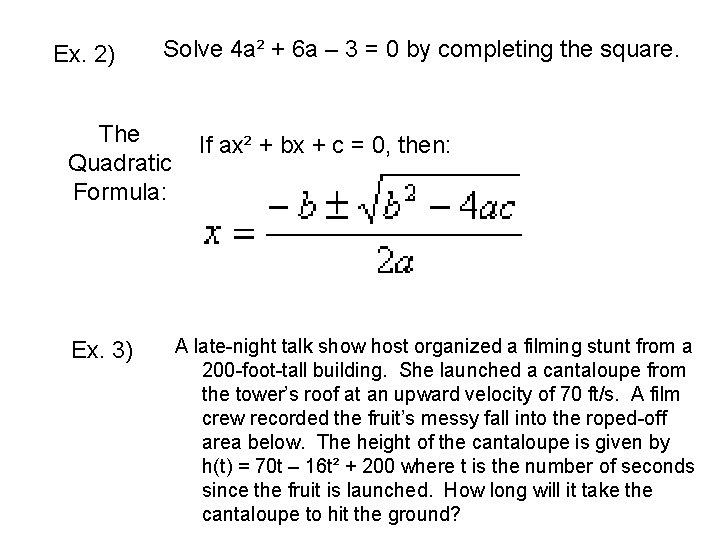
Ex. 2) Solve 4 a² + 6 a – 3 = 0 by completing the square. The Quadratic Formula: Ex. 3) If ax² + bx + c = 0, then: A late-night talk show host organized a filming stunt from a 200 -foot-tall building. She launched a cantaloupe from the tower’s roof at an upward velocity of 70 ft/s. A film crew recorded the fruit’s messy fall into the roped-off area below. The height of the cantaloupe is given by h(t) = 70 t – 16 t² + 200 where t is the number of seconds since the fruit is launched. How long will it take the cantaloupe to hit the ground?

Discriminant: Ex. 4) Conjugates: Ex. 5) b² - 4 ac → shows the nature of the roots. b² - 4 ac > 0 → 2 real roots b² - 4 ac = 0 → exactly one real root b² - 4 ac < 0 → no real roots (2 imaginary roots) Find the discriminant of x² + 2 x – 2 = 0 and describe the nature of the roots of the equation. Then solve the equation by using the Quadratic Formula. a + bi and a - bi Solve 2 x² + 3 x + 5 = 0
- Slides: 4







