2 MULTIPLE INTEGRALS MULTIPLE INTEGRALS In this chapter










![VOLUMES § Thus, we form the subrectangles Rij = [xi– 1, xi] x [yj– VOLUMES § Thus, we form the subrectangles Rij = [xi– 1, xi] x [yj–](https://slidetodoc.com/presentation_image/93618f5b2b5127aca6164f5ffbf534b4/image-15.jpg)









- Slides: 34

2 MULTIPLE INTEGRALS

MULTIPLE INTEGRALS In this chapter, we extend the idea of a definite integral to double and triple integrals of functions of two or three variables.

MULTIPLE INTEGRALS 2. 1 Double Integrals over Rectangles In this section, we will learn about: Double integrals and using them to find volumes.

DOUBLE INTEGRALS OVER RECTANGLES Just as our attempt to solve the area problem led to the definition of a definite integral, we now seek to find the volume of a solid. In the process, we arrive at the definition of a double integral.

DEFINITE INTEGRAL—REVIEW First, let’s recall the basic facts concerning definite integrals of functions of a single variable.

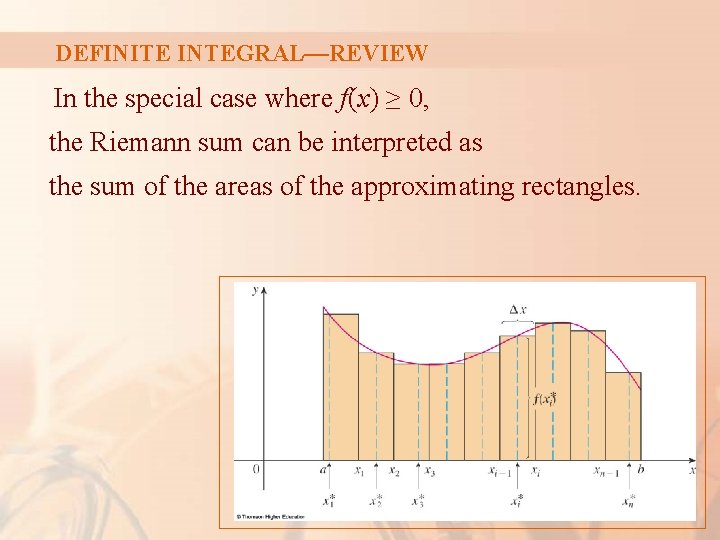
DEFINITE INTEGRAL—REVIEW If f(x) is defined for a ≤ x ≤ b, we start by dividing the interval [a, b] into n subintervals [xi– 1, xi] of equal width ∆x = (b – a)/n. We choose sample points xi* in these subintervals.

DEFINITE INTEGRAL—REVIEW Equation 1 Then, we form the Riemann sum

DEFINITE INTEGRAL—REVIEW Equation 2 Then, we take the limit of such sums as n → ∞ to obtain the definite integral of f from a to b:

DEFINITE INTEGRAL—REVIEW In the special case where f(x) ≥ 0, the Riemann sum can be interpreted as the sum of the areas of the approximating rectangles.

DEFINITE INTEGRAL—REVIEW Then, represents the area under the curve y = f(x) from a to b.

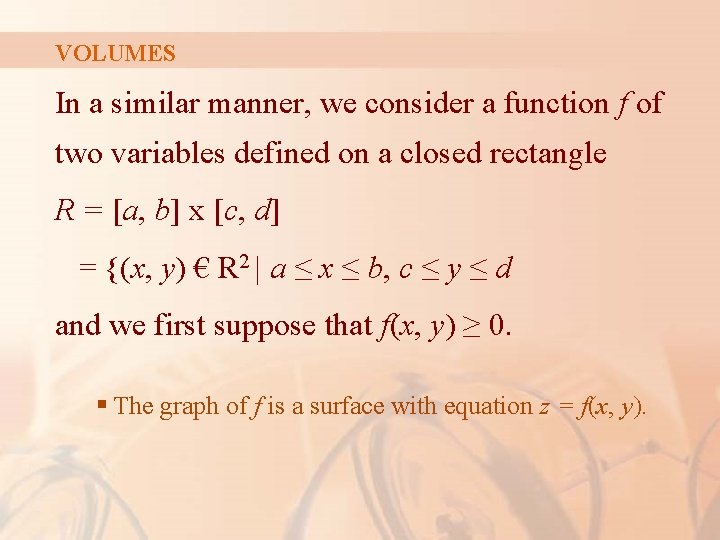
VOLUMES In a similar manner, we consider a function f of two variables defined on a closed rectangle R = [a, b] x [c, d] = {(x, y) € R 2 | a ≤ x ≤ b, c ≤ y ≤ d and we first suppose that f(x, y) ≥ 0. § The graph of f is a surface with equation z = f(x, y).

VOLUMES Let S be the solid that lies above R and under the graph of f, that is, S = {(x, y, z) �R 3 | 0 ≤ z ≤ f(x, y), (x, y) �R} Our goal is to find the volume of S.

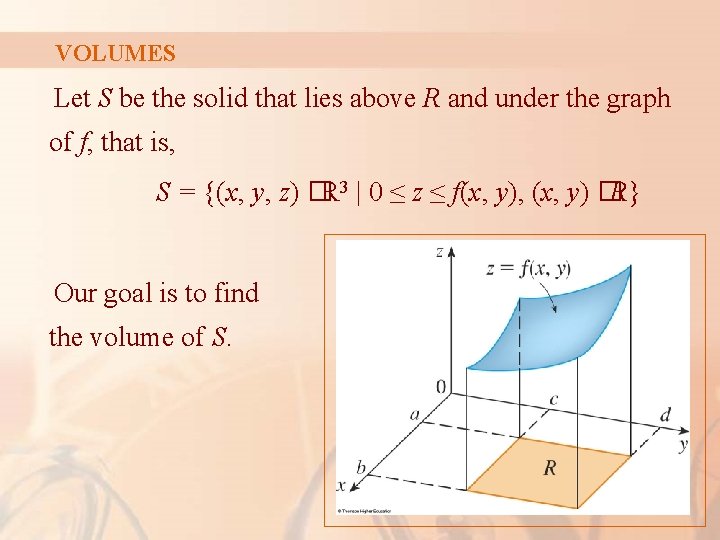
VOLUMES The first step is to divide the rectangle R into subrectangles. § We divide the interval [a, b] into m subintervals [xi– 1, xi] of equal width ∆x = (b – a)/m. § Then, we divide [c, d] into n subintervals [yj– 1, yj] of equal width ∆y = (d – c)/n.

VOLUMES § Next, we draw lines parallel to the coordinate axes through the endpoints of these subintervals.
![VOLUMES Thus we form the subrectangles Rij xi 1 xi x yj VOLUMES § Thus, we form the subrectangles Rij = [xi– 1, xi] x [yj–](https://slidetodoc.com/presentation_image/93618f5b2b5127aca6164f5ffbf534b4/image-15.jpg)
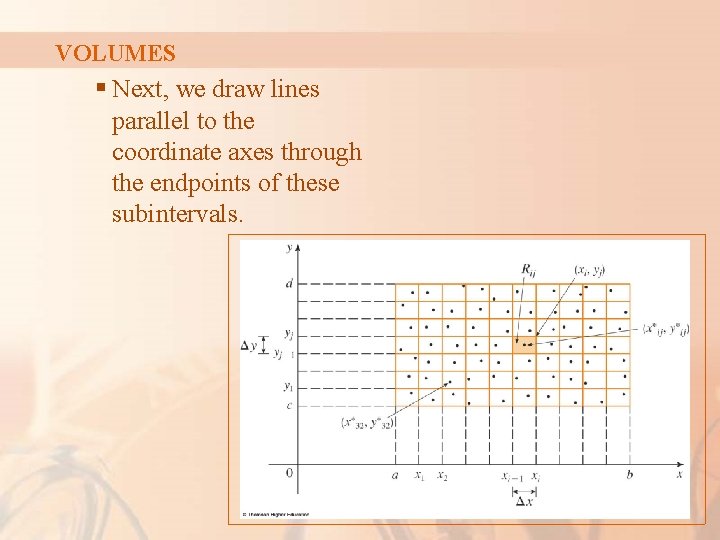
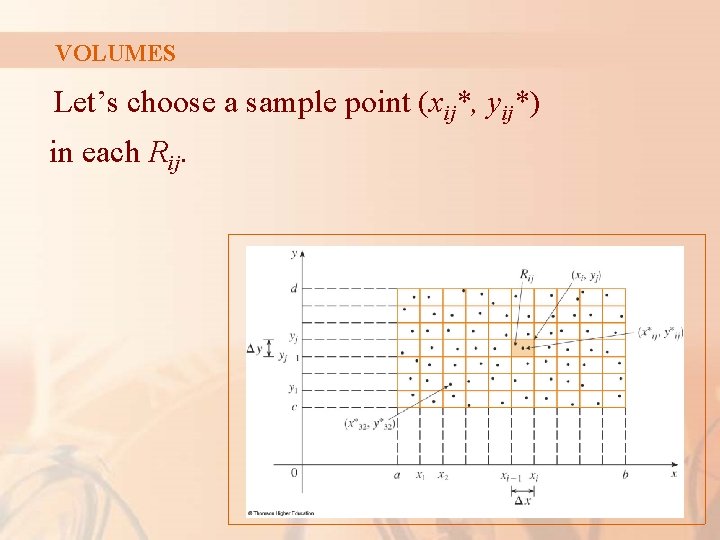
VOLUMES § Thus, we form the subrectangles Rij = [xi– 1, xi] x [yj– 1, yj] = {(x, y) | xi– 1 ≤ xi, yj– 1 ≤ yj} each with area ∆A = ∆x ∆y

VOLUMES Let’s choose a sample point (xij*, yij*) in each Rij.

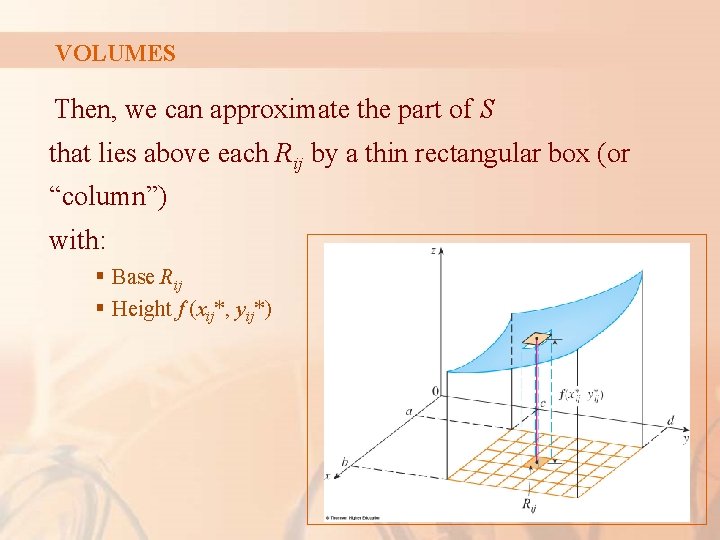
VOLUMES Then, we can approximate the part of S that lies above each Rij by a thin rectangular box (or “column”) with: § Base Rij § Height f (xij*, yij*)

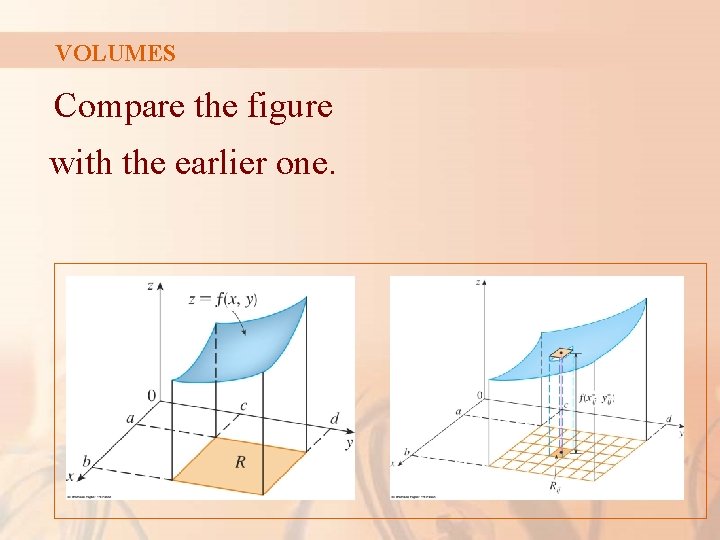
VOLUMES Compare the figure with the earlier one.

VOLUMES The volume of this box is the height of the box times the area of the base rectangle: f(xij *, yij *) ∆A

VOLUMES We follow this procedure for all the rectangles and add the volumes of the corresponding boxes.

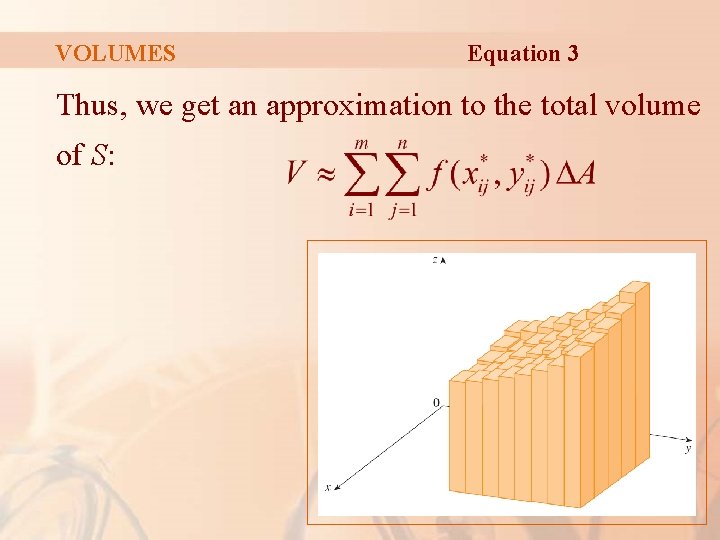
VOLUMES Equation 3 Thus, we get an approximation to the total volume of S:

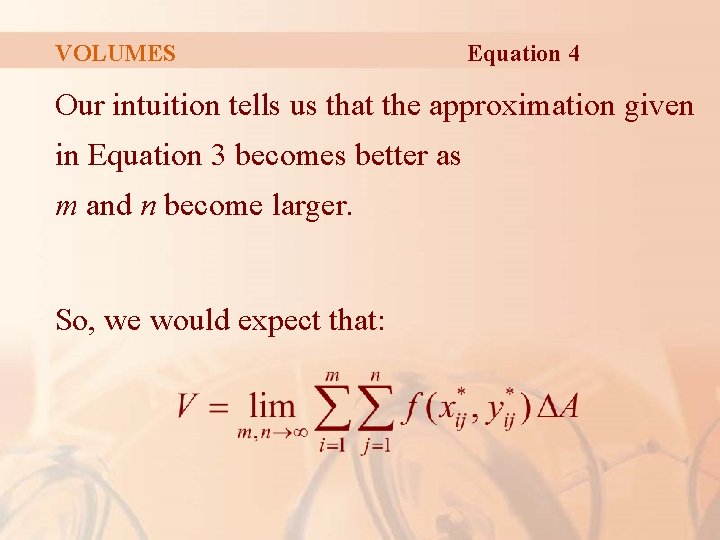
VOLUMES Equation 4 Our intuition tells us that the approximation given in Equation 3 becomes better as m and n become larger. So, we would expect that:

VOLUMES We use the expression in Equation 4 to define the volume of the solid S that lies under the graph of f and above the rectangle R.

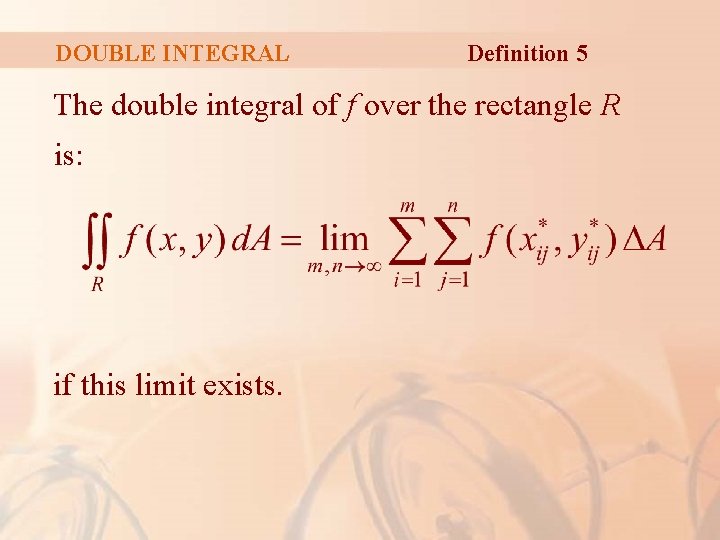
DOUBLE INTEGRAL Definition 5 The double integral of f over the rectangle R is: if this limit exists.

DOUBLE INTEGRAL The sample point (xij*, yij*) can be chosen to be any point in the subrectangle Rij*.

DOUBLE INTEGRAL If f(x, y) ≥ 0, then the volume V of the solid that lies above the rectangle R and below the surface z = f(x, y) is:

DOUBLE REIMANN SUM The sum in Definition 5 is called a double Riemann sum.

DOUBLE INTEGRALS Example 1 Estimate the volume of the solid that lies above the square R = [0, 2] x [0, 2] and below the elliptic paraboloid z = 16 – x 2 – 2 y 2. § Divide R into four equal squares and choose the sample point to be the upper right corner of each square Rij. § Sketch the solid and the approximating rectangular boxes.

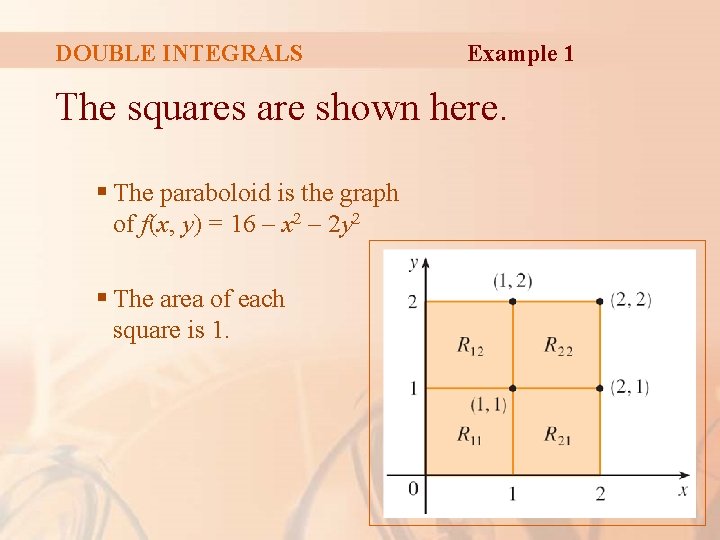
DOUBLE INTEGRALS Example 1 The squares are shown here. § The paraboloid is the graph of f(x, y) = 16 – x 2 – 2 y 2 § The area of each square is 1.

DOUBLE INTEGRALS Example 1 Approximating the volume by the Riemann sum with m = n = 2, we have:

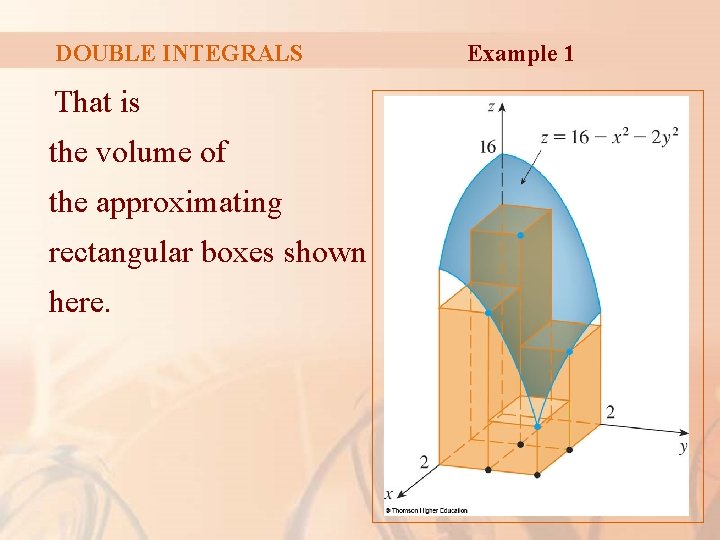
DOUBLE INTEGRALS That is the volume of the approximating rectangular boxes shown here. Example 1

DOUBLE INTEGRALS We get better approximations to the volume in Example 1 if we increase the number of squares.

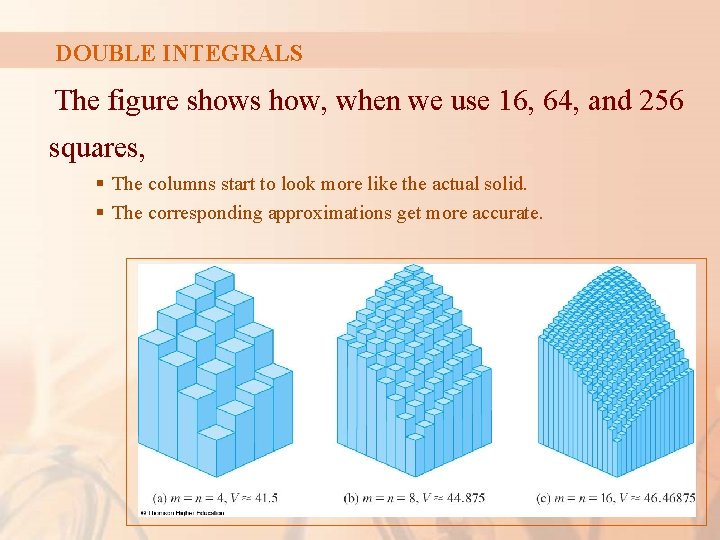
DOUBLE INTEGRALS The figure shows how, when we use 16, 64, and 256 squares, § The columns start to look more like the actual solid. § The corresponding approximations get more accurate.

DOUBLE INTEGRALS In Section 2. 3, we will be able to show that the exact volume is 48.
 Change of variables multiple integrals
Change of variables multiple integrals Calculus chapter 5 integrals
Calculus chapter 5 integrals The substitution rule for definite integrals
The substitution rule for definite integrals Calculus theorems
Calculus theorems Definite integral sigma notation
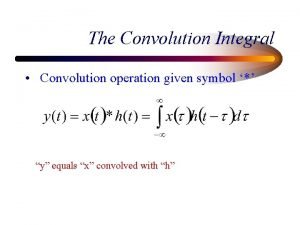
Definite integral sigma notation Circular convolution symbol
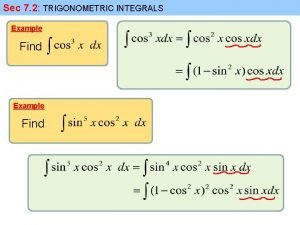
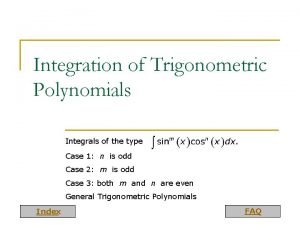
Circular convolution symbol Trigonemtric integrals
Trigonemtric integrals Definite integral vs indefinite integral
Definite integral vs indefinite integral Properties of indefinite integrals
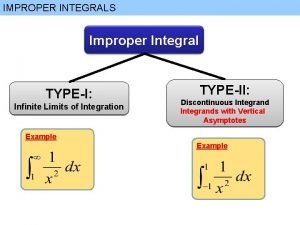
Properties of indefinite integrals Integral
Integral Integral trig identities
Integral trig identities Chain rule integration
Chain rule integration Integral permukaan adalah
Integral permukaan adalah Slidetodoc. com
Slidetodoc. com Notasi integral
Notasi integral Duality theorem in antenna
Duality theorem in antenna Calculate rate of change
Calculate rate of change Maurits haverkort
Maurits haverkort Application of residue theorem to evaluate real integrals
Application of residue theorem to evaluate real integrals Chain rule integrals
Chain rule integrals Application of residue theorem to evaluate real integrals
Application of residue theorem to evaluate real integrals Peta konsep integral
Peta konsep integral интеграл от арксинуса
интеграл от арксинуса Norm of the partition
Norm of the partition Double integral in polar coordinates
Double integral in polar coordinates Max min inequality definite integrals
Max min inequality definite integrals Fourier series and integrals
Fourier series and integrals Fubini's theorem
Fubini's theorem Chain rule formula u v
Chain rule formula u v Hard trig integrals
Hard trig integrals Double integral over rectangle
Double integral over rectangle Triple integral cylindrical coordinates
Triple integral cylindrical coordinates Exploration 1-3a introduction to definite integrals
Exploration 1-3a introduction to definite integrals Integral laws
Integral laws Surface integrals
Surface integrals