2 column proofs Proofs in geometry must be

2 column proofs Proofs in geometry must be done step by step, and each step must have a justification. These justifications can include the given information, definitions, postulates, theorems, and properties

5 parts of a 2 column proof n n n 1. given statements: the information that is provided 2. prove statement: the statement indicating what is to be proved 3. diagram: a sketch that summarizes the provided information. (Sometimes you will need to draw the sketch yourself based on given information? ) 4. statements: the specific steps that are written in the left-hand column 5. reasons: postulates, theorems, definitions, or properties written in the right hand column, which justify each statement.

Given: Point C is not on line AB Prove: Exactly one plane contains line AB and C n n Statements 1. point C is noncollinear with line AB 2. exactly 1 plane contains points A, B, and C n 3. Exactly 1 plane contains line AB and C n n n Reasons 1. given 2. Through any 3 noncollinear points there exists exactly 1 plane (post. 6) 3. If 2 points lie in a plane, then the line containing the points lies in the plane (post 8)

Given: <1 is complementary to < 2 <3 is complementary to < 2 Prove: <1 is congruent to <3 n n n n n 1. <1 is compl to <2 <3 is compl to <2 2. m<1 + m<2 =90 m<3 + m<2 = 90 3. m<1+ m<2= m<3 + m<2 4. m<1 + m<2 -m<2= m<3+m<2 -m<2 5. m<1 = m<3 6. <1 is congruent to <3 n 1. given n 2. n 3. n 4. n 5. 6. n

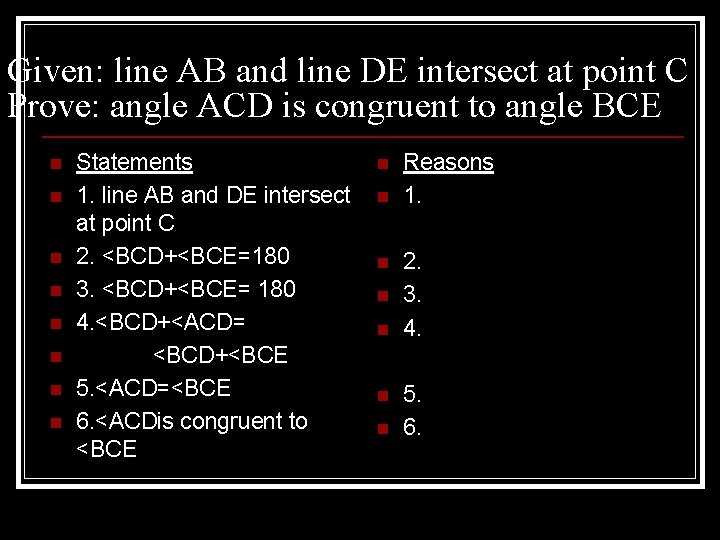
Given: line AB and line DE intersect at point C Prove: angle ACD is congruent to angle BCE n n n n Statements 1. line AB and DE intersect at point C 2. <BCD+<BCE=180 3. <BCD+<BCE= 180 4. <BCD+<ACD= <BCD+<BCE 5. <ACD=<BCE 6. <ACDis congruent to <BCE n n n n Reasons 1. 2. 3. 4. 5. 6.