2 7 Solving Quadratic Inequalities Warm Up Lesson

2 -7 Solving Quadratic Inequalities Warm Up Lesson Presentation Lesson Quiz Holt Mc. Dougal Algebra 2 Algebra 22 Holt Mc. Dougal

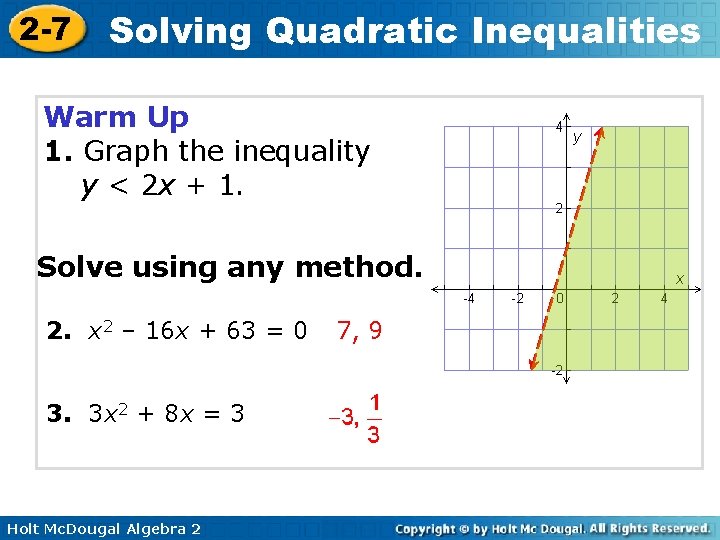
2 -7 Solving Quadratic Inequalities Warm Up 1. Graph the inequality y < 2 x + 1. Solve using any method. 2. x 2 – 16 x + 63 = 0 3. 3 x 2 + 8 x = 3 Holt Mc. Dougal Algebra 2 7, 9

2 -7 Solving Quadratic Inequalities Objectives Solve quadratic inequalities by using tables and graphs. Solve quadratic inequalities by using algebra. Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Vocabulary quadratic inequality in two variables Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Many business profits can be modeled by quadratic functions. To ensure that the profit is above a certain level, financial planners may need to graph and solve quadratic inequalities. A quadratic inequality in two variables can be written in one of the following forms, where a, b, and c are real numbers and a ≠ 0. Its solution set is a set of ordered pairs (x, y). Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities y < ax 2 + bx + c y > ax 2 + bx + c y ≤ ax 2 + bx + c y ≥ ax 2 + bx + c In Lesson 2 -5, you solved linear inequalities in two variables by graphing. You can use a similar procedure to graph quadratic inequalities. Holt Mc. Dougal Algebra 2

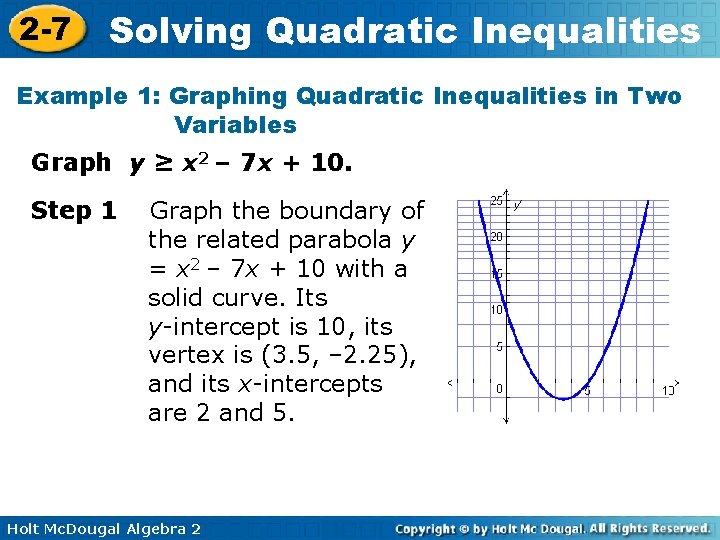
2 -7 Solving Quadratic Inequalities Example 1: Graphing Quadratic Inequalities in Two Variables Graph y ≥ x 2 – 7 x + 10. Step 1 Graph the boundary of the related parabola y = x 2 – 7 x + 10 with a solid curve. Its y-intercept is 10, its vertex is (3. 5, – 2. 25), and its x-intercepts are 2 and 5. Holt Mc. Dougal Algebra 2

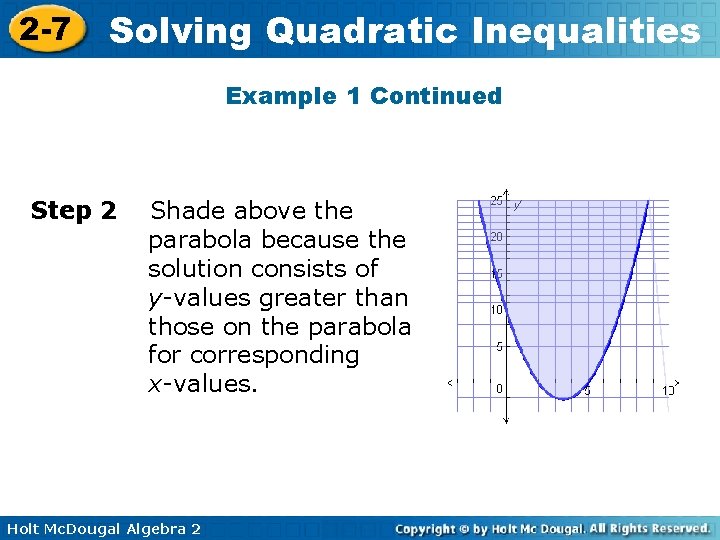
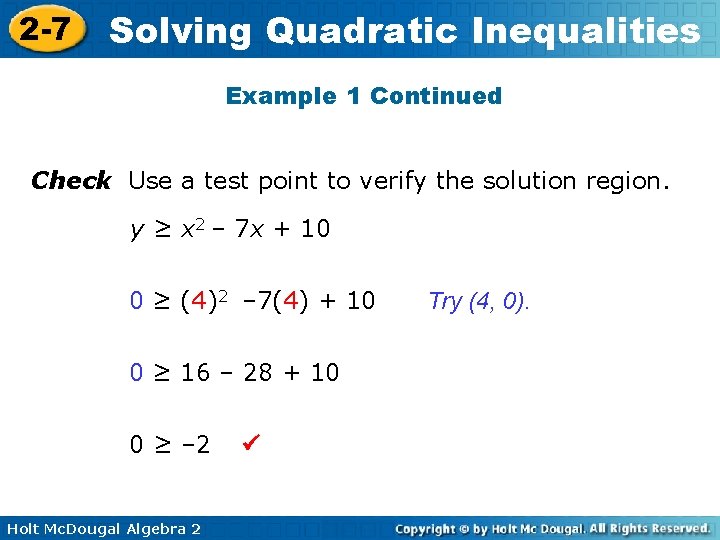
2 -7 Solving Quadratic Inequalities Example 1 Continued Step 2 Shade above the parabola because the solution consists of y-values greater than those on the parabola for corresponding x-values. Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Example 1 Continued Check Use a test point to verify the solution region. y ≥ x 2 – 7 x + 10 0 ≥ (4)2 – 7(4) + 10 0 ≥ 16 – 28 + 10 0 ≥ – 2 Holt Mc. Dougal Algebra 2 Try (4, 0).

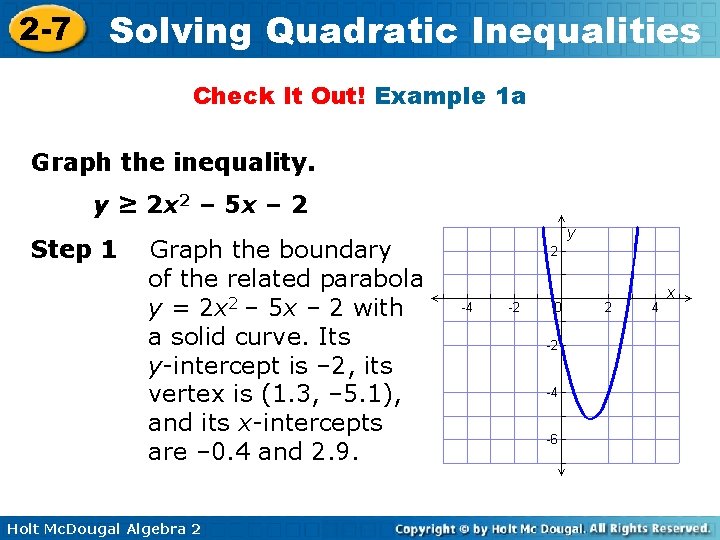
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 a Graph the inequality. y ≥ 2 x 2 – 5 x – 2 Step 1 Graph the boundary of the related parabola y = 2 x 2 – 5 x – 2 with a solid curve. Its y-intercept is – 2, its vertex is (1. 3, – 5. 1), and its x-intercepts are – 0. 4 and 2. 9. Holt Mc. Dougal Algebra 2

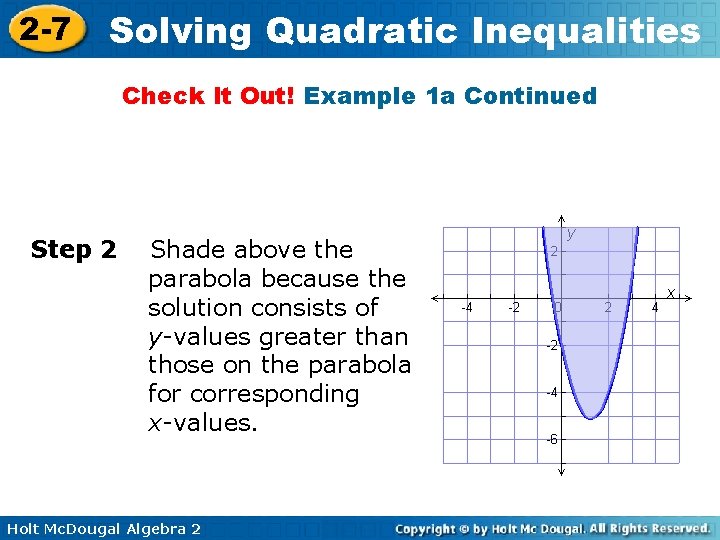
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 a Continued Step 2 Shade above the parabola because the solution consists of y-values greater than those on the parabola for corresponding x-values. Holt Mc. Dougal Algebra 2

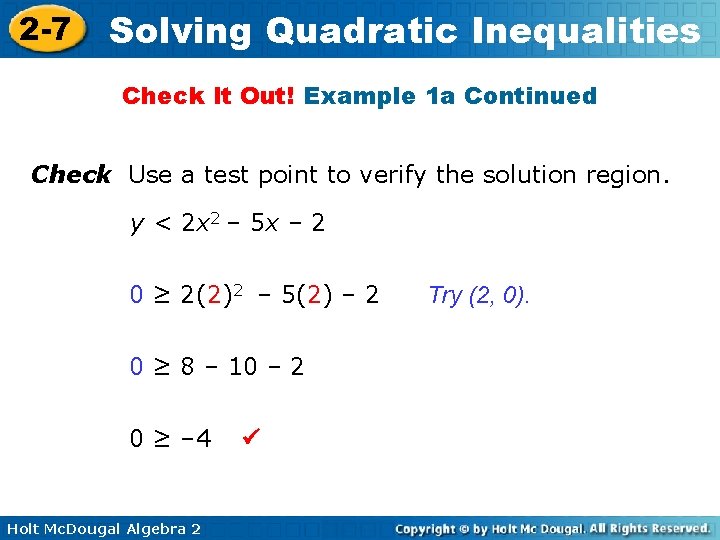
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 a Continued Check Use a test point to verify the solution region. y < 2 x 2 – 5 x – 2 0 ≥ 2(2)2 – 5(2) – 2 0 ≥ 8 – 10 – 2 0 ≥ – 4 Holt Mc. Dougal Algebra 2 Try (2, 0).

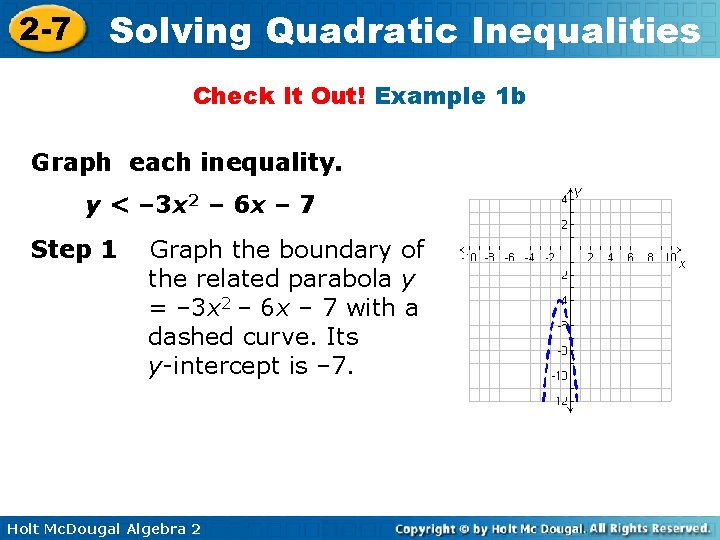
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 b Graph each inequality. y < – 3 x 2 – 6 x – 7 Step 1 Graph the boundary of the related parabola y = – 3 x 2 – 6 x – 7 with a dashed curve. Its y-intercept is – 7. Holt Mc. Dougal Algebra 2

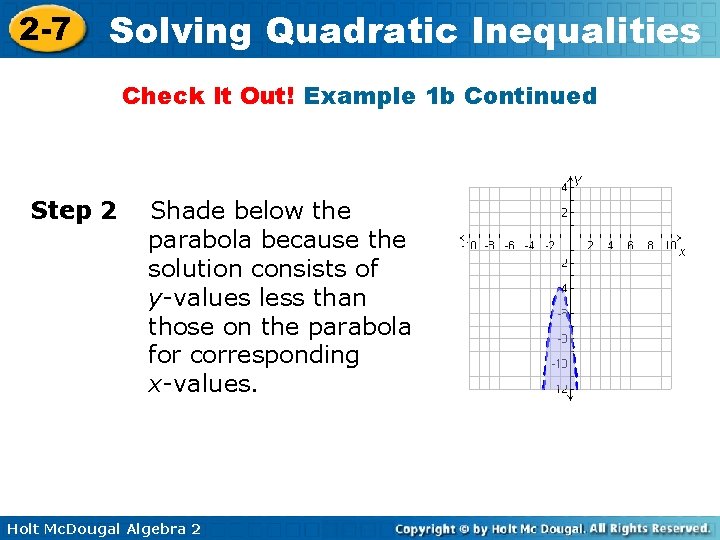
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 b Continued Step 2 Shade below the parabola because the solution consists of y-values less than those on the parabola for corresponding x-values. Holt Mc. Dougal Algebra 2

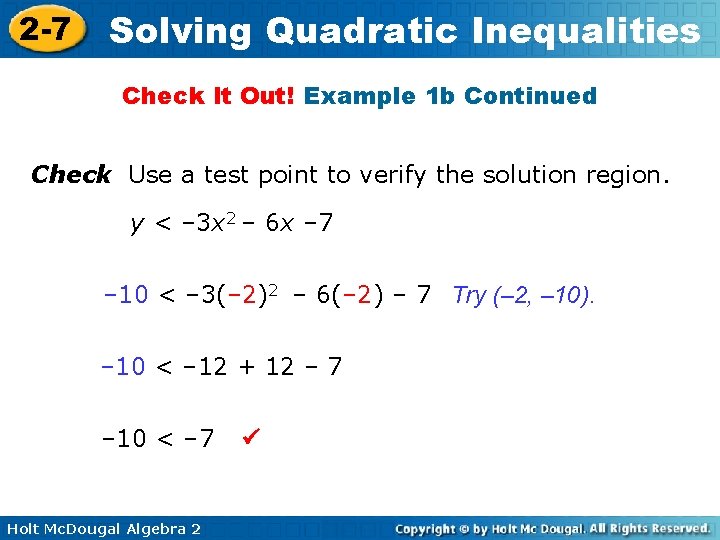
2 -7 Solving Quadratic Inequalities Check It Out! Example 1 b Continued Check Use a test point to verify the solution region. y < – 3 x 2 – 6 x – 7 – 10 < – 3(– 2)2 – 6(– 2) – 7 Try (– 2, – 10). – 10 < – 12 + 12 – 7 – 10 < – 7 Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Quadratic inequalities in one variable, such as ax 2 + bx + c > 0 (a ≠ 0), have solutions in one variable that are graphed on a number line. Reading Math For and statements, both of the conditions must be true. For or statements, at least one of the conditions must be true. Holt Mc. Dougal Algebra 2

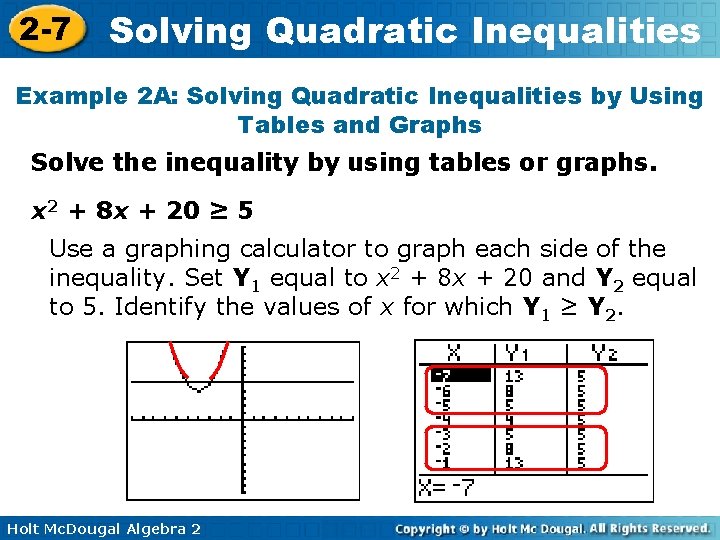
2 -7 Solving Quadratic Inequalities Example 2 A: Solving Quadratic Inequalities by Using Tables and Graphs Solve the inequality by using tables or graphs. x 2 + 8 x + 20 ≥ 5 Use a graphing calculator to graph each side of the inequality. Set Y 1 equal to x 2 + 8 x + 20 and Y 2 equal to 5. Identify the values of x for which Y 1 ≥ Y 2. Holt Mc. Dougal Algebra 2

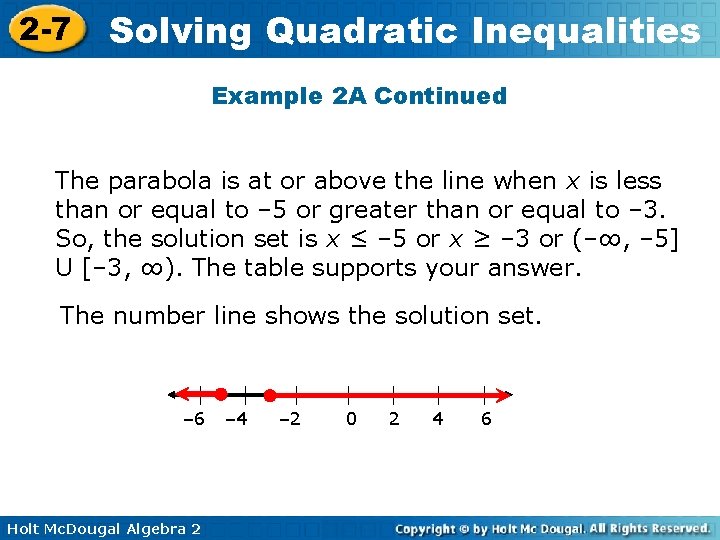
2 -7 Solving Quadratic Inequalities Example 2 A Continued The parabola is at or above the line when x is less than or equal to – 5 or greater than or equal to – 3. So, the solution set is x ≤ – 5 or x ≥ – 3 or (–∞, – 5] U [– 3, ∞). The table supports your answer. The number line shows the solution set. – 6 Holt Mc. Dougal Algebra 2 – 4 – 2 0 2 4 6

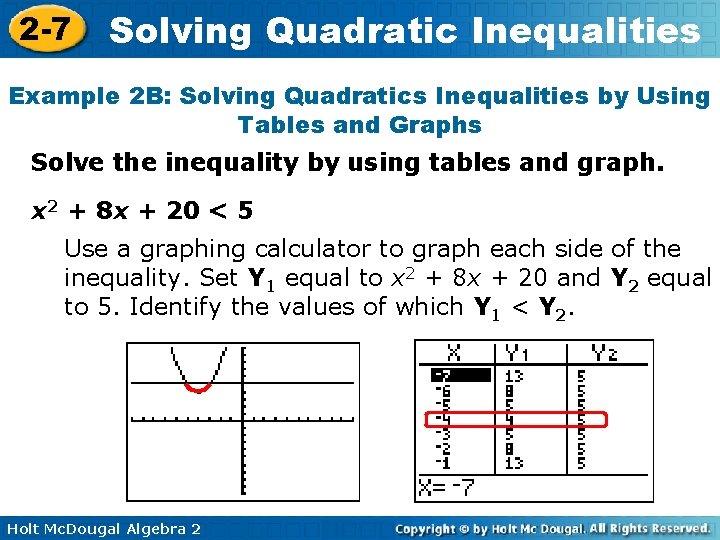
2 -7 Solving Quadratic Inequalities Example 2 B: Solving Quadratics Inequalities by Using Tables and Graphs Solve the inequality by using tables and graph. x 2 + 8 x + 20 < 5 Use a graphing calculator to graph each side of the inequality. Set Y 1 equal to x 2 + 8 x + 20 and Y 2 equal to 5. Identify the values of which Y 1 < Y 2. Holt Mc. Dougal Algebra 2

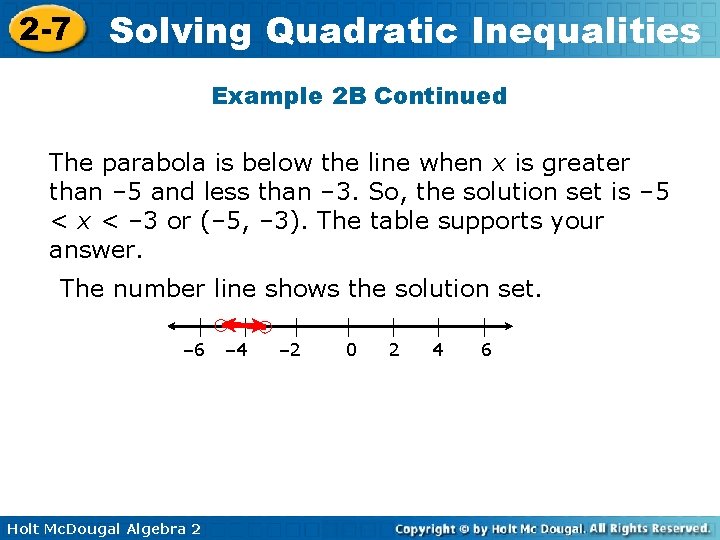
2 -7 Solving Quadratic Inequalities Example 2 B Continued The parabola is below the line when x is greater than – 5 and less than – 3. So, the solution set is – 5 < x < – 3 or (– 5, – 3). The table supports your answer. The number line shows the solution set. – 6 Holt Mc. Dougal Algebra 2 – 4 – 2 0 2 4 6

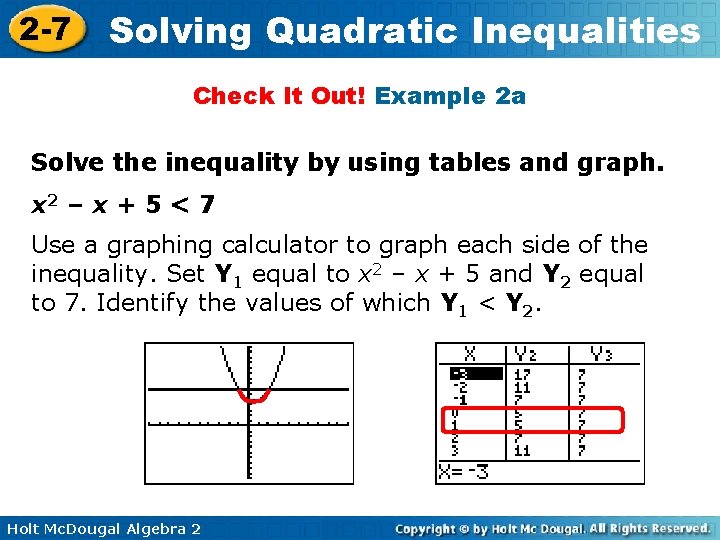
2 -7 Solving Quadratic Inequalities Check It Out! Example 2 a Solve the inequality by using tables and graph. x 2 – x + 5 < 7 Use a graphing calculator to graph each side of the inequality. Set Y 1 equal to x 2 – x + 5 and Y 2 equal to 7. Identify the values of which Y 1 < Y 2. Holt Mc. Dougal Algebra 2

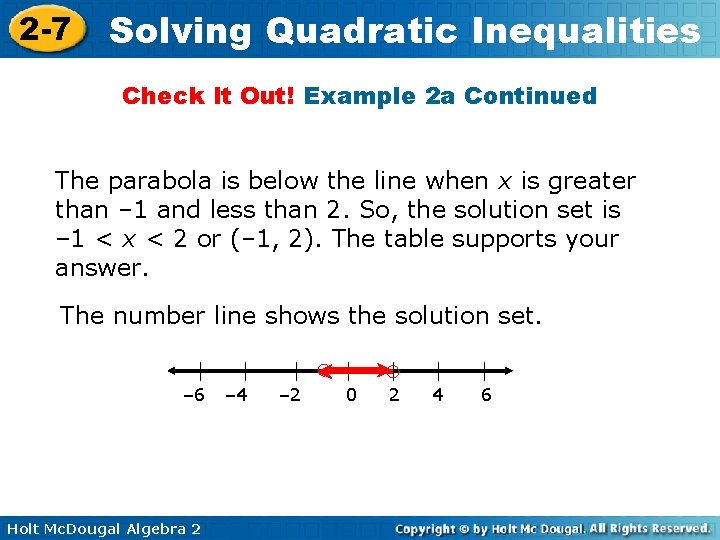
2 -7 Solving Quadratic Inequalities Check It Out! Example 2 a Continued The parabola is below the line when x is greater than – 1 and less than 2. So, the solution set is – 1 < x < 2 or (– 1, 2). The table supports your answer. The number line shows the solution set. – 6 Holt Mc. Dougal Algebra 2 – 4 – 2 0 2 4 6

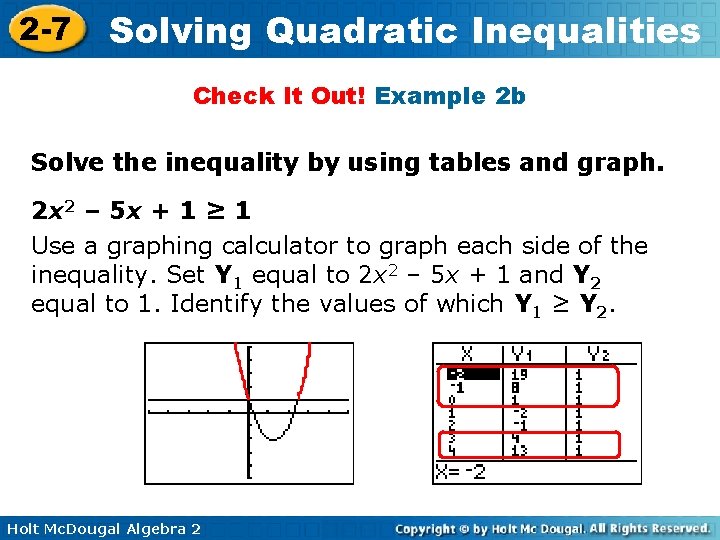
2 -7 Solving Quadratic Inequalities Check It Out! Example 2 b Solve the inequality by using tables and graph. 2 x 2 – 5 x + 1 ≥ 1 Use a graphing calculator to graph each side of the inequality. Set Y 1 equal to 2 x 2 – 5 x + 1 and Y 2 equal to 1. Identify the values of which Y 1 ≥ Y 2. Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Check It Out! Example 2 b Continued The parabola is at or above the line when x is less than or equal to 0 or greater than or equal to 2. 5. So, the solution set is (–∞, 0] U [2. 5, ∞) The number line shows the solution set. – 6 Holt Mc. Dougal Algebra 2 – 4 – 2 0 2 4 6

2 -7 Solving Quadratic Inequalities The number lines showing the solution sets in Example 2 are divided into three distinct regions by the points – 5 and – 3. These points are called critical values. By finding the critical values, you can solve quadratic inequalities algebraically. Holt Mc. Dougal Algebra 2

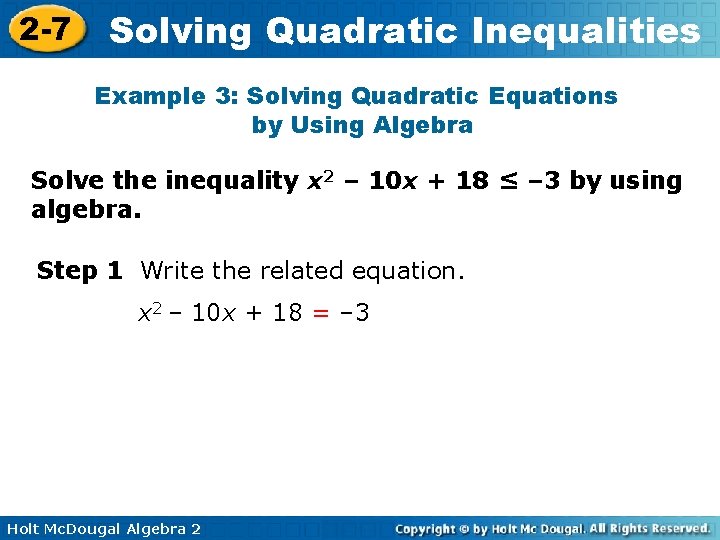
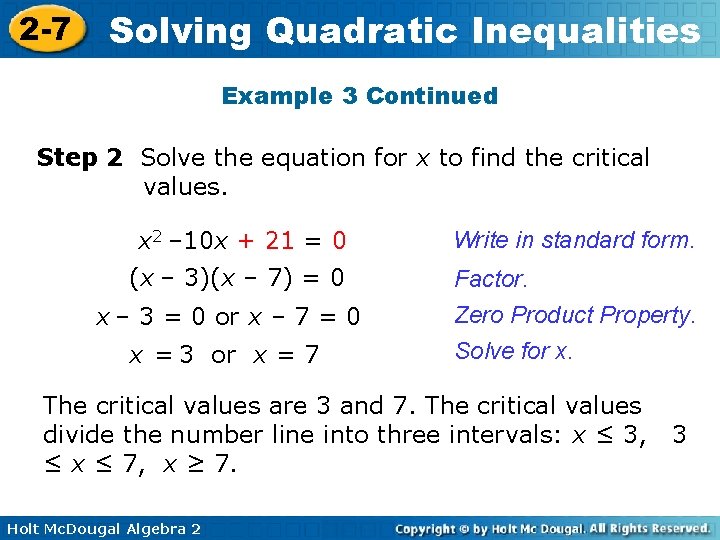
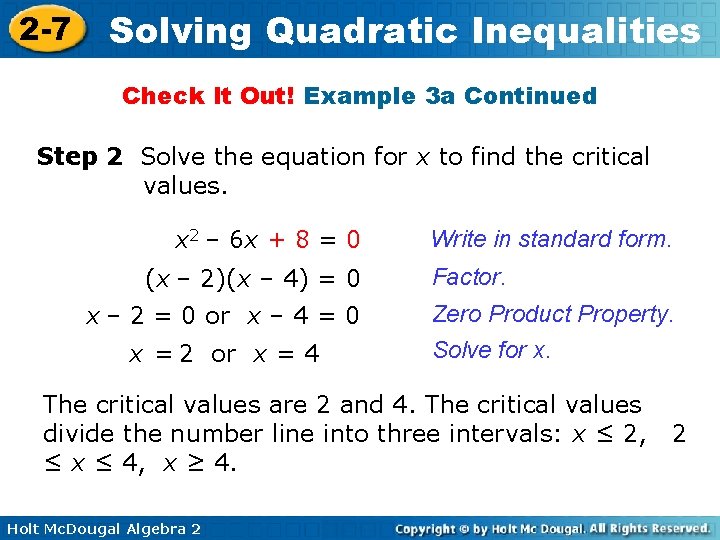
2 -7 Solving Quadratic Inequalities Example 3: Solving Quadratic Equations by Using Algebra Solve the inequality x 2 – 10 x + 18 ≤ – 3 by using algebra. Step 1 Write the related equation. x 2 – 10 x + 18 = – 3 Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Example 3 Continued Step 2 Solve the equation for x to find the critical values. x 2 – 10 x + 21 = 0 Write in standard form. (x – 3)(x – 7) = 0 Factor. Zero Product Property. Solve for x. x – 3 = 0 or x – 7 = 0 x = 3 or x = 7 The critical values are 3 and 7. The critical values divide the number line into three intervals: x ≤ 3, ≤ x ≤ 7, x ≥ 7. Holt Mc. Dougal Algebra 2 3

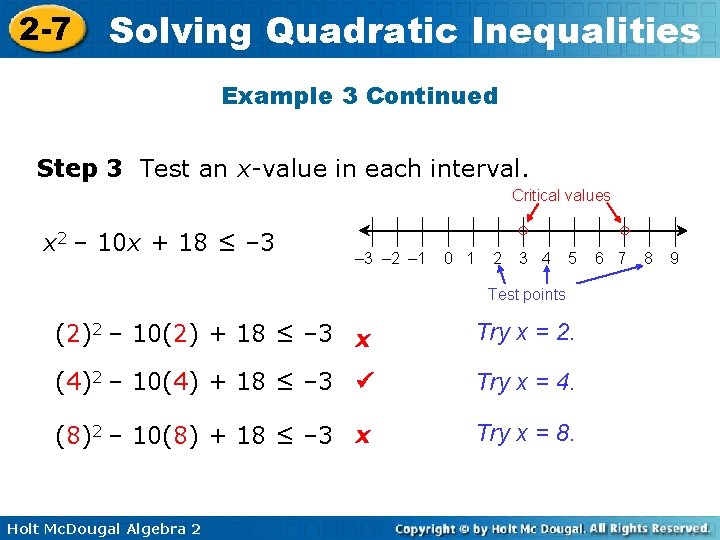
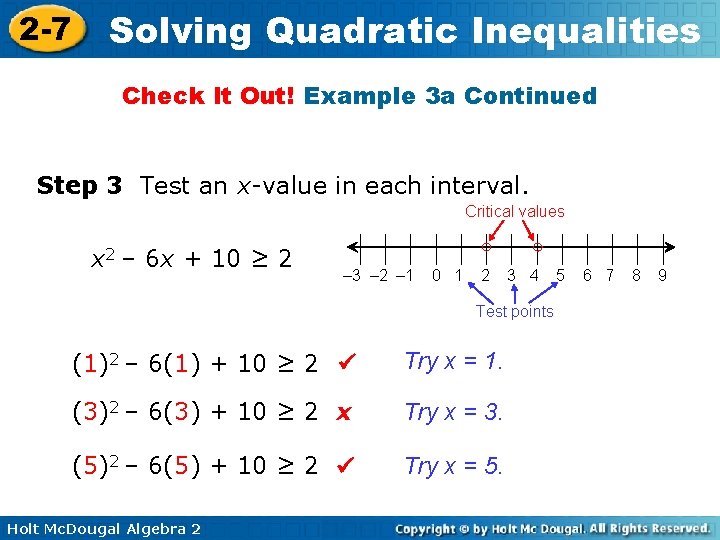
2 -7 Solving Quadratic Inequalities Example 3 Continued Step 3 Test an x-value in each interval. Critical values x 2 – 10 x + 18 ≤ – 3 – 2 – 1 0 1 2 3 4 5 Test points (2)2 – 10(2) + 18 ≤ – 3 x Try x = 2. (4)2 – 10(4) + 18 ≤ – 3 Try x = 4. (8)2 – 10(8) + 18 ≤ – 3 x Try x = 8. Holt Mc. Dougal Algebra 2 6 7 8 9

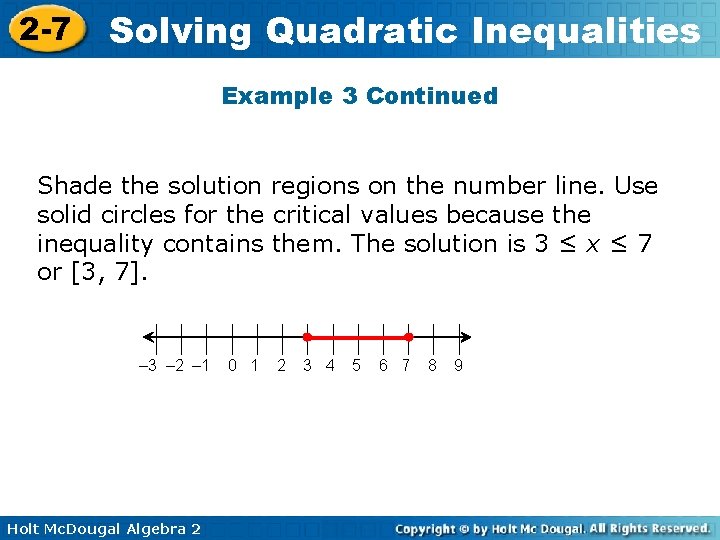
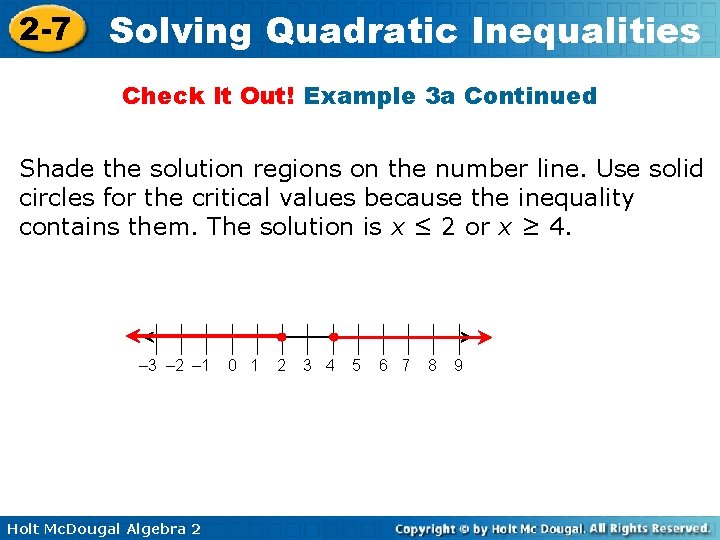
2 -7 Solving Quadratic Inequalities Example 3 Continued Shade the solution regions on the number line. Use solid circles for the critical values because the inequality contains them. The solution is 3 ≤ x ≤ 7 or [3, 7]. – 3 – 2 – 1 Holt Mc. Dougal Algebra 2 0 1 2 3 4 5 6 7 8 9

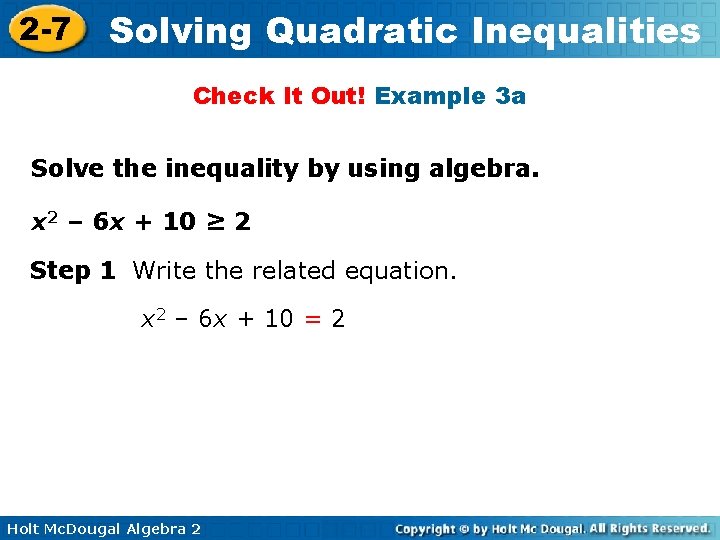
2 -7 Solving Quadratic Inequalities Check It Out! Example 3 a Solve the inequality by using algebra. x 2 – 6 x + 10 ≥ 2 Step 1 Write the related equation. x 2 – 6 x + 10 = 2 Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Check It Out! Example 3 a Continued Step 2 Solve the equation for x to find the critical values. x 2 – 6 x + 8 = 0 (x – 2)(x – 4) = 0 x – 2 = 0 or x – 4 = 0 x = 2 or x = 4 Write in standard form. Factor. Zero Product Property. Solve for x. The critical values are 2 and 4. The critical values divide the number line into three intervals: x ≤ 2, ≤ x ≤ 4, x ≥ 4. Holt Mc. Dougal Algebra 2 2

2 -7 Solving Quadratic Inequalities Check It Out! Example 3 a Continued Step 3 Test an x-value in each interval. Critical values x 2 – 6 x + 10 ≥ 2 – 3 – 2 – 1 0 1 2 3 4 Test points (1)2 – 6(1) + 10 ≥ 2 Try x = 1. (3)2 – 6(3) + 10 ≥ 2 x Try x = 3. (5)2 – 6(5) + 10 ≥ 2 Try x = 5. Holt Mc. Dougal Algebra 2 5 6 7 8 9

2 -7 Solving Quadratic Inequalities Check It Out! Example 3 a Continued Shade the solution regions on the number line. Use solid circles for the critical values because the inequality contains them. The solution is x ≤ 2 or x ≥ 4. – 3 – 2 – 1 Holt Mc. Dougal Algebra 2 0 1 2 3 4 5 6 7 8 9

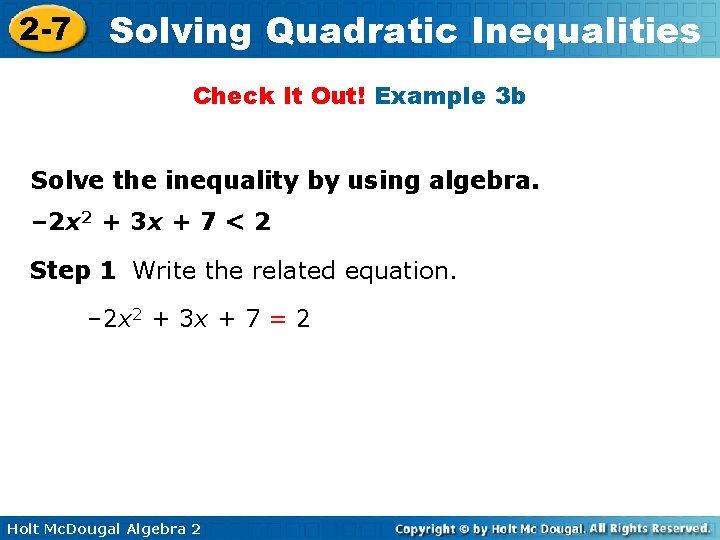
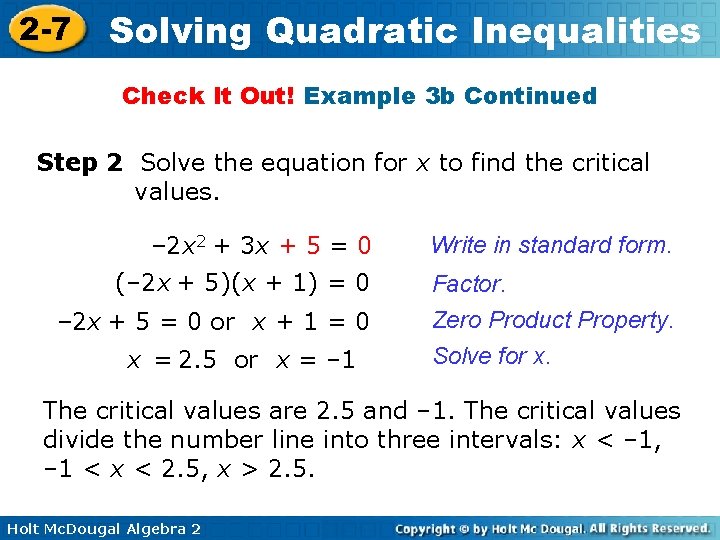
2 -7 Solving Quadratic Inequalities Check It Out! Example 3 b Solve the inequality by using algebra. – 2 x 2 + 3 x + 7 < 2 Step 1 Write the related equation. – 2 x 2 + 3 x + 7 = 2 Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Check It Out! Example 3 b Continued Step 2 Solve the equation for x to find the critical values. – 2 x 2 + 3 x + 5 = 0 Write in standard form. (– 2 x + 5)(x + 1) = 0 Factor. Zero Product Property. Solve for x. – 2 x + 5 = 0 or x + 1 = 0 x = 2. 5 or x = – 1 The critical values are 2. 5 and – 1. The critical values divide the number line into three intervals: x < – 1, – 1 < x < 2. 5, x > 2. 5. Holt Mc. Dougal Algebra 2

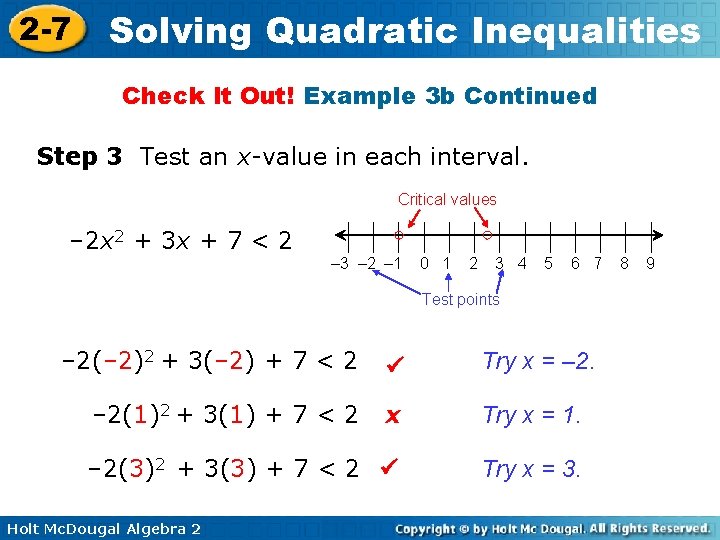
2 -7 Solving Quadratic Inequalities Check It Out! Example 3 b Continued Step 3 Test an x-value in each interval. Critical values – 2 x 2 + 3 x + 7 < 2 – 3 – 2 – 1 0 1 2 3 4 5 6 7 Test points – 2(– 2)2 + 3(– 2) + 7 < 2 Try x = – 2(1)2 + 3(1) + 7 < 2 x Try x = 1. – 2(3)2 + 3(3) + 7 < 2 Try x = 3. Holt Mc. Dougal Algebra 2 8 9

2 -7 Solving Quadratic Inequalities Check It Out! Example 3 Shade the solution regions on the number line. Use open circles for the critical values because the inequality does not contain or equal to. The solution is x < – 1 or x > 2. 5. – 3 – 2 – 1 Holt Mc. Dougal Algebra 2 0 1 2 3 4 5 6 7 8 9

2 -7 Solving Quadratic Inequalities Remember! A compound inequality such as 12 ≤ x ≤ 28 can be written as {x|x ≥ 12 U x ≤ 28}, or x ≥ 12 and x ≤ 28. (see Lesson 2 -8). Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Example 4: Problem-Solving Application The monthly profit P of a small business that sells bicycle helmets can be modeled by the function P(x) = – 8 x 2 + 600 x – 4200, where x is the average selling price of a helmet. What range of selling prices will generate a monthly profit of at least $6000? Holt Mc. Dougal Algebra 2

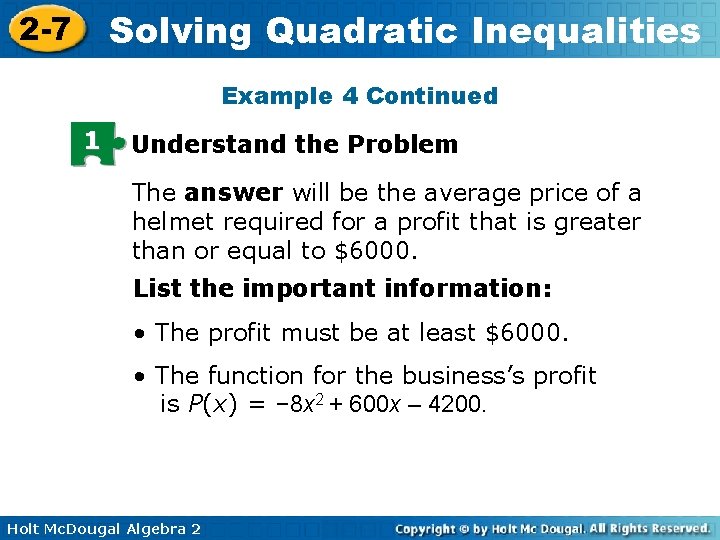
Solving Quadratic Inequalities 2 -7 Example 4 Continued 1 Understand the Problem The answer will be the average price of a helmet required for a profit that is greater than or equal to $6000. List the important information: • The profit must be at least $6000. • The function for the business’s profit is P(x) = – 8 x 2 + 600 x – 4200. Holt Mc. Dougal Algebra 2

Solving Quadratic Inequalities 2 -7 Example 4 Continued 2 Make a Plan Write an inequality showing profit greater than or equal to $6000. Then solve the inequality by using algebra. Holt Mc. Dougal Algebra 2

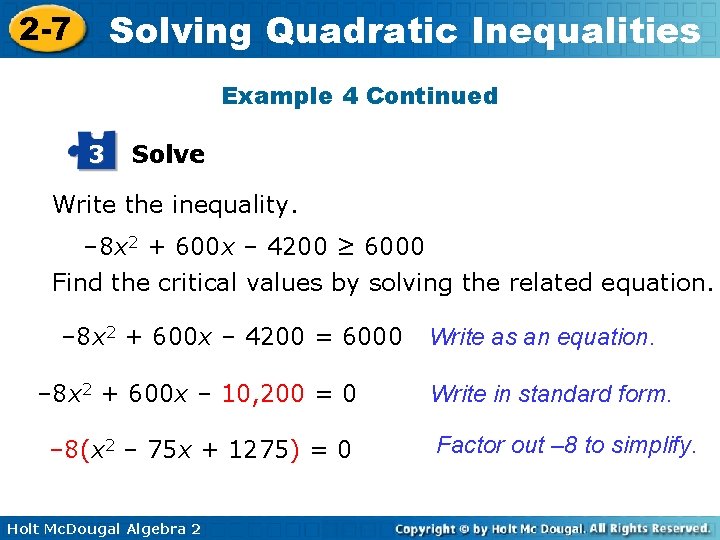
Solving Quadratic Inequalities 2 -7 Example 4 Continued 3 Solve Write the inequality. – 8 x 2 + 600 x – 4200 ≥ 6000 Find the critical values by solving the related equation. – 8 x 2 + 600 x – 4200 = 6000 – 8 x 2 + 600 x – 10, 200 = 0 – 8(x 2 – 75 x + 1275) = 0 Holt Mc. Dougal Algebra 2 Write as an equation. Write in standard form. Factor out – 8 to simplify.

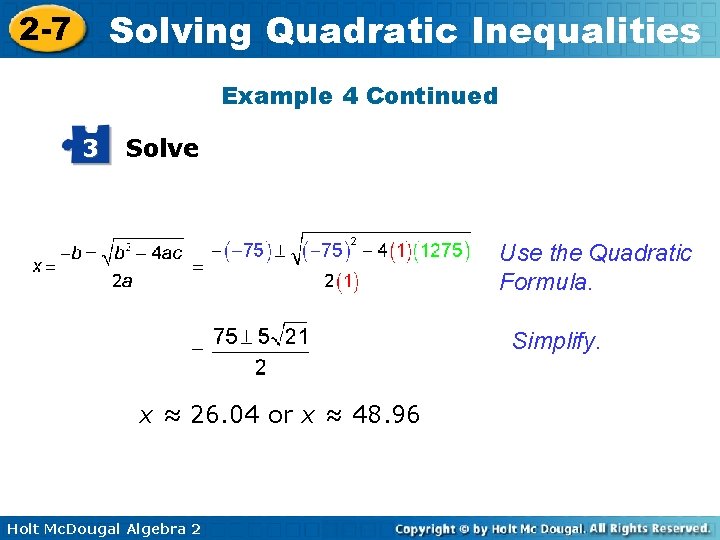
Solving Quadratic Inequalities 2 -7 Example 4 Continued 3 Solve Use the Quadratic Formula. Simplify. x ≈ 26. 04 or x ≈ 48. 96 Holt Mc. Dougal Algebra 2

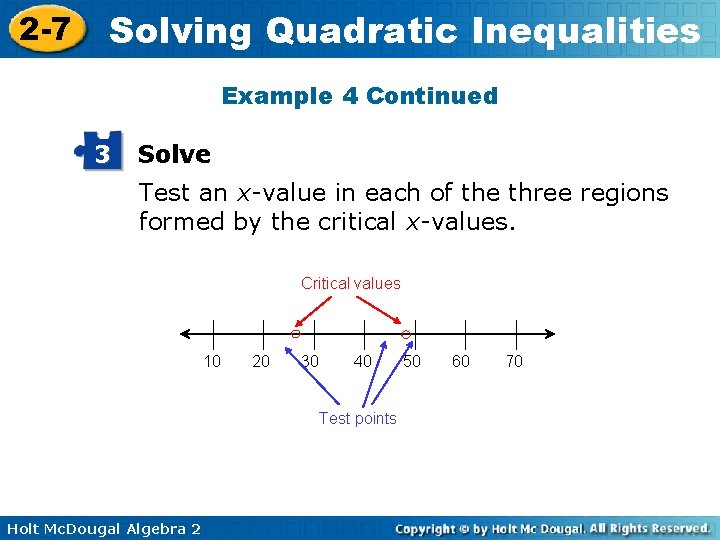
2 -7 Solving Quadratic Inequalities Example 4 Continued 3 Solve Test an x-value in each of the three regions formed by the critical x-values. Critical values 10 20 30 40 Test points Holt Mc. Dougal Algebra 2 50 60 70

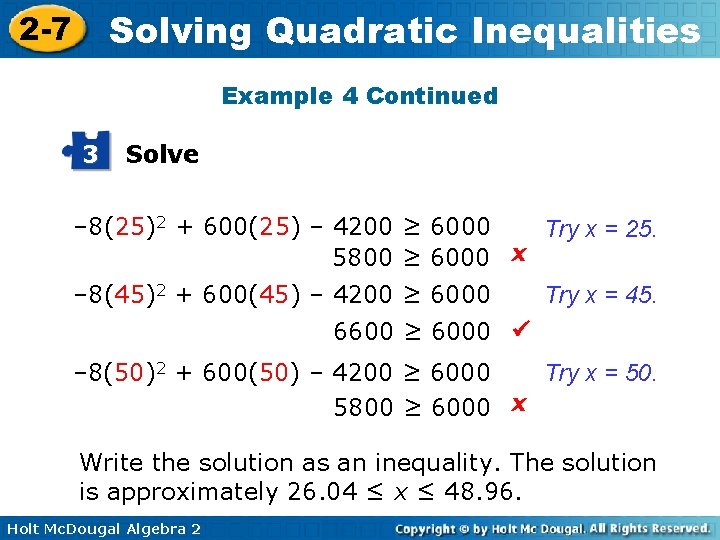
Solving Quadratic Inequalities 2 -7 Example 4 Continued 3 Solve – 8(25)2 + 600(25) – 4200 ≥ 6000 Try x = 25. 5800 ≥ 6000 x – 8(45)2 + 600(45) – 4200 ≥ 6000 Try x = 45. 6600 ≥ 6000 Try x = 50. – 8(50)2 + 600(50) – 4200 ≥ 6000 5800 ≥ 6000 x Write the solution as an inequality. The solution is approximately 26. 04 ≤ x ≤ 48. 96. Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Example 4 Continued 3 Solve For a profit of $6000, the average price of a helmet needs to be between $26. 04 and $48. 96, inclusive. Holt Mc. Dougal Algebra 2

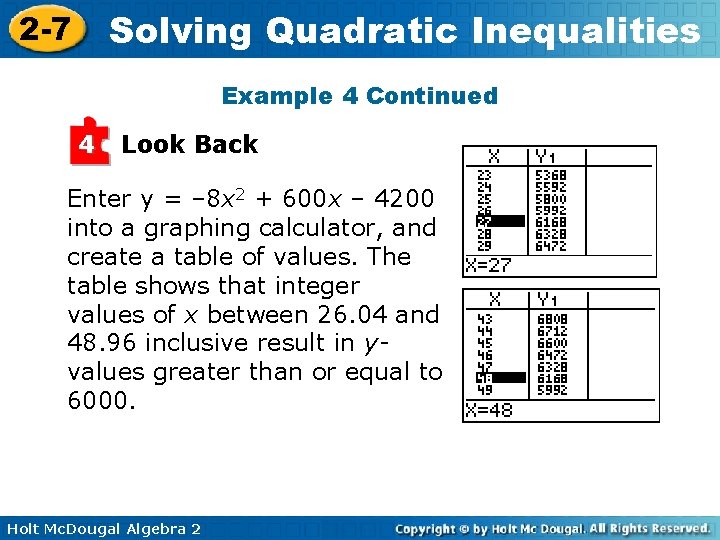
Solving Quadratic Inequalities 2 -7 Example 4 Continued 4 Look Back Enter y = – 8 x 2 + 600 x – 4200 into a graphing calculator, and create a table of values. The table shows that integer values of x between 26. 04 and 48. 96 inclusive result in yvalues greater than or equal to 6000. Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Check It Out! Example 4 A business offers educational tours to Patagonia, a region of South America that includes parts of Chile and Argentina. The profit P for x number of persons is P(x) = – 25 x 2 + 1250 x – 5000. The trip will be rescheduled if the profit is less $7500. How many people must have signed up if the trip is rescheduled? Holt Mc. Dougal Algebra 2

Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 1 Understand the Problem The answer will be the number of people signed up for the trip if the profit is less than $7500. List the important information: • The profit will be less than $7500. • The function for the profit is P(x) = – 25 x 2 + 1250 x – 5000. Holt Mc. Dougal Algebra 2

Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 2 Make a Plan Write an inequality showing profit less than $7500. Then solve the inequality by using algebra. Holt Mc. Dougal Algebra 2

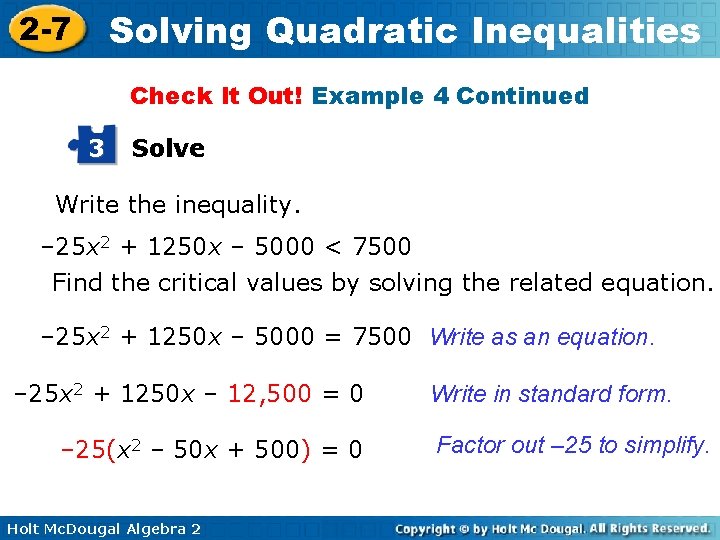
Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 3 Solve Write the inequality. – 25 x 2 + 1250 x – 5000 < 7500 Find the critical values by solving the related equation. – 25 x 2 + 1250 x – 5000 = 7500 Write as an equation. – 25 x 2 + 1250 x – 12, 500 = 0 – 25(x 2 – 50 x + 500) = 0 Holt Mc. Dougal Algebra 2 Write in standard form. Factor out – 25 to simplify.

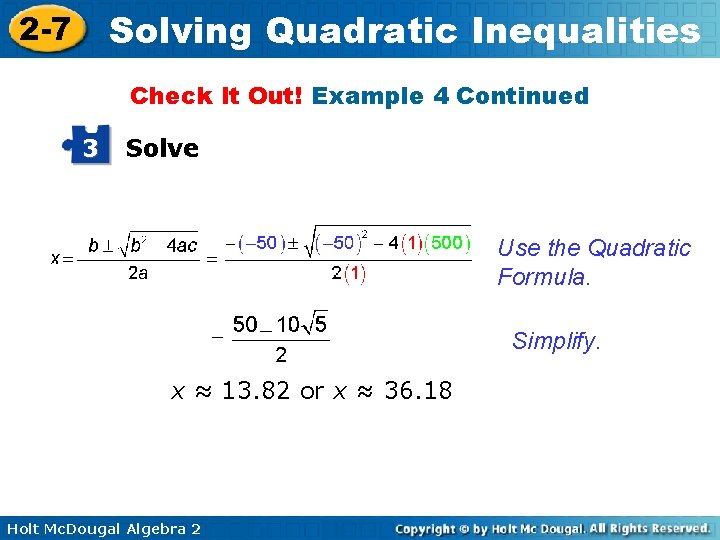
Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 3 Solve Use the Quadratic Formula. Simplify. x ≈ 13. 82 or x ≈ 36. 18 Holt Mc. Dougal Algebra 2

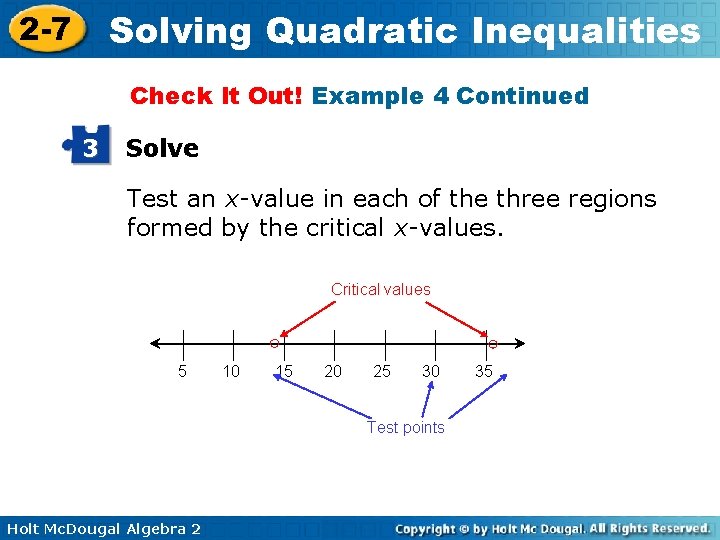
Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 3 Solve Test an x-value in each of the three regions formed by the critical x-values. Critical values 5 10 15 20 25 30 Test points Holt Mc. Dougal Algebra 2 35

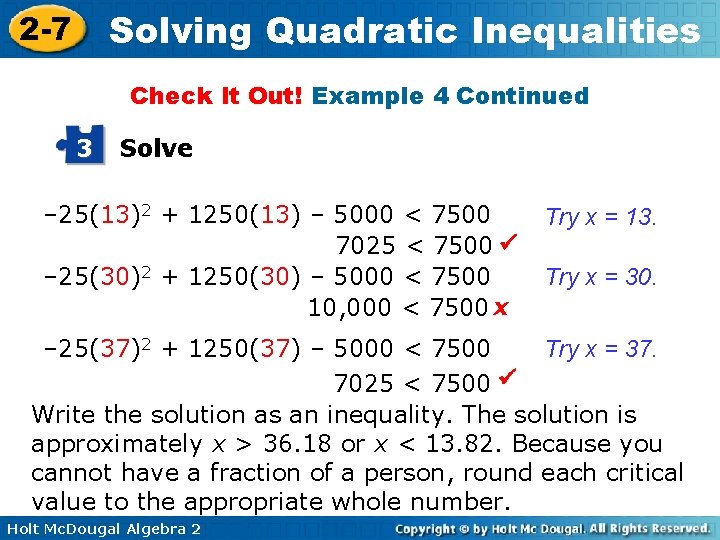
Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 3 Solve – 25(13)2 + 1250(13) – 5000 < 7500 7025 < 7500 – 25(30)2 + 1250(30) – 5000 < 7500 10, 000 < 7500 x Try x = 13. Try x = 30. Try x = 37. – 25(37)2 + 1250(37) – 5000 < 7500 7025 < 7500 Write the solution as an inequality. The solution is approximately x > 36. 18 or x < 13. 82. Because you cannot have a fraction of a person, round each critical value to the appropriate whole number. Holt Mc. Dougal Algebra 2

Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 3 Solve The trip will be rescheduled if the number of people signed up is fewer than 14 people or more than 36 people. Holt Mc. Dougal Algebra 2

Solving Quadratic Inequalities 2 -7 Check It Out! Example 4 Continued 4 Look Back Enter y = – 25 x 2 + 1250 x – 5000 into a graphing calculator, and create a table of values. The table shows that integer values of x less than 13. 81 and greater than 36. 18 result in y-values less than 7500. Holt Mc. Dougal Algebra 2

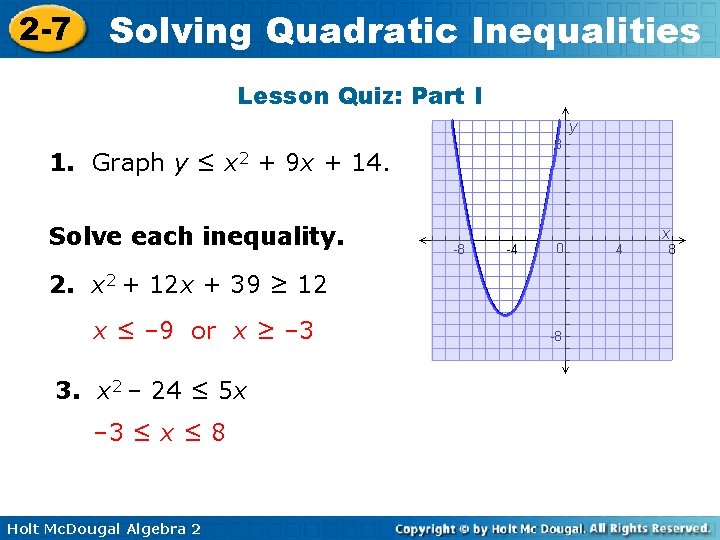
2 -7 Solving Quadratic Inequalities Lesson Quiz: Part I 1. Graph y ≤ x 2 + 9 x + 14. Solve each inequality. 2. x 2 + 12 x + 39 ≥ 12 x ≤ – 9 or x ≥ – 3 3. x 2 – 24 ≤ 5 x – 3 ≤ x ≤ 8 Holt Mc. Dougal Algebra 2

2 -7 Solving Quadratic Inequalities Lesson Quiz: Part II 4. A boat operator wants to offer tours of San Francisco Bay. His profit P for a trip can be modeled by P(x) = – 2 x 2 + 120 x – 788, where x is the cost per ticket. What range of ticket prices will generate a profit of at least $500? between $14 and $46, inclusive Holt Mc. Dougal Algebra 2