2 6 Ratios Rates and Conversions Ratio a

2. 6 Ratios, Rates and Conversions: Ratio: a comparison of two numbers using division. Rate: A ratio that compares quantities measured in different units. Unit Rate: A rate with 1 in the denominato

Conversion Factor: A ratio of two equivalent measures in different units. Unit Analysis (Dimension Analysis): The process of including the units of quantities in a conversion to obtain the desired units.

GOAL:

REAL-WORLD: Justin runs for 25 minutes and does 8 laps around the track. What is the time he takes to do a lap?

Solution: Justin runs 25 minutes Justin does 8 laps The unit rate is: Justin takes an average of 3. 125 minutes per lap

We can use unit rates to compare quantities: Ex: There are three stores that sell the same shirt. Use the info to tell which stores is the most affordable? Store A: $25 for 2 shirts Store B: $45 for 4 shirts Store C: $30 for 3 shirts In order to compare the prices, we must find out the price of a single shirt.

SOLUTION: To find the price per shirt we must find the ratio of price to one shirt. A: $25 for 2 shirts B: $45 for 4 shirts C: $30 for 3 shirts Looking at the price per shirt, Store C is the most affordable.

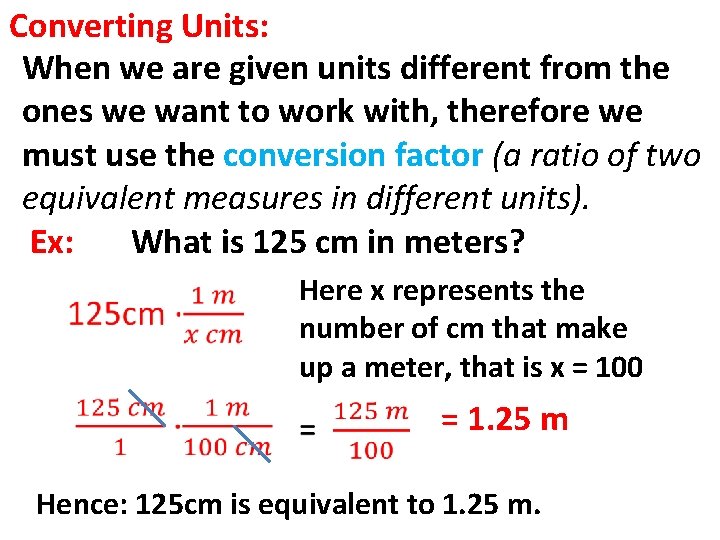
Converting Units: When we are given units different from the ones we want to work with, therefore we must use the conversion factor (a ratio of two equivalent measures in different units). Ex: What is 125 cm in meters? Here x represents the number of cm that make up a meter, that is x = 100 = 1. 25 m Hence: 125 cm is equivalent to 1. 25 m.

NOTICE: By now you must have a knowledge of the conversions we use in everyday life, such as: ALSO: This is the on that will be given to you on the baselines, interims and EOCs.

YOU TRY IT: Convert 5 kg to milligrams?

YOU TRY IT: (Solution) Here we must start by relating kilograms to milligrams: We must know that: 1 kg = 1000 grams 1 gram = 100 milligrams and Using this info we set up the conversion as: Then:

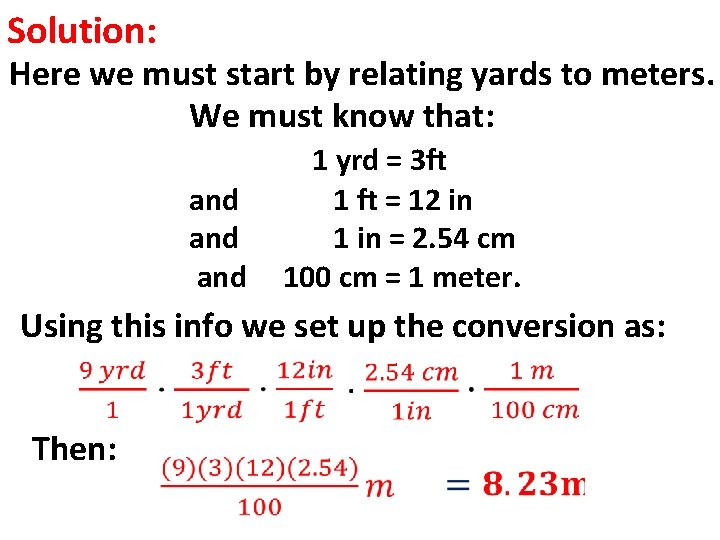
YOU TRY IT: What is 9 yards in meters?

Solution: Here we must start by relating yards to meters. We must know that: 1 yrd = 3 ft 1 ft = 12 in 1 in = 2. 54 cm 100 cm = 1 meter. and and Using this info we set up the conversion as: Then:

YOU TRY IT: Copy and complete the statement. 2. 5 days = ____ sec.

Solution: Relate days to minutes: and 1 day = 24 hrs 1 hr = 24 min 1 min = 60 sec. Using this info we set up the conversion as: Then:

VIDEOS: Unit Conversion https: //www. khanacademy. org/math/arithmetic/r ates-and-ratios/unit_conversion/v/convertingyards-into-inches https: //www. khanacademy. org/math/arithmetic/r ates-and-ratios/unit_conversion/v/convertinggallons-to-quarts-pints-and-cups https: //www. khanacademy. org/math/arithmetic/r ates-and-ratios/unit_conversion/v/convertingpounds-to-ounces

CLASS WORK: Pages: 121 – 123 Problems: As many as it takes to master the concept.