14 Scattering 14 A Cross Section and Differential













- Slides: 36

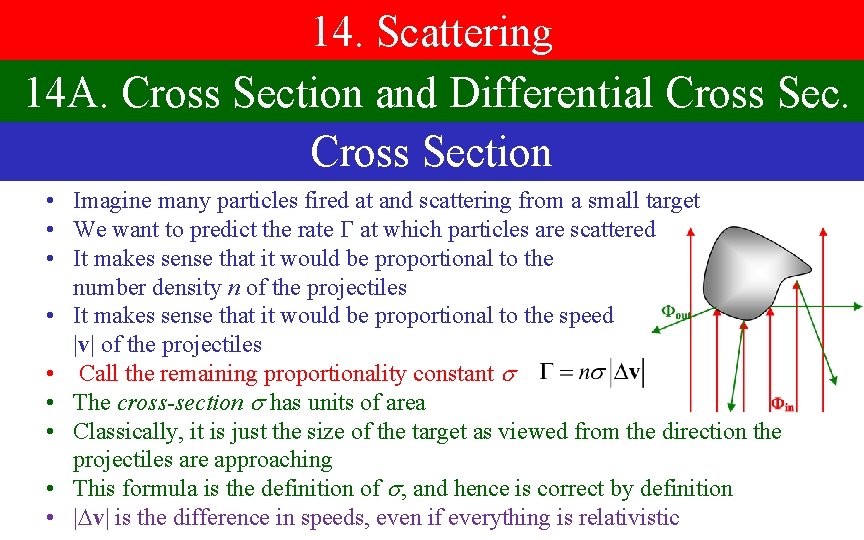
14. Scattering 14 A. Cross Section and Differential Cross Section • Imagine many particles fired at and scattering from a small target • We want to predict the rate at which particles are scattered • It makes sense that it would be proportional to the number density n of the projectiles • It makes sense that it would be proportional to the speed |v| of the projectiles • Call the remaining proportionality constant • The cross-section has units of area • Classically, it is just the size of the target as viewed from the direction the projectiles are approaching • This formula is the definition of , and hence is correct by definition • | v| is the difference in speeds, even if everything is relativistic

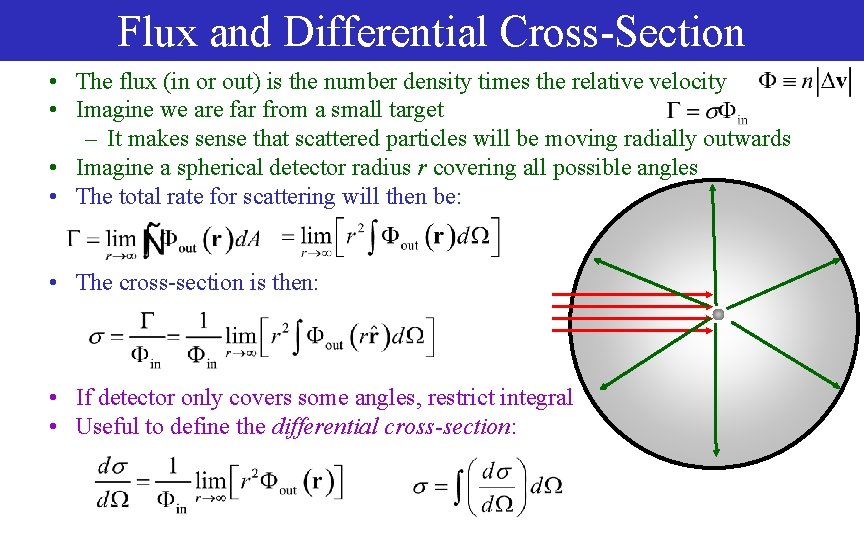
Flux and Differential Cross-Section • The flux (in or out) is the number density times the relative velocity • Imagine we are far from a small target – It makes sense that scattered particles will be moving radially outwards • Imagine a spherical detector radius r covering all possible angles • The total rate for scattering will then be: • The cross-section is then: • If detector only covers some angles, restrict integral • Useful to define the differential cross-section:

Lab Frame and Center of Mass Frame There are two types of collision experiments v' that commonly occur: L v • The lab frame, where the target is stationary and the projectile collides with it v'cm • The center of mass frame, vcm where the incoming particles have net zero momentum • Usually easier to calculate in the cm frame • But then we must relate these two frames • Let the mass of the projectile be m and the target M • Commonly write things in terms of the ratio: • The velocity of the center of mass compared to the lab frame can be found:

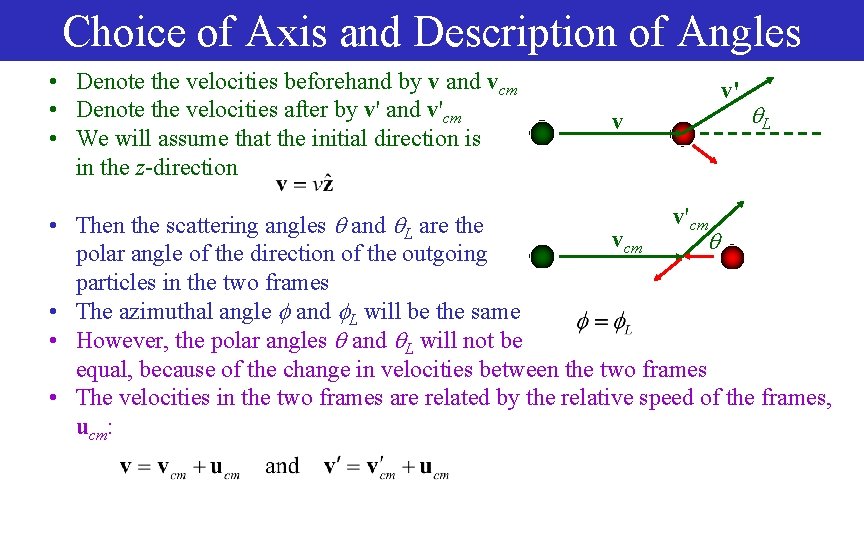
Choice of Axis and Description of Angles • Denote the velocities beforehand by v and vcm • Denote the velocities after by v' and v'cm • We will assume that the initial direction is in the z-direction v' v L v'cm • Then the scattering angles and L are the vcm polar angle of the direction of the outgoing particles in the two frames • The azimuthal angle and L will be the same • However, the polar angles and L will not be equal, because of the change in velocities between the two frames • The velocities in the two frames are related by the relative speed of the frames, ucm:

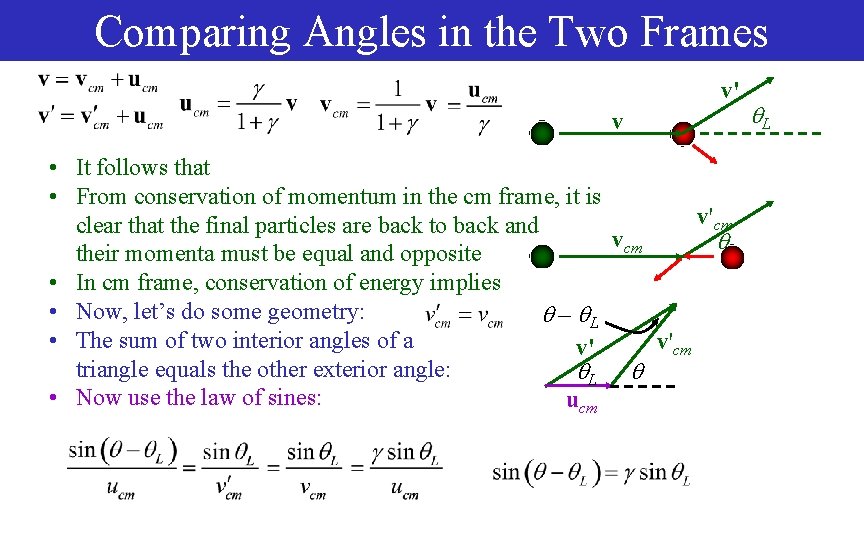
Comparing Angles in the Two Frames v' v • It follows that • From conservation of momentum in the cm frame, it is v'cm clear that the final particles are back to back and vcm their momenta must be equal and opposite • In cm frame, conservation of energy implies • Now, let’s do some geometry: – L • The sum of two interior angles of a v'cm v' triangle equals the other exterior angle: L • Now use the law of sines: ucm L

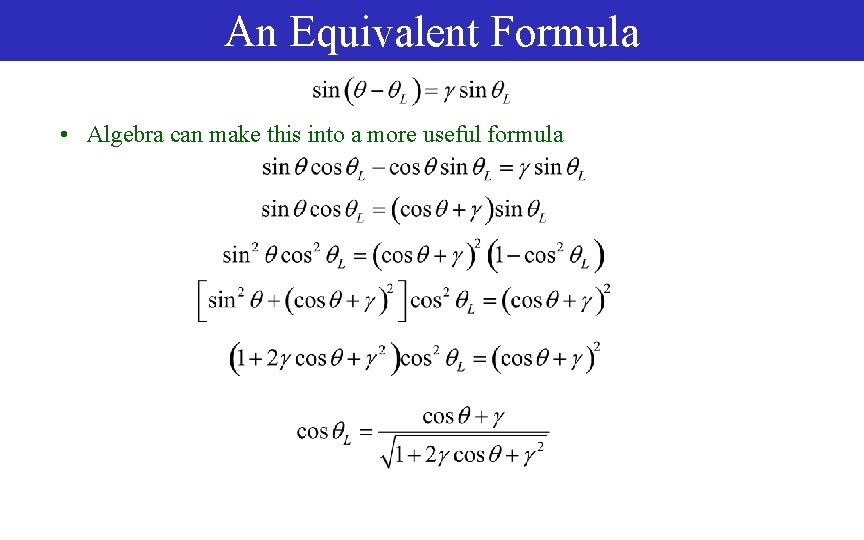
An Equivalent Formula • Algebra can make this into a more useful formula

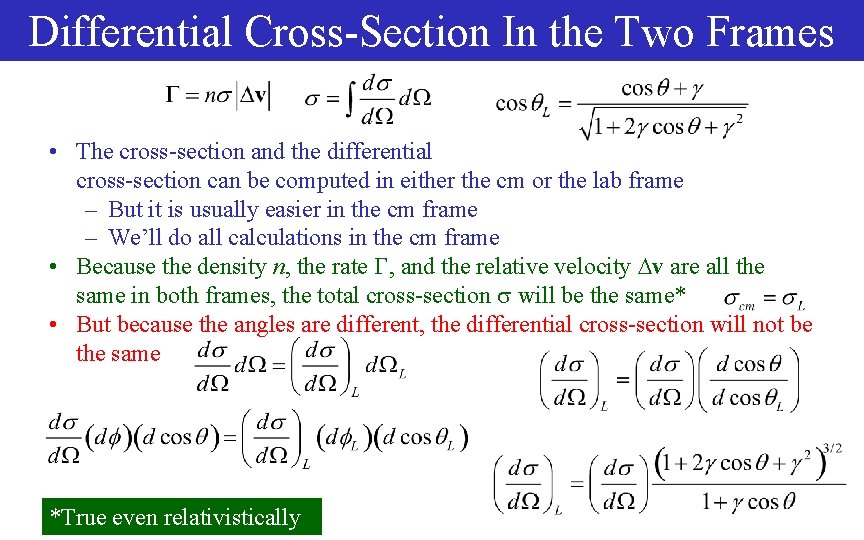
Differential Cross-Section In the Two Frames • The cross-section and the differential cross-section can be computed in either the cm or the lab frame – But it is usually easier in the cm frame – We’ll do all calculations in the cm frame • Because the density n, the rate , and the relative velocity v are all the same in both frames, the total cross-section will be the same* • But because the angles are different, the differential cross-section will not be the same *True even relativistically

Quantum Mechanics and Cross-Section • We need to figure out how to calculate these expression in QM • Typically, we don’t want to use multiple particles at once • Fortunately, even for one particle, we have a pretty good idea of what the density and the flux are: – Number density corresponds to probability density: – Flux corresponds to probability current: • Our formula for differential cross section becomes: • Note that since j appears in numerator and denominator, no need to normalize

Solution in the Asymptotic Region • We now need to start solving Schrödinger’s Equation: • First define the asymptotic wave number k and the scaled potential U by • Schrödinger’s equation is now: • Assume potential vanishes (sufficiently quickly) at infinity – Roughly speaking, it must vanish faster than 1/r • For the incoming wave, we expect a plane wave in the +z direction • For the outgoing wave, makes sense to work in spherical coordinates • Since we are taking r , drop any terms with 1/r 2:

Asymptotic Form and Probability Currents • The gk corresponds to waves coming in from infinity – Physically wrong, so ignore it • The wave at infinity is therefore • We will need probability currents: • Probability current in: • Probability current out:

Cross Section from Asymptotic Form • Now we can do the differential cross-section: • And then we can get the total cross-section • Of course, we don’t yet have a clue what fk is

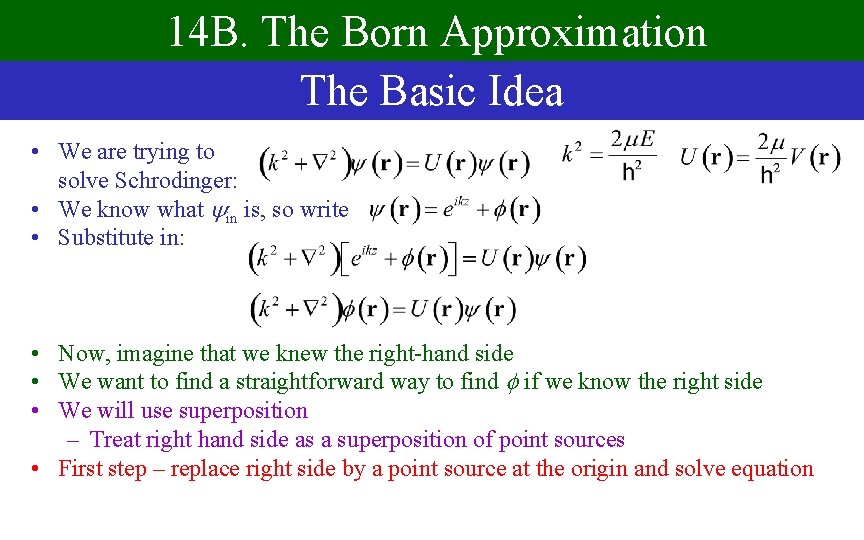
14 B. The Born Approximation The Basic Idea • We are trying to solve Schrodinger: • We know what in is, so write • Substitute in: • Now, imagine that we knew the right-hand side • We want to find a straightforward way to find if we know the right side • We will use superposition – Treat right hand side as a superposition of point sources • First step – replace right side by a point source at the origin and solve equation

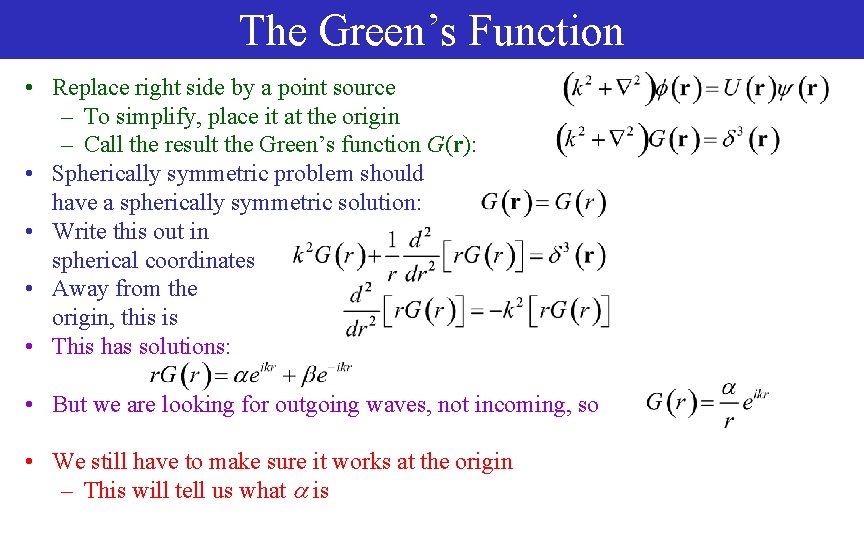
The Green’s Function • Replace right side by a point source – To simplify, place it at the origin – Call the result the Green’s function G(r): • Spherically symmetric problem should have a spherically symmetric solution: • Write this out in spherical coordinates • Away from the origin, this is • This has solutions: • But we are looking for outgoing waves, not incoming, so • We still have to make sure it works at the origin – This will tell us what is

Getting G to Work at the Origin • It is hard to check this equation at the origin, because everything is infinite • To avoid this problem, integrate over a sphere of tiny radius R • Take the limit R 0: • We now know that • We could easily have put the source delta-function anywhere, so we generalize • Laplacian is understood to affect r, not r'

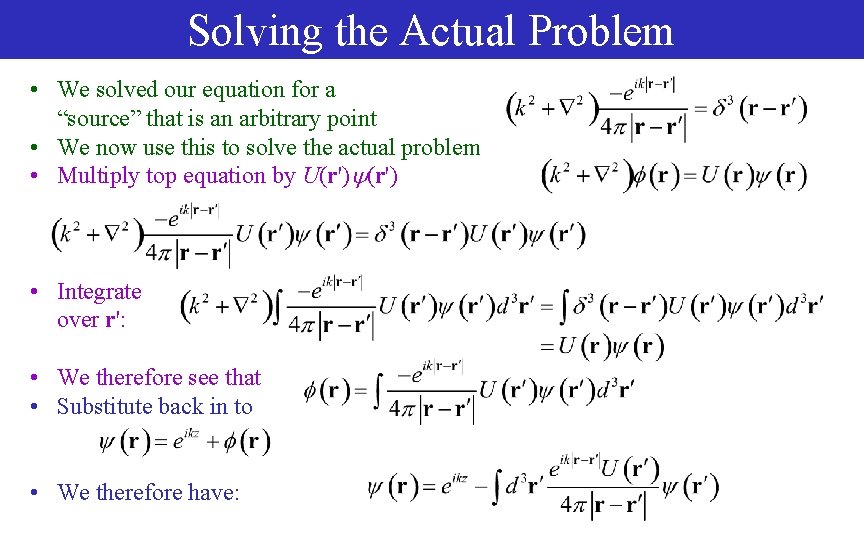
Solving the Actual Problem • We solved our equation for a “source” that is an arbitrary point • We now use this to solve the actual problem • Multiply top equation by U(r') • Integrate over r': • We therefore see that • Substitute back in to • We therefore have:

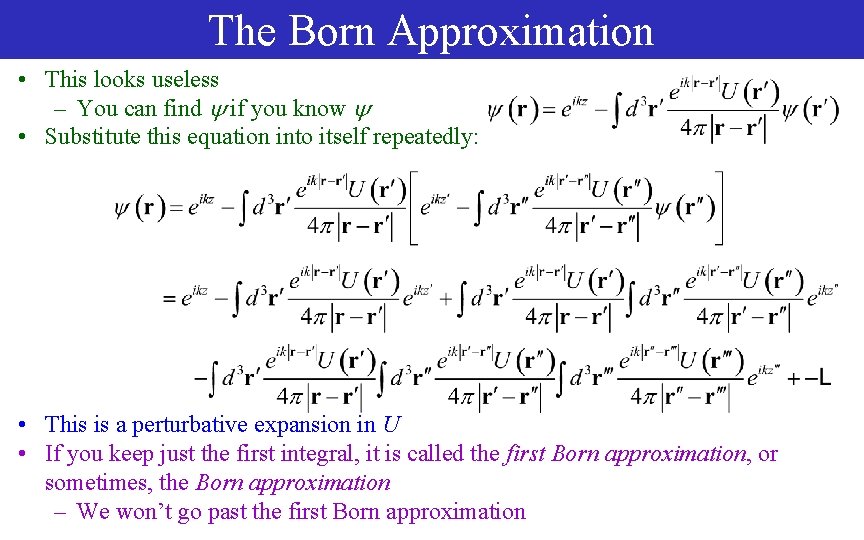
The Born Approximation • This looks useless – You can find if you know • Substitute this equation into itself repeatedly: • This is a perturbative expansion in U • If you keep just the first integral, it is called the first Born approximation, or sometimes, the Born approximation – We won’t go past the first Born approximation

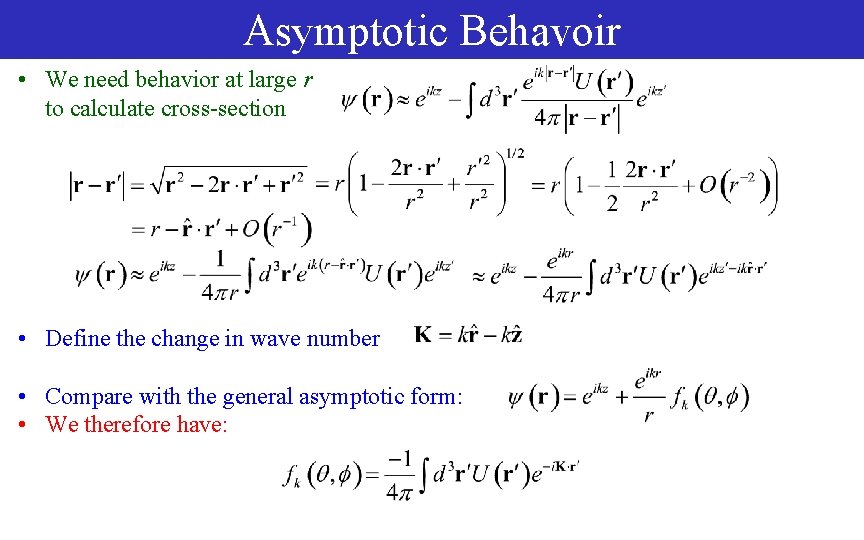
Asymptotic Behavoir • We need behavior at large r to calculate cross-section • Define the change in wave number • Compare with the general asymptotic form: • We therefore have:

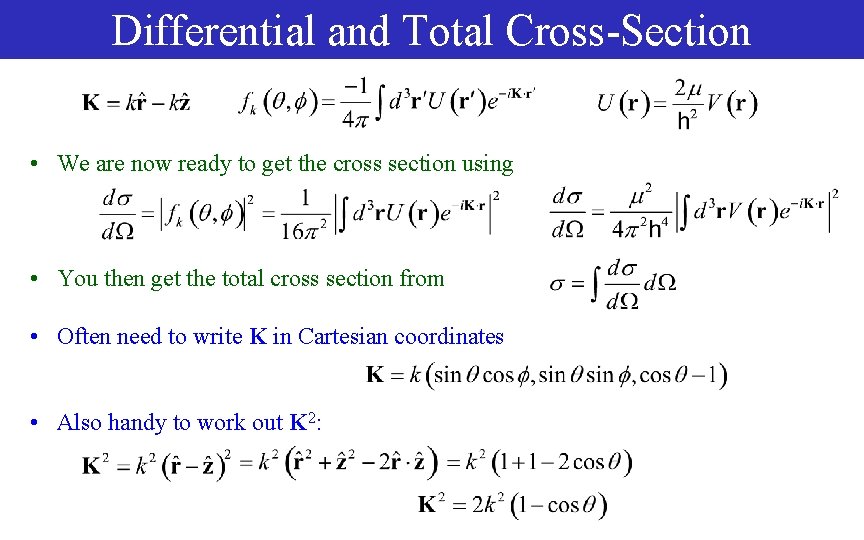
Differential and Total Cross-Section • We are now ready to get the cross section using • You then get the total cross section from • Often need to write K in Cartesian coordinates • Also handy to work out K 2:

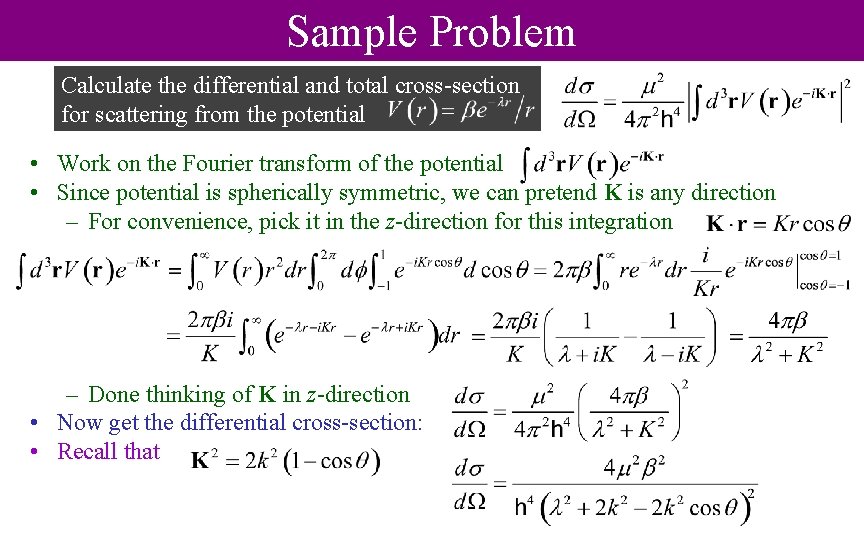
Sample Problem Calculate the differential and total cross-section for scattering from the potential • Work on the Fourier transform of the potential • Since potential is spherically symmetric, we can pretend K is any direction – For convenience, pick it in the z-direction for this integration – Done thinking of K in z-direction • Now get the differential cross-section: • Recall that

Sample Problem (2) Calculate the differential and total cross-section for scattering from the potential • Now get the total cross-section

The Coulomb Potential • Consider the Coulomb potential, given by • We immediately get the differential cross section: • Rewrite in terms of the energy E = 2 k 2/2 Why this is a cheat: • We assumed potential falls off faster than 1/r, for = 0 it does not • Turns out this only causes a shift in the phase of at large r, so answer is right • Total cross-section is infinite • Comes from small angles • Experimentally, there is a minimum angle where we can tell if it scattered

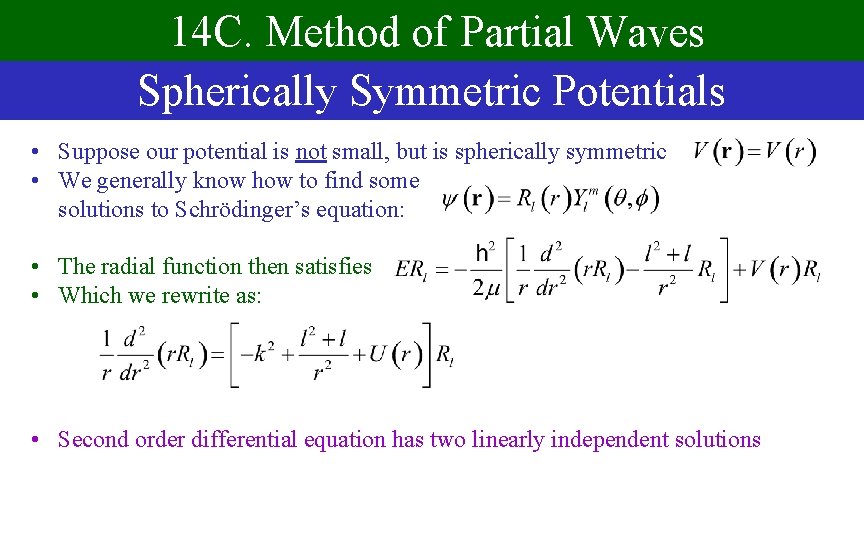
14 C. Method of Partial Waves Spherically Symmetric Potentials • Suppose our potential is not small, but is spherically symmetric • We generally know how to find some solutions to Schrödinger’s equation: • The radial function then satisfies • Which we rewrite as: • Second order differential equation has two linearly independent solutions

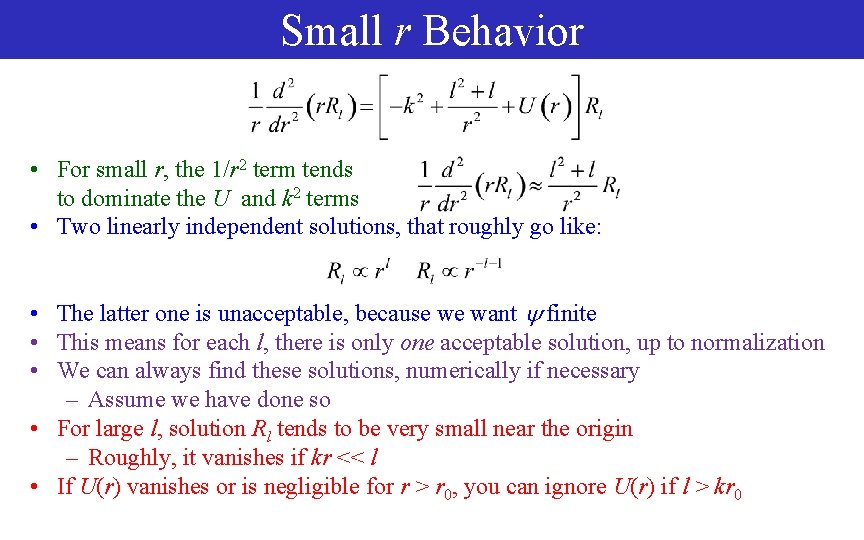
Small r Behavior • For small r, the 1/r 2 term tends to dominate the U and k 2 terms • Two linearly independent solutions, that roughly go like: • The latter one is unacceptable, because we want finite • This means for each l, there is only one acceptable solution, up to normalization • We can always find these solutions, numerically if necessary – Assume we have done so • For large l, solution Rl tends to be very small near the origin – Roughly, it vanishes if kr << l • If U(r) vanishes or is negligible for r > r 0, you can ignore U(r) if l > kr 0

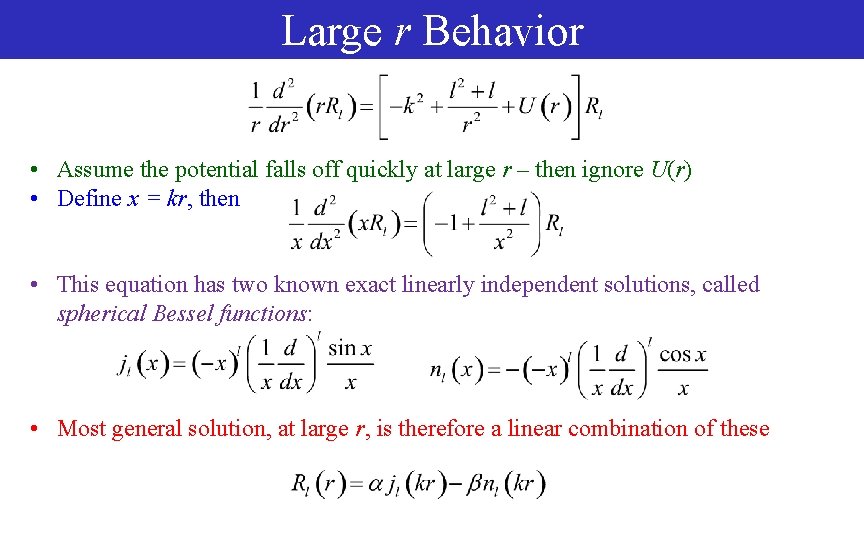
Large r Behavior • Assume the potential falls off quickly at large r – then ignore U(r) • Define x = kr, then • This equation has two known exact linearly independent solutions, called spherical Bessel functions: • Most general solution, at large r, is therefore a linear combination of these

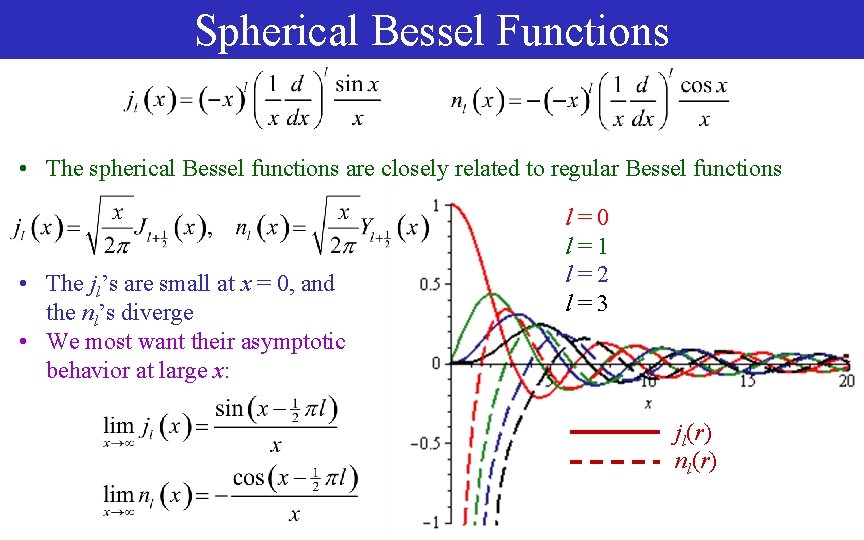
Spherical Bessel Functions • The spherical Bessel functions are closely related to regular Bessel functions • The jl’s are small at x = 0, and the nl’s diverge • We most want their asymptotic behavior at large x: l=0 l=1 l=2 l=3 jl(r) nl(r)

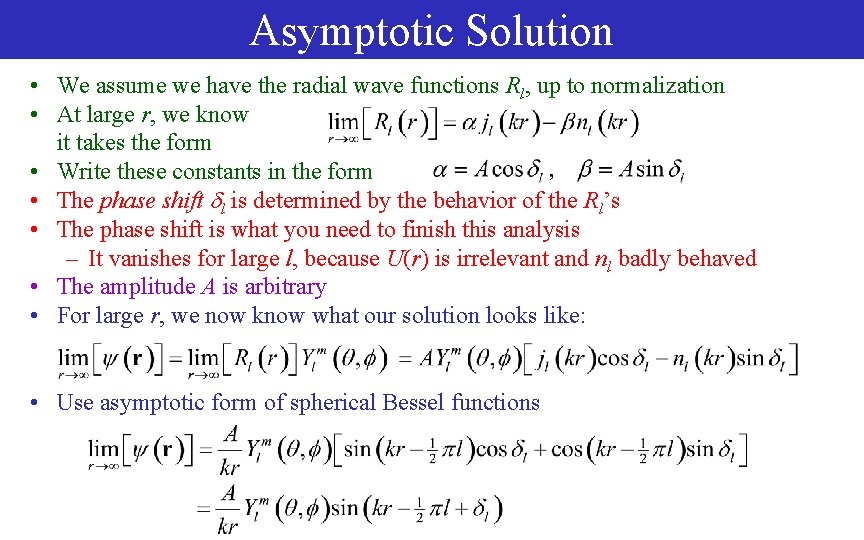
Asymptotic Solution • We assume we have the radial wave functions Rl, up to normalization • At large r, we know it takes the form • Write these constants in the form • The phase shift l is determined by the behavior of the Rl’s • The phase shift is what you need to finish this analysis – It vanishes for large l, because U(r) is irrelevant and nl badly behaved • The amplitude A is arbitrary • For large r, we now know what our solution looks like: • Use asymptotic form of spherical Bessel functions

Our Remaining Goal • Write this in terms of exponentials • This expression has waves coming in and going out in all directions • We want a wave that looks like • Most general solution will be a linear combination • Want to find a combination to make it look like what we want • First step: write eikz in terms of spherical harmonics at large r

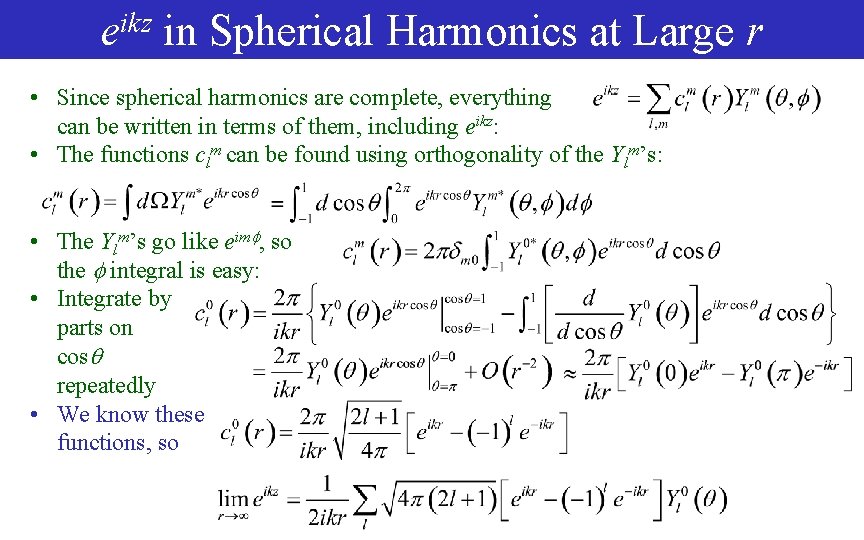
ikz e in Spherical Harmonics at Large r • Since spherical harmonics are complete, everything can be written in terms of them, including eikz: • The functions clm can be found using orthogonality of the Ylm’s: • The Ylm’s go like eim , so the integral is easy: • Integrate by parts on cos repeatedly • We know these functions, so

Announcements ASSIGNMENTS Day Read Homework Today none 14. 3 Wednesday none Friday no class Test Thursday 1: 45, Olin 102 Covers through 13 Reviews on Monday and Wed. Homework Solutions Chapter 12 3/2

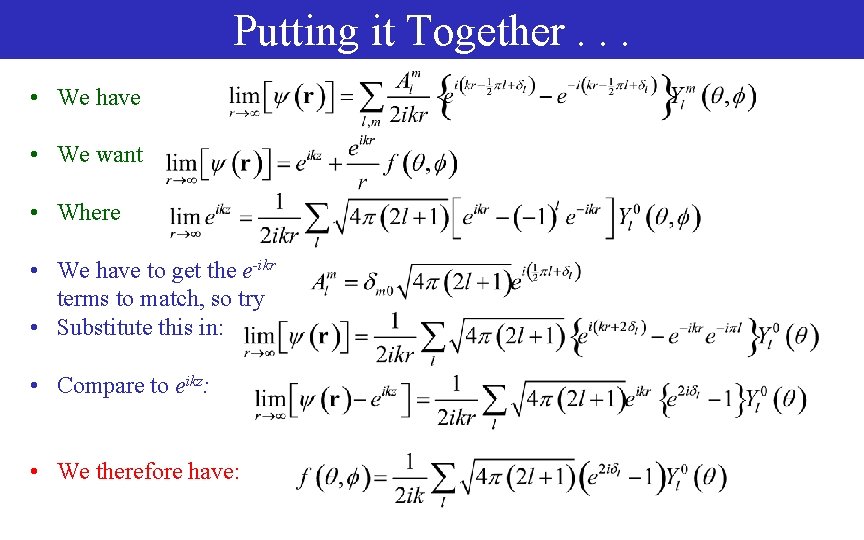
Putting it Together. . . • We have • We want • Where • We have to get the e-ikr terms to match, so try • Substitute this in: • Compare to eikz: • We therefore have:

The Differential and Total Cross-Section • Some algebra: • The differential cross section: • The total cross-section:

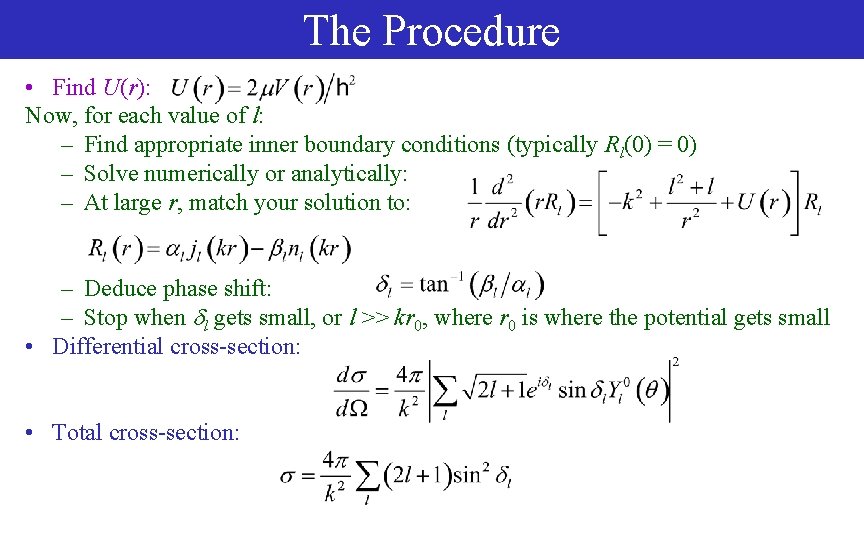
The Procedure • Find U(r): Now, for each value of l: – Find appropriate inner boundary conditions (typically Rl(0) = 0) – Solve numerically or analytically: – At large r, match your solution to: – Deduce phase shift: – Stop when l gets small, or l >> kr 0, where r 0 is where the potential gets small • Differential cross-section: • Total cross-section:

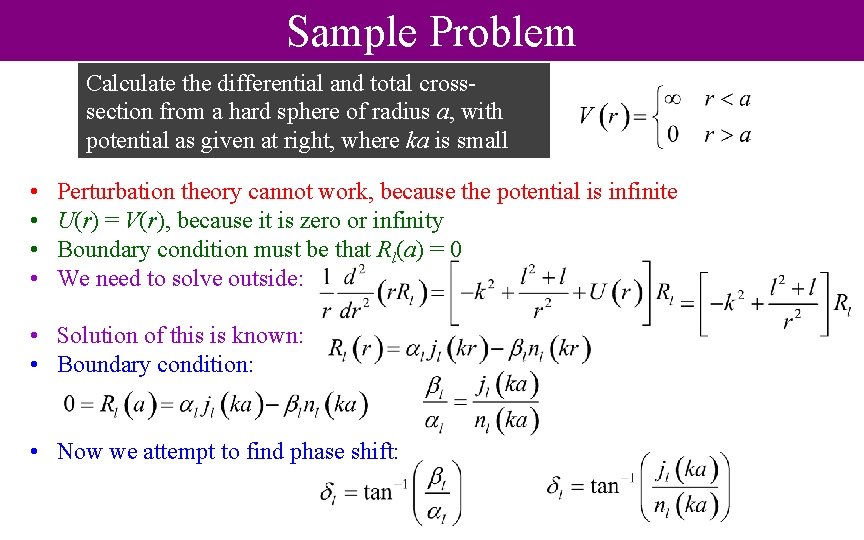
Sample Problem Calculate the differential and total crosssection from a hard sphere of radius a, with potential as given at right, where ka is small • • Perturbation theory cannot work, because the potential is infinite U(r) = V(r), because it is zero or infinity Boundary condition must be that Rl(a) = 0 We need to solve outside: • Solution of this is known: • Boundary condition: • Now we attempt to find phase shift:

Sample Problem (2) Calculate the differential and total crosssection from a hard sphere of radius a, with potential as given at right, where ka is small • • • Now use approximation ka small This means we need to keep up to l ~ ka <<1 Just keep l = 0 Look up spherical Bessel functions: Get the phase shift: Now find differential cross section: • We assumed ka small, so

Comments on Cross Sections • Interestingly, the cross-section is equal to the surface area (NOT the silhouette area) • Generally described as diffraction allows particles to scatter off of all sides • Note that whenever the scale of the potential is small, scattering is dominated by l = 1 (s-wave scattering) • All angles are scattered equally

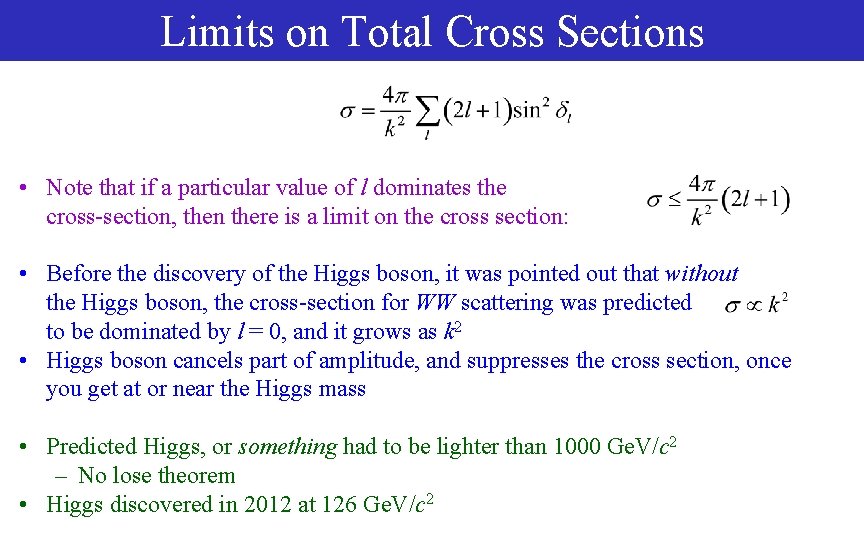
Limits on Total Cross Sections • Note that if a particular value of l dominates the cross-section, then there is a limit on the cross section: • Before the discovery of the Higgs boson, it was pointed out that without the Higgs boson, the cross-section for WW scattering was predicted to be dominated by l = 0, and it grows as k 2 • Higgs boson cancels part of amplitude, and suppresses the cross section, once you get at or near the Higgs mass • Predicted Higgs, or something had to be lighter than 1000 Ge. V/c 2 – No lose theorem • Higgs discovered in 2012 at 126 Ge. V/c 2
 Scattering cross section in nuclear physics
Scattering cross section in nuclear physics Differential cross section
Differential cross section Cross sectional area
Cross sectional area Diffraction and scattering
Diffraction and scattering Test cross and back cross
Test cross and back cross Test cross and back cross with example
Test cross and back cross with example Co dominant meaning
Co dominant meaning How to plot zimm plot in excel
How to plot zimm plot in excel Scattering of light in suspension
Scattering of light in suspension Scattering in a central force field
Scattering in a central force field Riley scattering
Riley scattering Mie scattering vs rayleigh
Mie scattering vs rayleigh Photodesintegration
Photodesintegration Abcd matrix transmission line
Abcd matrix transmission line Liquid crystal display
Liquid crystal display Scattering reaction
Scattering reaction Compton scattering vs photoelectric effect
Compton scattering vs photoelectric effect Polarisation by scattering
Polarisation by scattering Compton effect
Compton effect Scattering matrix for a reciprocal network is
Scattering matrix for a reciprocal network is Coherence and incoherence
Coherence and incoherence Double scattering
Double scattering Monokhromatik
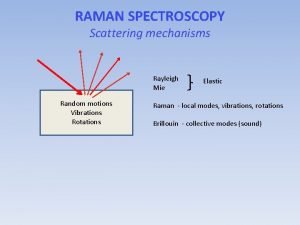
Monokhromatik Raman scattering definition
Raman scattering definition Coherent scattering
Coherent scattering Dynamic light scattering 원리
Dynamic light scattering 원리 Mie plot
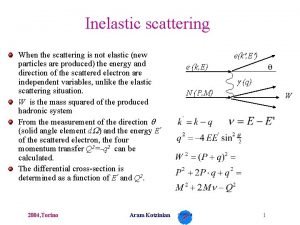
Mie plot Non elastic scattering
Non elastic scattering Rutherford scattering
Rutherford scattering Rayleigh theory of light scattering
Rayleigh theory of light scattering Scattering matrix
Scattering matrix Pauli blocking of light scattering in degenerate fermions
Pauli blocking of light scattering in degenerate fermions Magic t
Magic t Double parton scattering
Double parton scattering Scattering efficiency
Scattering efficiency Scattering matrix
Scattering matrix Scattering of light
Scattering of light