10 3 Inferences What about making inferences r

10 -3 Inferences

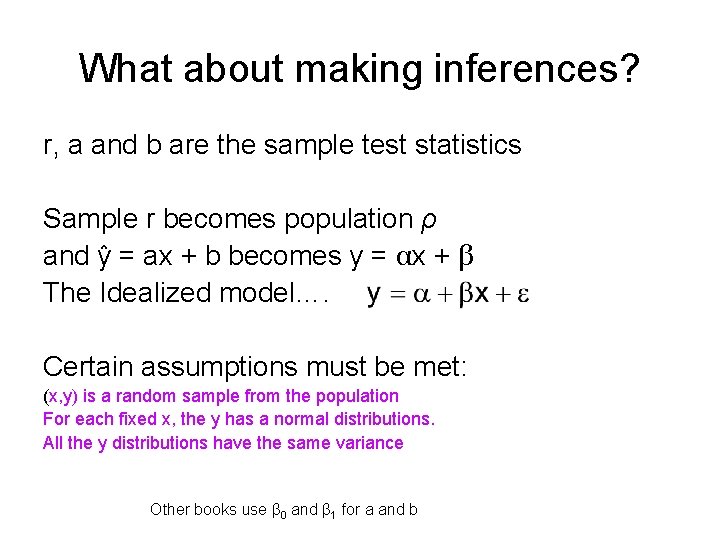
What about making inferences? r, a and b are the sample test statistics Sample r becomes population ρ and ŷ = ax + b becomes y = αx + β The Idealized model…. Certain assumptions must be met: (x, y) is a random sample from the population For each fixed x, the y has a normal distributions. All the y distributions have the same variance Other books use β 0 and β 1 for a and b

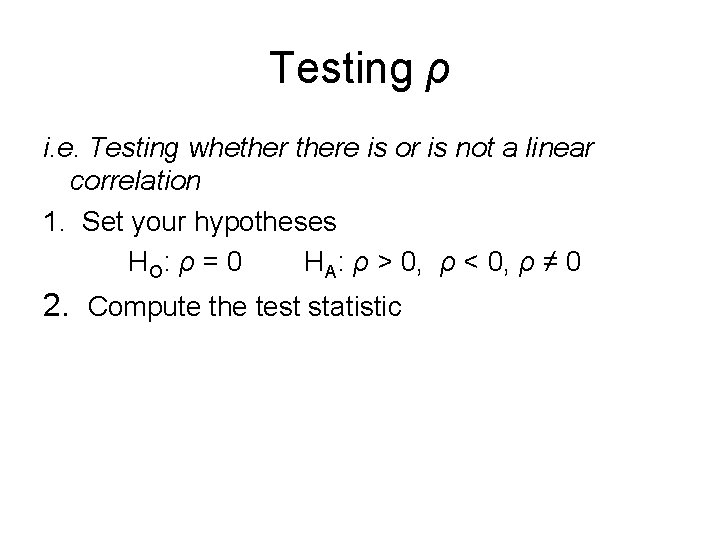
Testing ρ i. e. Testing whethere is or is not a linear correlation 1. Set your hypotheses H O: ρ = 0 HA: ρ > 0, ρ < 0, ρ ≠ 0 2. Compute the test statistic

Testing ρ i. e. Testing whethere is or is not a linear correlation 1. Set your hypotheses H O: ρ = 0 HA: ρ > 0, ρ < 0, ρ ≠ 0 2. Compute the test statistic d. f. = n – 2 Logic dictates how many data sets (n)?

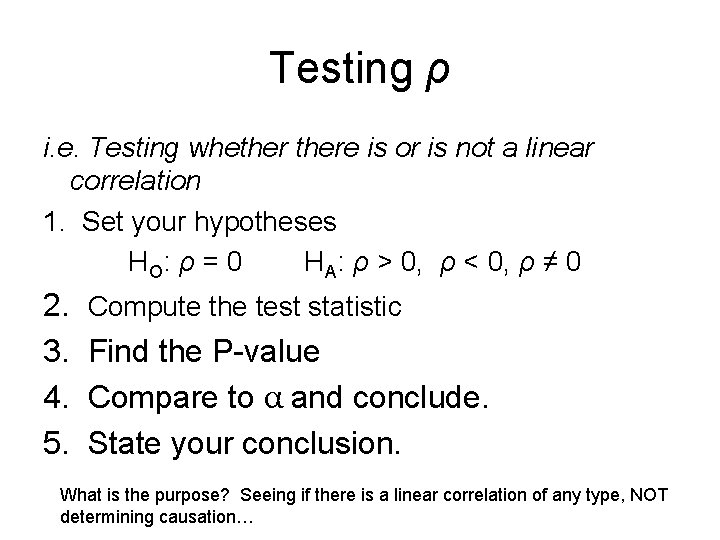
Testing ρ i. e. Testing whethere is or is not a linear correlation 1. Set your hypotheses H O: ρ = 0 HA: ρ > 0, ρ < 0, ρ ≠ 0 2. 3. 4. 5. Compute the test statistic Find the P-value Compare to α and conclude. State your conclusion. What is the purpose? Seeing if there is a linear correlation of any type, NOT determining causation…

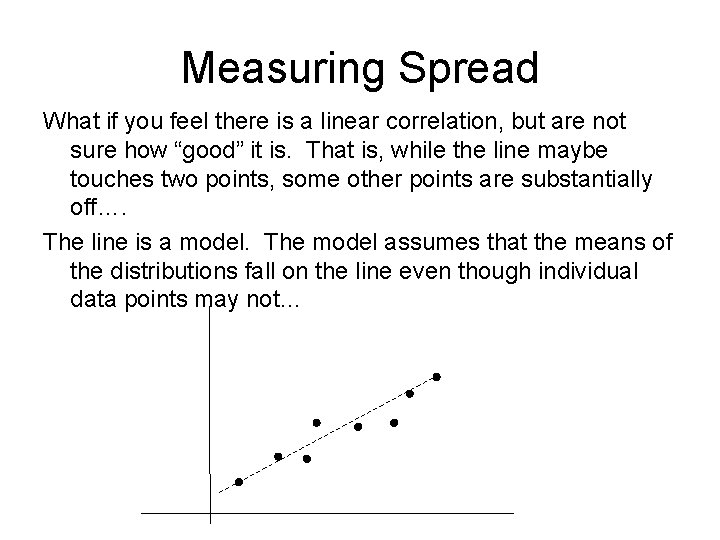
Measuring Spread What if you feel there is a linear correlation, but are not sure how “good” it is. That is, while the line maybe touches two points, some other points are substantially off…. The line is a model. The model assumes that the means of the distributions fall on the line even though individual data points may not…

Measuring Spread Error can be determined a number of ways Method 1: Using residual

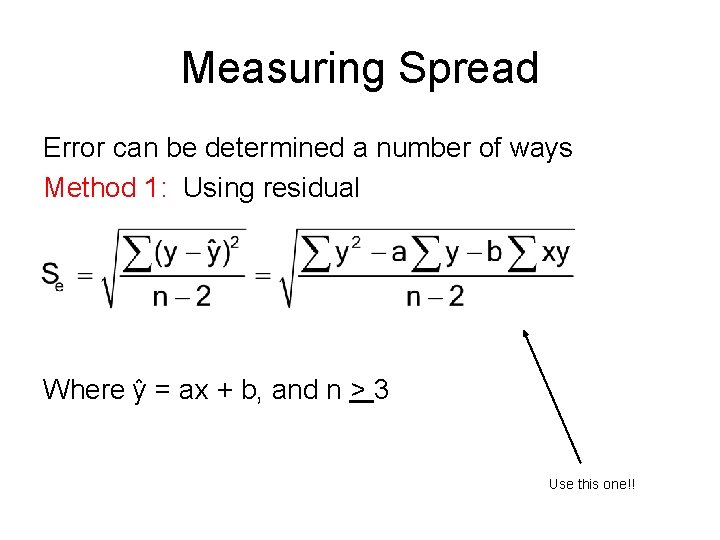
Measuring Spread Error can be determined a number of ways Method 1: Using residual Where ŷ = ax + b, and n > 3 Use this one!!

Measuring Spread (cont) Method 2: Find a confidence interval for y True y, for a population, has a population slope, a population y intercept, plus some sort of random error. Therefore, we can create a confidence interval for y that allows us to predict true y.

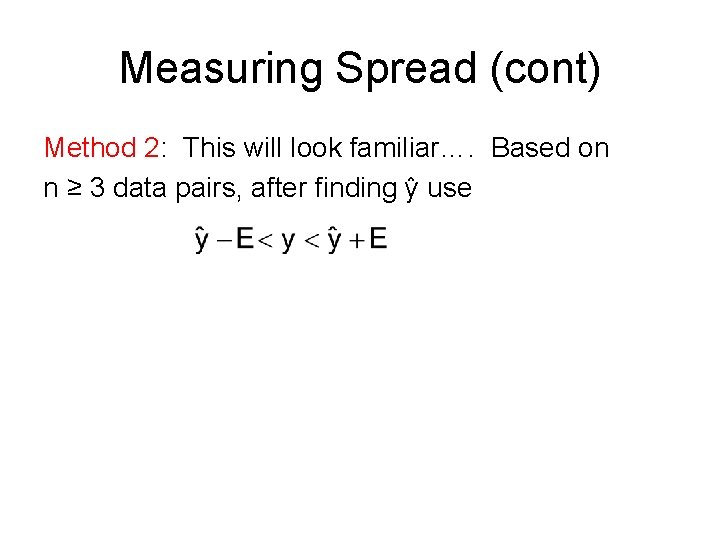
Measuring Spread (cont) Method 2: This will look familiar…. Based on n ≥ 3 data pairs, after finding ŷ use

Measuring Spread (cont) Method 2: This will look familiar…. Based on n ≥ 3 data pairs, after finding ŷ use Where ŷ = ax + b, c = confidence level, n = number of data pairs, and Se is the standard error of estimate

Measuring Spread (cont) Method 3: Using a student t distribution, you will test the population slope (β) Based on a null hypotheses of β = 0 and an alternate like before (greater, less than or not equal to. . ) What will the test statistic simplify to be?

Measuring Spread (cont) Method 3: Using a student t distribution, you will test the population slope (β) d. f. = n-2 Based on a null hypotheses of β = 0 and an alternate like before (greater, less than or not equal to. . ) What will the test statistic simplify to be?

Measuring Spread (cont) Method 3: Therefore, the confidence interval will be

Calculator Your calculator can find standard error, and can also do a linear regression test (callced a Lin. Reg. TTest)

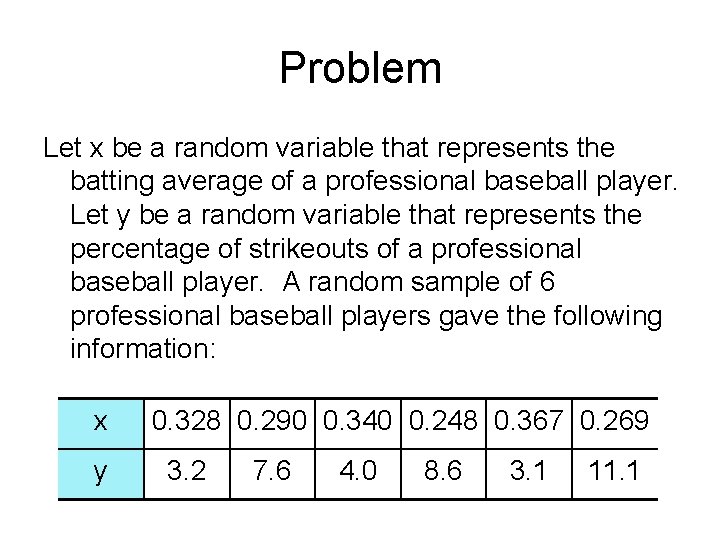
Problem Let x be a random variable that represents the batting average of a professional baseball player. Let y be a random variable that represents the percentage of strikeouts of a professional baseball player. A random sample of 6 professional baseball players gave the following information: x y 0. 328 0. 290 0. 340 0. 248 0. 367 0. 269 3. 2 7. 6 4. 0 8. 6 3. 1 11. 1

Given the following a) use a 5% confidence level to test the claim that ρ≠ 0. b) Find Se, a and b c) Find the predicted percentage of strikeouts for a player with an x = 0. 300 batting average d) Find an 80% confidence interval for y when x = 0. 300 e) Use a 5% level of significance ot test the claim that β≠ 0 f) Find a 90% confidence interval for β and interpret its meaning.