1 Linear and Nonlinear Oscillators Jeffrey Eldred Classical




- Slides: 12

1 Linear and Nonlinear Oscillators Jeffrey Eldred Classical Mechanics and Electromagnetism June 2018 USPAS at MSU

2 Primes and Dots x-prime means derivative of x with respect to s, where s is some spatial coordinate. x-dot mean derivative of x with respect to t, where t is some temporal coordinate. 2 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

3 Linearization of a second order differential equation 3 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

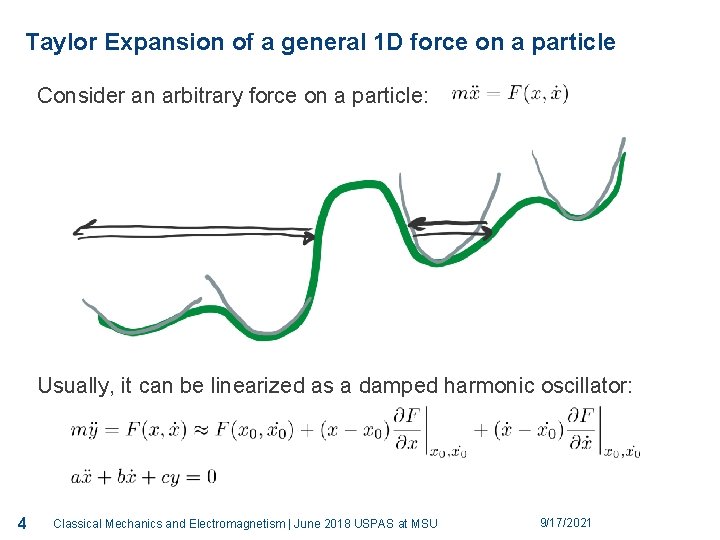
4 Taylor Expansion of a general 1 D force on a particle Consider an arbitrary force on a particle: Usually, it can be linearized as a damped harmonic oscillator: 4 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

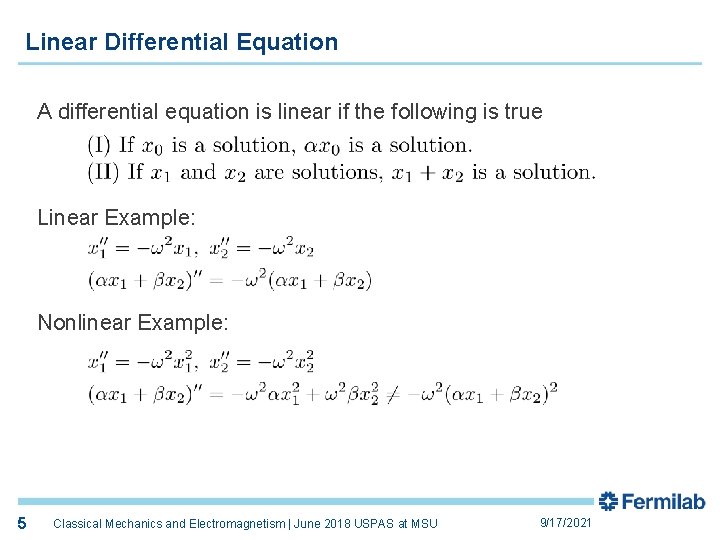
5 Linear Differential Equation A differential equation is linear if the following is true Linear Example: Nonlinear Example: 5 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

6 Method of Undetermined Coefficients (Solving the Harmonic Oscillator) 6 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

7 Linear Differential Equation A differential equation is linear if the following is true Linear Example: Nonlinear Example: 7 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

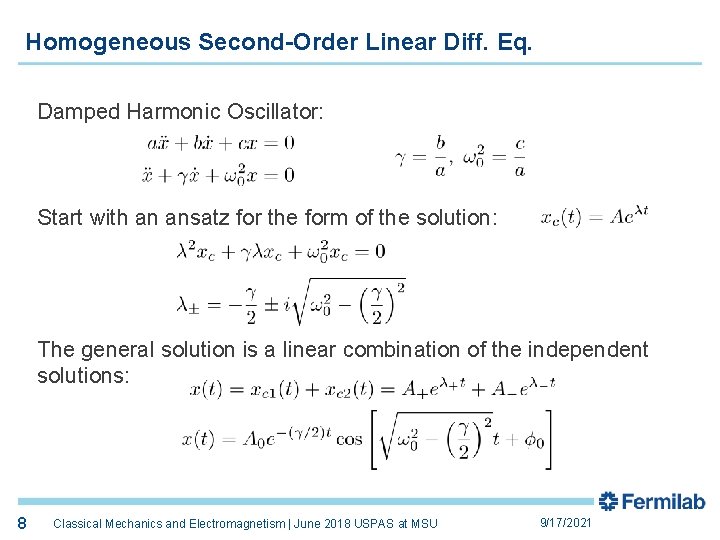
8 Homogeneous Second-Order Linear Diff. Eq. Damped Harmonic Oscillator: Start with an ansatz for the form of the solution: The general solution is a linear combination of the independent solutions: 8 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

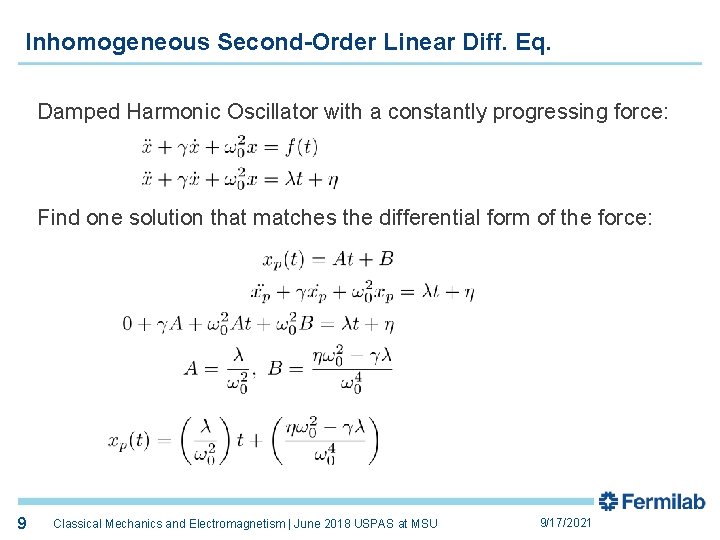
9 Inhomogeneous Second-Order Linear Diff. Eq. Damped Harmonic Oscillator with a constantly progressing force: Find one solution that matches the differential form of the force: 9 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

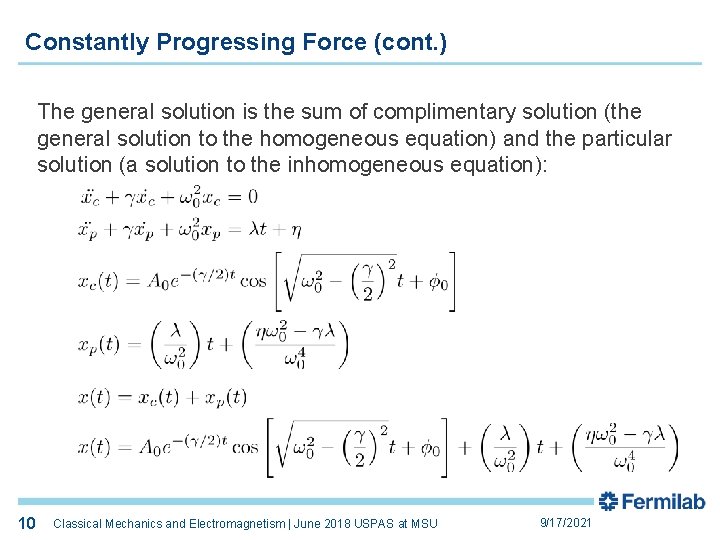
10 Constantly Progressing Force (cont. ) The general solution is the sum of complimentary solution (the general solution to the homogeneous equation) and the particular solution (a solution to the inhomogeneous equation): 10 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

11 Constantly Progressing Force (cont. ) This is just the oscillator, but with it’s pivot point moving at a constant velocity: 11 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021

12 More Oscillator Examples See Lecture 2. 12 Classical Mechanics and Electromagnetism | June 2018 USPAS at MSU 9/17/2021